Question and Answers Forum

1) find the value of ∫_0 ^1 ln(1−x^3 )dx then find the value of ∫_0 ^1 ln(1+x+x^2 )dx 2)find the value of ∫_0 ^1 ln(1+x^3 )dx then calculate ∫_0 ^1 ln(1−x +x^2 )dx

$$\left.\mathrm{1}\right)\:{find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{1}−{x}^{\mathrm{3}} \right){dx}\:{then} \\ $$$${find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{1}+{x}+{x}^{\mathrm{2}} \right){dx} \\ $$$$\left.\mathrm{2}\right){find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\mathrm{1}+{x}^{\mathrm{3}} \right){dx}\:{then}\: \\ $$$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{ln}\left(\mathrm{1}−{x}\:+{x}^{\mathrm{2}} \right){dx} \\ $$

Question Number 36690 Answers: 0 Comments: 1

let f(t) =∫_0 ^1 ln(1 −tx^3 )dx with 0<t≤1 find a simple form of f(t) 2)calculate ∫_0 ^1 ln(2−x^3 )dx .

$${let}\:\:{f}\left({t}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:{ln}\left(\mathrm{1}\:−{tx}^{\mathrm{3}} \right){dx}\:\:{with}\:\mathrm{0}<{t}\leqslant\mathrm{1} \\ $$$${find}\:{a}\:{simple}\:{form}\:{of}\:{f}\left({t}\right)\: \\ $$$$\left.\mathrm{2}\right){calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{ln}\left(\mathrm{2}−{x}^{\mathrm{3}} \right){dx}\:. \\ $$

Question Number 36677 Answers: 4 Comments: 3

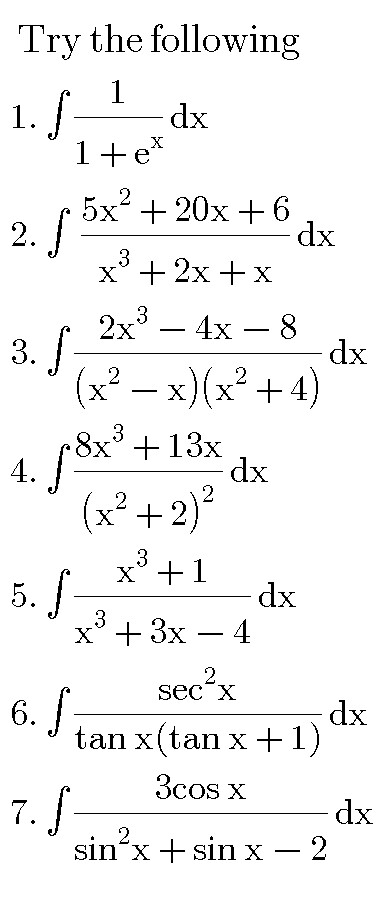
Question Number 36649 Answers: 1 Comments: 4

∫ (1/(x^4 +1)) dx

$$\int\:\frac{\mathrm{1}}{{x}^{\mathrm{4}} +\mathrm{1}}\:{dx} \\ $$

Question Number 36643 Answers: 2 Comments: 1

∫ (x/(x^4 +x^2 +1)) dx = ?

$$\int\:\frac{{x}}{{x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{1}}\:{dx}\:=\:? \\ $$

Question Number 36633 Answers: 0 Comments: 1

calculate ∫_0 ^1 arctan(x^2 +x+1)dx

$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{arctan}\left({x}^{\mathrm{2}} \:+{x}+\mathrm{1}\right){dx} \\ $$

Question Number 36613 Answers: 1 Comments: 5

Question Number 36597 Answers: 2 Comments: 2

∫(dx/(x^(2/3) (1+x^(2/3) ))) = ?

$$\int\frac{\mathrm{d}{x}}{{x}^{\frac{\mathrm{2}}{\mathrm{3}}} \left(\mathrm{1}+{x}^{\frac{\mathrm{2}}{\mathrm{3}}} \right)}\:=\:? \\ $$

Question Number 36659 Answers: 1 Comments: 3

∫ (1/(sin^4 x+cos^4 x)) dx

$$\int\:\frac{\mathrm{1}}{\mathrm{sin}\:^{\mathrm{4}} {x}+\mathrm{cos}\:^{\mathrm{4}} {x}}\:{dx} \\ $$

Question Number 36595 Answers: 2 Comments: 0

∫ ((x dx)/(√(1+x^2 +(√((1+x^2 )^3 ))))) = ?

$$\int\:\frac{{x}\:{dx}}{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} +\sqrt{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{3}} }}}\:=\:? \\ $$

Question Number 36547 Answers: 3 Comments: 0

∫((5x^4 +4x^5 )/((x^5 +x+1)^2 ))

$$\int\frac{\mathrm{5}{x}^{\mathrm{4}} +\mathrm{4}{x}^{\mathrm{5}} }{\left({x}^{\mathrm{5}} +{x}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$

Question Number 36546 Answers: 0 Comments: 0

∫(((x−1))/((x+1)(√(x^3 +x^2 +x))))dx

$$\int\frac{\left({x}−\mathrm{1}\right)}{\left({x}+\mathrm{1}\right)\sqrt{{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}}}{dx} \\ $$

Question Number 36545 Answers: 2 Comments: 6

∫((2x+1)/((x^2 +4x+1)^(3/2) ))dx

$$\int\frac{\mathrm{2}{x}+\mathrm{1}}{\left({x}^{\mathrm{2}} +\mathrm{4}{x}+\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} }{dx} \\ $$

Question Number 36544 Answers: 1 Comments: 0

∫(dx/(tanx+cotx+secx+cosecx))

$$\int\frac{{dx}}{{tanx}+{cotx}+{secx}+{cosecx}} \\ $$

Question Number 36543 Answers: 0 Comments: 0

∫cot^(−1) (x^2 +x+1) dx

$$\int{cot}^{−\mathrm{1}} \left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)\:{dx} \\ $$

Question Number 36541 Answers: 0 Comments: 1

∫(x+(√(1+x^2 )) )^n dx

$$\int\left({x}+\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }\:\right)^{{n}} {dx} \\ $$

Question Number 36538 Answers: 0 Comments: 1

∫(dx/((a+bx^2 )(√(b−ax^2 ))))

$$\int\frac{{dx}}{\left({a}+{bx}^{\mathrm{2}} \right)\sqrt{{b}−{ax}^{\mathrm{2}} \:}}\:\: \\ $$

Question Number 36537 Answers: 0 Comments: 3

Pg 287 Pg 288 Pg 289 Pg 290 Pg 291 Pg 292 Pg 293 Pg 294 Pg 295 Pg 296

Contact: info@tinkutara.com