Question and Answers Forum

All Questions Topic List

IntegrationQuestion and Answers: Page 290

Question Number 37279 Answers: 1 Comments: 1

cslculate ∫∫_([0,1]^2 ) (x−y)e^(−x−y) dxdy .

$${cslculate}\:\int\int_{\left[\mathrm{0},\mathrm{1}\right]^{\mathrm{2}} } \:\:\:\left({x}−{y}\right){e}^{−{x}−{y}} {dxdy}\:. \\ $$

Question Number 37278 Answers: 0 Comments: 1

calculate ∫∫_D x cos(x^2 +y^2 )dxdy with D={(x,y)∈R^2 / 0≤x≤1 and 1≤y≤3}

$$\:{calculate}\:\int\int_{{D}} \:{x}\:{cos}\left({x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} \right){dxdy} \\ $$$${with}\:{D}=\left\{\left({x},{y}\right)\in{R}^{\mathrm{2}} /\:\mathrm{0}\leqslant{x}\leqslant\mathrm{1}\:{and}\right. \\ $$$$\left.\mathrm{1}\leqslant{y}\leqslant\mathrm{3}\right\} \\ $$

Question Number 37276 Answers: 0 Comments: 0

calculate I_n =∫_0 ^4 (−1)^([x]) (x^n −x)dx

$${calculate}\:\:{I}_{{n}} =\int_{\mathrm{0}} ^{\mathrm{4}} \:\left(−\mathrm{1}\right)^{\left[{x}\right]} \left({x}^{{n}} \:−{x}\right){dx} \\ $$

Question Number 37275 Answers: 0 Comments: 0

let A_n = ∫_0 ^(1/n) arctan(1+x^2 )dx 1) calculate A_n 2)find lim_(n→+∞) A_n .

$${let}\:{A}_{{n}} \:=\:\int_{\mathrm{0}} ^{\frac{\mathrm{1}}{{n}}} \:{arctan}\left(\mathrm{1}+{x}^{\mathrm{2}} \right){dx} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{A}_{{n}} \\ $$$$\left.\mathrm{2}\right){find}\:{lim}_{{n}\rightarrow+\infty} \:{A}_{{n}} \:. \\ $$

Question Number 37272 Answers: 0 Comments: 0

let f(x)=cos(x−e^(−x) ) developp f at integr serie.

$${let}\:{f}\left({x}\right)={cos}\left({x}−{e}^{−{x}} \right) \\ $$$${developp}\:{f}\:{at}\:{integr}\:{serie}. \\ $$

Question Number 37271 Answers: 0 Comments: 2

find A_n =∫_1 ^2 ( 1 +(1/x) +(1/x^2 ) +...+(1/x^n ))^2 dx

$${find}\:\:{A}_{{n}} =\int_{\mathrm{1}} ^{\mathrm{2}} \left(\:\mathrm{1}\:+\frac{\mathrm{1}}{{x}}\:+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }\:+...+\frac{\mathrm{1}}{{x}^{{n}} }\right)^{\mathrm{2}} {dx} \\ $$

Question Number 37270 Answers: 1 Comments: 0

find ∫_0 ^1 (((1−x^(n+1) )/(1−x)))^2 dx .

$${find}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \left(\frac{\mathrm{1}−{x}^{{n}+\mathrm{1}} }{\mathrm{1}−{x}}\right)^{\mathrm{2}} {dx}\:. \\ $$

Question Number 37258 Answers: 1 Comments: 0

∫ ((x^3 +1)/(√(x^2 +x))) dx = ?

$$\int\:\frac{{x}^{\mathrm{3}} +\mathrm{1}}{\sqrt{{x}^{\mathrm{2}} +{x}}}\:{dx}\:=\:? \\ $$

Question Number 37540 Answers: 1 Comments: 0

For x>1 , ∫ sin^(−1) (((2x)/(1+x^2 )))dx = ?

$$\mathrm{For}\:{x}>\mathrm{1}\:,\: \\ $$$$\int\:\mathrm{sin}^{−\mathrm{1}} \left(\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\right){dx}\:=\:? \\ $$

Question Number 37243 Answers: 0 Comments: 0

find f (t) =∫_0 ^∞ e^x ln(1+e^(−tx) )dx with t >0 . 2) let u_n = ∫_0 ^∞ e^x ln(1+e^(−nx) ) dx find lim_(n→+∞) u_n .

$${find}\:{f}\:\left({t}\right)\:=\int_{\mathrm{0}} ^{\infty} \:{e}^{{x}} {ln}\left(\mathrm{1}+{e}^{−{tx}} \right){dx}\:{with}\:{t}\:>\mathrm{0}\:. \\ $$$$\left.\mathrm{2}\right)\:{let}\:{u}_{{n}} =\:\int_{\mathrm{0}} ^{\infty} \:\:{e}^{{x}} {ln}\left(\mathrm{1}+{e}^{−{nx}} \right)\:{dx} \\ $$$${find}\:{lim}_{{n}\rightarrow+\infty} {u}_{{n}} \:. \\ $$

Question Number 37237 Answers: 1 Comments: 1

calculate ∫_0 ^(π/2) ((cosθ.sinθ)/(cosθ +sinθ)) dθ .

$${calculate}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:\frac{{cos}\theta.{sin}\theta}{{cos}\theta\:+{sin}\theta}\:{d}\theta\:. \\ $$

Question Number 37236 Answers: 0 Comments: 0

calculate ∫_0 ^(2π) ∣sin(((kt)/2))∣ dt with k integr and k≥3

$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} \mid{sin}\left(\frac{{kt}}{\mathrm{2}}\right)\mid\:{dt}\:\:{with}\:{k}\:{integr} \\ $$$${and}\:{k}\geqslant\mathrm{3} \\ $$

Question Number 37235 Answers: 1 Comments: 0

calculate ∫_0 ^(π/2) (√(4sin^2 t +cos^2 (t))) dt

$${calculate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \sqrt{\mathrm{4}{sin}^{\mathrm{2}} {t}\:+{cos}^{\mathrm{2}} \left({t}\right)}\:\:{dt} \\ $$

Question Number 37233 Answers: 0 Comments: 0

calculate ∫_(−∞) ^(+∞) (((1+ix)/(1−ix)))^(m−n) (dx/(1+x^2 )) dx with m and n integrs

$${calculate} \\ $$$$\int_{−\infty} ^{+\infty} \:\:\left(\frac{\mathrm{1}+{ix}}{\mathrm{1}−{ix}}\right)^{{m}−{n}} \:\:\:\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{2}} }\:{dx}\:\:{with}\:{m}\:{and}\:{n} \\ $$$${integrs}\: \\ $$

Question Number 37225 Answers: 0 Comments: 0

let n≥2 and f : R_n [x]→R_2 [x] / f(p) =xp(1) +(x^2 −4)p(0) 1) prove that f is linear 2) find dim Kerf and dimIm(f)

$${let}\:{n}\geqslant\mathrm{2}\:{and}\:{f}\:\::\:{R}_{{n}} \left[{x}\right]\rightarrow{R}_{\mathrm{2}} \left[{x}\right]\:/ \\ $$$${f}\left({p}\right)\:={xp}\left(\mathrm{1}\right)\:+\left({x}^{\mathrm{2}} \:−\mathrm{4}\right){p}\left(\mathrm{0}\right) \\ $$$$\left.\mathrm{1}\right)\:{prove}\:{that}\:{f}\:{is}\:{linear} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{dim}\:{Kerf}\:{and}\:{dimIm}\left({f}\right) \\ $$

Question Number 37224 Answers: 0 Comments: 0

let p(x)=(1+jx)^n −(1−jx)^n with j=e^(i((2π)/3)) find p at form r(x)e^(iθ(x)) 2) calculate ∫_0 ^1 r(x) e^(iθ(x)) dx .

$${let}\:{p}\left({x}\right)=\left(\mathrm{1}+{jx}\right)^{{n}} \:−\left(\mathrm{1}−{jx}\right)^{{n}} \:{with} \\ $$$${j}={e}^{{i}\frac{\mathrm{2}\pi}{\mathrm{3}}} \:\:\:{find}\:{p}\:{at}\:\:{form}\:{r}\left({x}\right){e}^{{i}\theta\left({x}\right)} \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} {r}\left({x}\right)\:{e}^{{i}\theta\left({x}\right)} {dx}\:. \\ $$

Question Number 37179 Answers: 0 Comments: 4

∫ ((3x^2 +1)/((x^2 −1)^3 )) dx = ?

$$\int\:\frac{\mathrm{3}{x}^{\mathrm{2}} +\mathrm{1}}{\left({x}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{3}} }\:{dx}\:=\:? \\ $$

Question Number 37171 Answers: 0 Comments: 0

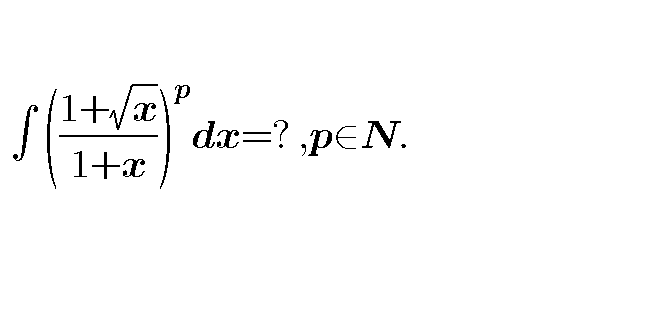
Question Number 37143 Answers: 0 Comments: 0

Why are following statements wrong? a) There exists a function with domain R satisfying f(x)<0 ∀x , f′(x)>0∀x and f′′(x)>0∀x. b) If f′′(c)=0 then (c,f(c)) is an inflection point.

$$\mathrm{Why}\:\mathrm{are}\:\mathrm{following}\:\mathrm{statements}\:\mathrm{wrong}? \\ $$$$\left.\mathrm{a}\right)\:\mathrm{There}\:\mathrm{exists}\:\mathrm{a}\:\mathrm{function}\:\mathrm{with}\:\mathrm{domain}\: \\ $$$$\mathrm{R}\:\mathrm{satisfying}\:\mathrm{f}\left(\mathrm{x}\right)<\mathrm{0}\:\forall\mathrm{x}\:,\:\mathrm{f}'\left(\mathrm{x}\right)>\mathrm{0}\forall\mathrm{x}\:\mathrm{and} \\ $$$$\mathrm{f}''\left(\mathrm{x}\right)>\mathrm{0}\forall\mathrm{x}. \\ $$$$ \\ $$$$\left.\mathrm{b}\right)\:\mathrm{If}\:\mathrm{f}''\left(\mathrm{c}\right)=\mathrm{0}\:\mathrm{then}\:\left(\mathrm{c},\mathrm{f}\left(\mathrm{c}\right)\right)\:\mathrm{is}\:\mathrm{an}\:\mathrm{inflection} \\ $$$$\mathrm{point}. \\ $$

Question Number 37108 Answers: 1 Comments: 0

If 4x+8cos x+tan x−2sec x−4log {cosx(1+sin x)}≥6 ∀ x ε [0,ψ) then largest value of ψ is ?

$$\mathrm{If}\:\mathrm{4}{x}+\mathrm{8cos}\:{x}+\mathrm{tan}\:{x}−\mathrm{2sec}\:{x}−\mathrm{4log}\:\left\{\mathrm{cos}{x}\left(\mathrm{1}+\mathrm{sin}\:{x}\right)\right\}\geqslant\mathrm{6} \\ $$$$\forall\:{x}\:\epsilon\:\left[\mathrm{0},\psi\right)\:\mathrm{then}\:\mathrm{largest}\:\mathrm{value}\:\mathrm{of}\:\psi\:\mathrm{is}\:? \\ $$

Question Number 37079 Answers: 1 Comments: 0

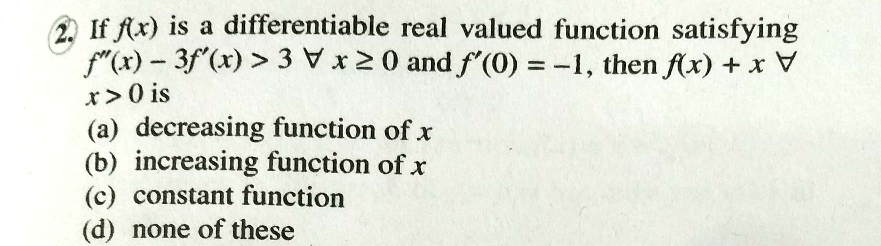
Question Number 37071 Answers: 2 Comments: 1

find the value of ∫_0 ^(π/2) ((xdx)/(1+cosx))

$${find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{xdx}}{\mathrm{1}+{cosx}} \\ $$

Question Number 37067 Answers: 2 Comments: 1

find ∫ (dx/((x+1)(√(1+x^2 ))))

$${find}\:\int\:\:\:\:\:\frac{{dx}}{\left({x}+\mathrm{1}\right)\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }} \\ $$

Question Number 37036 Answers: 0 Comments: 3

∫_0 ^( a) (1−((b−x)/(√((b−x)^2 +cx)))) dx = ?

$$\int_{\mathrm{0}} ^{\:\:{a}} \left(\mathrm{1}−\frac{{b}−{x}}{\sqrt{\left({b}−{x}\right)^{\mathrm{2}} +{cx}}}\right)\:{dx}\:=\:? \\ $$

Question Number 37028 Answers: 1 Comments: 0

∫((2x−1)/(5x^2 −x+2)) dx = ?

$$\int\frac{\mathrm{2}{x}−\mathrm{1}}{\mathrm{5}{x}^{\mathrm{2}} −{x}+\mathrm{2}}\:{dx}\:\:=\:\:? \\ $$

Question Number 37018 Answers: 3 Comments: 3

Pg 285 Pg 286 Pg 287 Pg 288 Pg 289 Pg 290 Pg 291 Pg 292 Pg 293 Pg 294

Terms of Service

Contact: info@tinkutara.com