Question and Answers Forum

All Questions Topic List

IntegrationQuestion and Answers: Page 267

Question Number 45151 Answers: 0 Comments: 2

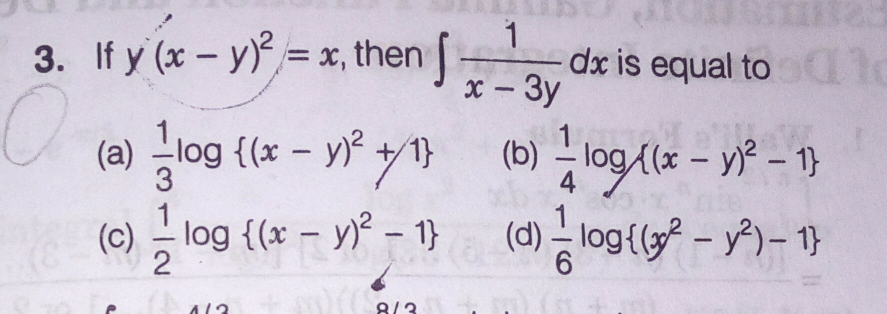
Question Number 45165 Answers: 1 Comments: 0

Question Number 45164 Answers: 0 Comments: 6

Question Number 45158 Answers: 0 Comments: 1

∫((e^(2x) −e^x +1)/((e^x sinx+cosx)(e^x cosx−sinx)))dx =?

$$\:\:\int\frac{{e}^{\mathrm{2}{x}} −{e}^{{x}} +\mathrm{1}}{\left({e}^{{x}} {sinx}+{cosx}\right)\left({e}^{{x}} {cosx}−{sinx}\right)}{dx}\:=? \\ $$

Question Number 45128 Answers: 1 Comments: 1

Question Number 45117 Answers: 1 Comments: 3

Prove that ∫_0 ^1 ((x^a −1)/(log x)) dx = log (a+1).

$${Prove}\:{that}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{x}^{{a}} −\mathrm{1}}{\mathrm{log}\:{x}}\:{dx}\:=\:\mathrm{log}\:\left({a}+\mathrm{1}\right). \\ $$

Question Number 45075 Answers: 1 Comments: 2

∫_0 ^∞ (t^(a−1) /(1+t))dt=(π/(sin(πa))) please prove that

$$\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{t}^{\mathrm{a}−\mathrm{1}} }{\mathrm{1}+\mathrm{t}}\mathrm{dt}=\frac{\pi}{\mathrm{sin}\left(\pi\mathrm{a}\right)}\:\:\: \\ $$$$\mathrm{please}\:\mathrm{prove}\:\mathrm{that} \\ $$

Question Number 45063 Answers: 0 Comments: 2

∫_0 ^(2π) e^(cos θ) cos (sin θ)dθ = ?

$$\int_{\mathrm{0}} ^{\mathrm{2}\pi} {e}^{\mathrm{cos}\:\theta} \mathrm{cos}\:\left(\mathrm{sin}\:\theta\right){d}\theta\:=\:? \\ $$

Question Number 45045 Answers: 1 Comments: 0

find the value of ∫_0 ^∞ (t^3 /(1+e^t ))dt .

$${find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}^{\mathrm{3}} }{\mathrm{1}+{e}^{{t}} }{dt}\:. \\ $$

Question Number 45044 Answers: 0 Comments: 1

let f(x) =x^2 , function 2π peridic even 1) developp f at fourier serie 2)find the value of Σ_(n=1) ^∞ (1/n^4 )

$${let}\:{f}\left({x}\right)\:={x}^{\mathrm{2}} \:,\:{function}\:\mathrm{2}\pi\:{peridic}\:{even} \\ $$$$\left.\mathrm{1}\right)\:{developp}\:{f}\:{at}\:{fourier}\:{serie} \\ $$$$\left.\mathrm{2}\right){find}\:{the}\:{value}\:{of}\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\mathrm{1}}{{n}^{\mathrm{4}} } \\ $$

Question Number 45043 Answers: 3 Comments: 0

let f(x)=((1+(√(1+x^2 )))/x) 1) calculate ∫_1 ^3 f(x)dx 2) determine f^(−1) (x) 3) find ∫ f^(−1) (x)dx .

$${let}\:{f}\left({x}\right)=\frac{\mathrm{1}+\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}{{x}} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:\:\int_{\mathrm{1}} ^{\mathrm{3}} {f}\left({x}\right){dx} \\ $$$$\left.\mathrm{2}\right)\:{determine}\:{f}^{−\mathrm{1}} \left({x}\right) \\ $$$$\left.\mathrm{3}\right)\:{find}\:\int\:{f}^{−\mathrm{1}} \left({x}\right){dx}\:\:. \\ $$

Question Number 45021 Answers: 2 Comments: 0

∫_0 ^(π/2) (dx/(1+tan^(13) x)) = ?

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{dx}}{\mathrm{1}+\mathrm{tan}\:^{\mathrm{13}} {x}}\:=\:? \\ $$

Question Number 45020 Answers: 2 Comments: 5

Question Number 45019 Answers: 1 Comments: 0

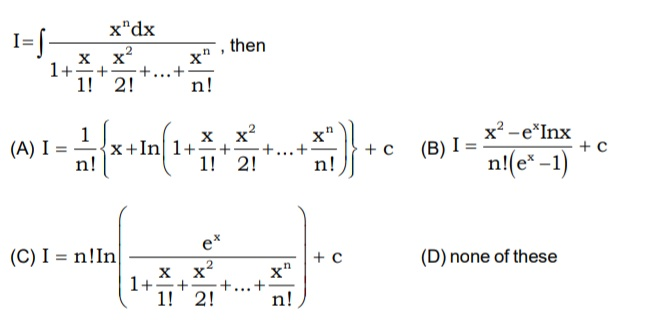
Question Number 44994 Answers: 1 Comments: 0

∫e^x (((1+sin x)/(1−cos x)))dx = ?

$$\int\mathrm{e}^{\mathrm{x}} \left(\frac{\mathrm{1}+\mathrm{sin}\:\mathrm{x}}{\mathrm{1}−\mathrm{cos}\:\mathrm{x}}\right)\mathrm{dx}\:=\:? \\ $$

Question Number 44993 Answers: 1 Comments: 1

∫(x/(sin x))dx=?

$$\int\frac{\mathrm{x}}{\mathrm{sin}\:\mathrm{x}}\mathrm{dx}=? \\ $$

Question Number 44992 Answers: 2 Comments: 0

∫e^x (((1−sin x)/(1+cos x)))dx ?

$$\int\mathrm{e}^{\mathrm{x}} \left(\frac{\mathrm{1}−\mathrm{sin}\:\mathrm{x}}{\mathrm{1}+\mathrm{cos}\:\mathrm{x}}\right)\mathrm{dx}\:? \\ $$

Question Number 44921 Answers: 1 Comments: 1

∫(1/(1+ln x))=?

$$\int\frac{\mathrm{1}}{\mathrm{1}+\mathrm{ln}\:\mathrm{x}}=? \\ $$

Question Number 44781 Answers: 2 Comments: 0

prove that:− ∫(1/(t(√(1−t^2 ))))dt = ln(1−(√(1−t^2 )))+C

$$\boldsymbol{{prove}}\:\boldsymbol{{that}}:−\:\int\frac{\mathrm{1}}{\boldsymbol{\mathrm{t}}\sqrt{\mathrm{1}−\boldsymbol{\mathrm{t}}^{\mathrm{2}} }}\boldsymbol{\mathrm{dt}}\:=\:\boldsymbol{\mathrm{ln}}\left(\mathrm{1}−\sqrt{\mathrm{1}−\boldsymbol{\mathrm{t}}^{\mathrm{2}} }\right)+\boldsymbol{\mathrm{C}} \\ $$

Question Number 44778 Answers: 0 Comments: 0

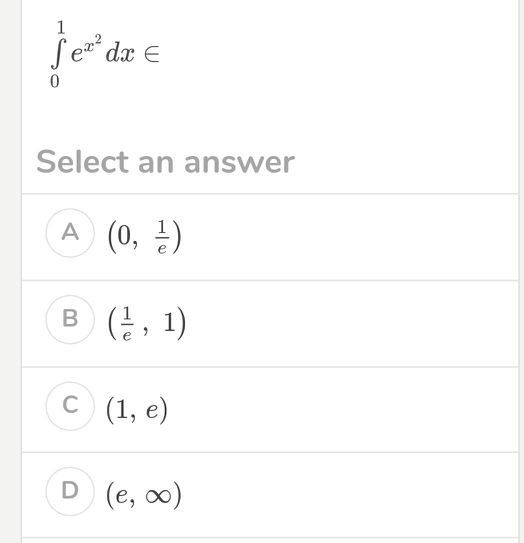
Question Number 44777 Answers: 0 Comments: 0

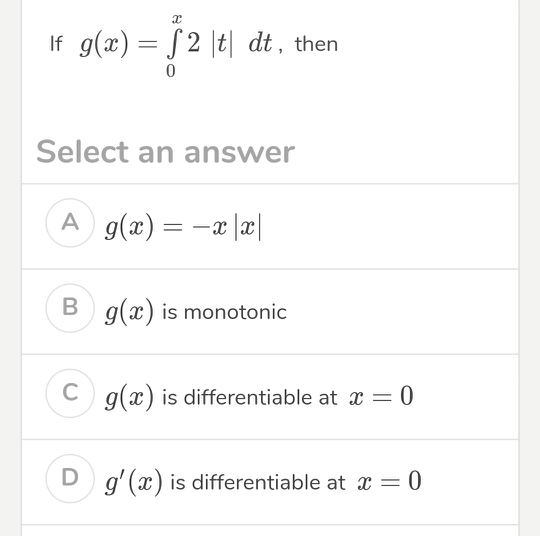
Question Number 44712 Answers: 3 Comments: 0

Question Number 44702 Answers: 0 Comments: 0

Question Number 44697 Answers: 0 Comments: 0

prove that:− ∫_0 ^∞ (t^(a−1) /(1+t))dt = (𝛑/(sin(𝛑a)))

$$\boldsymbol{{prove}}\:\boldsymbol{{that}}:− \\ $$$$\int_{\mathrm{0}} ^{\infty} \frac{\boldsymbol{{t}}^{\boldsymbol{{a}}−\mathrm{1}} }{\mathrm{1}+\boldsymbol{{t}}}\boldsymbol{{dt}}\:=\:\frac{\boldsymbol{\pi}}{\boldsymbol{{sin}}\left(\boldsymbol{\pi{a}}\right)} \\ $$

Question Number 44706 Answers: 0 Comments: 4

let f_α (x) = ((cos(αx))/(1+x^2 )) 1) calculate f^((n)) (x) and f^((n)) (0) 2) developp f at integr serie 3) give ∫_0 ^x f_α (t) dt at form of serie 4) developp ∫_0 ^∞ f_α (t)dt at integr serie .

$${let}\:{f}_{\alpha} \left({x}\right)\:=\:\frac{{cos}\left(\alpha{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}^{\left({n}\right)} \left({x}\right)\:{and}\:{f}^{\left({n}\right)} \left(\mathrm{0}\right) \\ $$$$\left.\mathrm{2}\right)\:{developp}\:{f}\:{at}\:{integr}\:{serie} \\ $$$$\left.\mathrm{3}\right)\:{give}\:\int_{\mathrm{0}} ^{{x}} \:{f}_{\alpha} \left({t}\right)\:{dt}\:\:{at}\:{form}\:{of}\:{serie}\: \\ $$$$\left.\mathrm{4}\right)\:{developp}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:{f}_{\alpha} \left({t}\right){dt}\:\:{at}\:\:{integr}\:{serie}\:. \\ $$

Question Number 44696 Answers: 1 Comments: 1

∫(1/(1+x^4 ))dx = ?

$$\int\frac{\mathrm{1}}{\mathrm{1}+\boldsymbol{\mathrm{x}}^{\mathrm{4}} }\boldsymbol{\mathrm{dx}}\:=\:? \\ $$

Pg 262 Pg 263 Pg 264 Pg 265 Pg 266 Pg 267 Pg 268 Pg 269 Pg 270 Pg 271

Contact: info@tinkutara.com