Question and Answers Forum

All Questions   Topic List

IntegrationQuestion and Answers: Page 26

Question Number 200604    Answers: 0   Comments: 2

Question Number 200603    Answers: 1   Comments: 0

Question Number 200602    Answers: 1   Comments: 0

Question Number 200601    Answers: 0   Comments: 6

Question Number 200586    Answers: 1   Comments: 0

Question Number 200570    Answers: 1   Comments: 0

Question Number 200569    Answers: 1   Comments: 0

Question Number 200553    Answers: 0   Comments: 0

∫_0 ^1 ((tan^(−1) (x))/( (√(1+x)))) dx =?

$$\:\:\:\:\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{x}\right)}{\:\sqrt{\mathrm{1}+\mathrm{x}}}\:\mathrm{dx}\:=?\: \\ $$

Question Number 200474    Answers: 1   Comments: 0

Question Number 200464    Answers: 1   Comments: 0

∫_0 ^(π/2) (dx/(1+tan^(2023) x))=???????

$$\underset{\mathrm{0}} {\overset{\frac{\pi}{\mathrm{2}}} {\int}}\frac{{dx}}{\mathrm{1}+\mathrm{tan}^{\mathrm{2023}} \:{x}}=??????? \\ $$

Question Number 200444    Answers: 1   Comments: 0

Question Number 200403    Answers: 1   Comments: 0

∫ (((x^2 + 1)dx)/(x(x−1)(x+1))) = ??

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\int\:\:\frac{\left(\boldsymbol{{x}}^{\mathrm{2}} \:+\:\:\mathrm{1}\right)\boldsymbol{{dx}}}{\boldsymbol{{x}}\left(\boldsymbol{{x}}−\mathrm{1}\right)\left(\boldsymbol{{x}}+\mathrm{1}\right)}\:=\:?? \\ $$$$ \\ $$

Question Number 200366    Answers: 1   Comments: 1

Question Number 200257    Answers: 1   Comments: 0

Question Number 200256    Answers: 0   Comments: 0

Question Number 200254    Answers: 1   Comments: 0

calculate ... Ω = ∫_(∫_0 ^( (π/2)) ln(tan(x))dx) ^( ∫_0 ^( ∞) ((sin^2 (x))/x^2 ) dx) ln(sin(x))dx=?

$$ \\ $$$$\:\:\:\:\:\:{calculate}\:... \\ $$$$\:\:\Omega\:=\:\int_{\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{tan}\left({x}\right)\right){dx}} ^{\:\int_{\mathrm{0}} ^{\:\infty} \:\frac{\mathrm{sin}^{\mathrm{2}} \left({x}\right)}{{x}^{\mathrm{2}} }\:{dx}} \mathrm{ln}\left(\mathrm{sin}\left({x}\right)\right){dx}=? \\ $$

Question Number 200253    Answers: 2   Comments: 0

Question Number 200250    Answers: 2   Comments: 0

Question Number 200159    Answers: 1   Comments: 2

Question Number 200155    Answers: 2   Comments: 0

Question Number 200130    Answers: 2   Comments: 0

solve by contour integrstion ∫_0 ^(2π) (dx/(1+acosx))

$$\:\:{solve}\:{by}\:{contour}\:{integrstion} \\ $$$$\:\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} \frac{{dx}}{\mathrm{1}+{a}\mathrm{cos}{x}}\: \\ $$

Question Number 200048    Answers: 0   Comments: 2

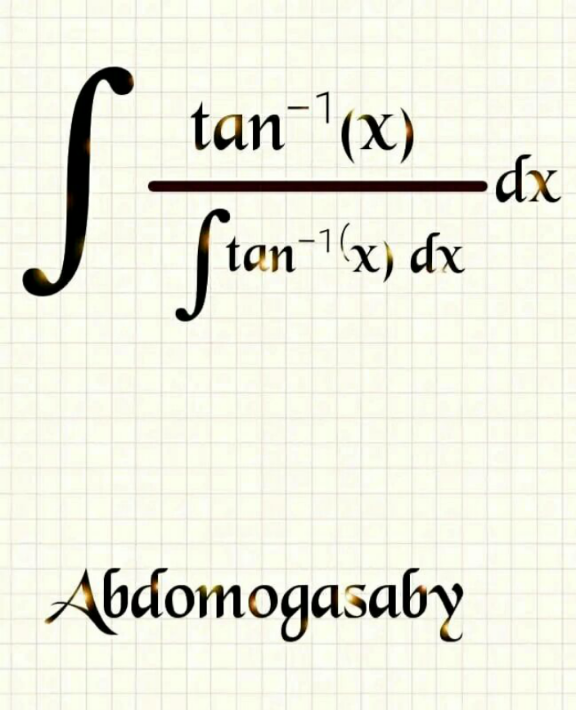
Question Number 200061    Answers: 1   Comments: 0

∫_(−∞) ^(+∞) ((xsinx )/((x^2 +1)(x^2 +4)))dx = ??

$$\:\:\:\:\int_{−\infty} ^{+\infty} \frac{{x}\mathrm{sin}{x}\:}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}^{\mathrm{2}} +\mathrm{4}\right)}{dx}\:\:=\:\:\:?? \\ $$

Question Number 199942    Answers: 1   Comments: 0

Question Number 199934    Answers: 2   Comments: 0

$$\:\:\:\cancel{\underline{\underbrace{ }}\:} \\ $$$$ \\ $$

Question Number 199921    Answers: 1   Comments: 0

  Pg 21      Pg 22      Pg 23      Pg 24      Pg 25      Pg 26      Pg 27      Pg 28      Pg 29      Pg 30   

Terms of Service

Privacy Policy

Contact: info@tinkutara.com