Question and Answers Forum

IntegrationQuestion and Answers: Page 248

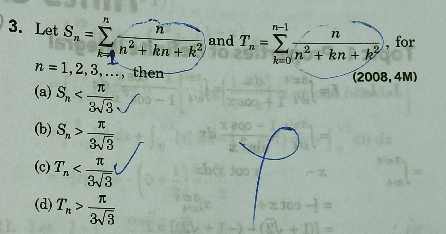
Question Number 57325 Answers: 0 Comments: 1

calculate ∫_0 ^(π/2) ((ln(1+sinx))/(sinx))dx

$${calculate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{ln}\left(\mathrm{1}+{sinx}\right)}{{sinx}}{dx} \\ $$

Question Number 57324 Answers: 0 Comments: 0

we want to find the vslue of I =∫_0 ^1 ((ln(1+x))/(1+x^2 )) dx let A=∫∫_W (x/((1+x^2 )(1+xy)))dxdy with W=[0,1]^2 calculate A by two method and conclude the value of I .

$${we}\:{want}\:{to}\:{find}\:{the}\:{vslue}\:{of} \\ $$$${I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }\:{dx}\:{let} \\ $$$${A}=\int\int_{{W}} \frac{{x}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\left(\mathrm{1}+{xy}\right)}{dxdy} \\ $$$${with}\:{W}=\left[\mathrm{0},\mathrm{1}\right]^{\mathrm{2}} \\ $$$${calculate}\:{A}\:{by}\:{two}\:{method}\:{and} \\ $$$${conclude}\:{the}\:{value}\:{of}\:{I}\:. \\ $$

Question Number 57323 Answers: 0 Comments: 1

calculate ∫∫_D ((x+y)/(3+(√(x^2 +y^2 ))))dxdy with D={(x,y)∈R^2 /x^2 +y^2 ≤2 and x≥0 ,y≥0}

$${calculate}\:\int\int_{{D}} \:\:\frac{{x}+{y}}{\mathrm{3}+\sqrt{{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} }}{dxdy} \\ $$$${with}\:{D}=\left\{\left({x},{y}\right)\in{R}^{\mathrm{2}} /{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} \leqslant\mathrm{2}\right. \\ $$$$\left.{and}\:{x}\geqslant\mathrm{0}\:,{y}\geqslant\mathrm{0}\right\} \\ $$

Question Number 57321 Answers: 1 Comments: 1

calculate ∫∫_D (x−y)(√(x^2 +y^2 ))dxdy with D ={ (x,y)∈R^2 /x^2 +y^2 ≤2 and x≥0}

$${calculate}\:\int\int_{{D}} \left({x}−{y}\right)\sqrt{{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} }{dxdy} \\ $$$${with}\:{D}\:=\left\{\:\left({x},{y}\right)\in{R}^{\mathrm{2}} /{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} \:\leqslant\mathrm{2}\:{and}\:{x}\geqslant\mathrm{0}\right\} \\ $$

Question Number 57320 Answers: 1 Comments: 1

calculate ∫∫_D xy e^(−x^2 −y^2 ) dxdy with D={(x,y)∈R^2 / 0≤x≤2 and 1≤y≤3}

$${calculate}\:\int\int_{{D}} {xy}\:{e}^{−{x}^{\mathrm{2}} −{y}^{\mathrm{2}} } \:{dxdy} \\ $$$${with}\:{D}=\left\{\left({x},{y}\right)\in{R}^{\mathrm{2}} /\:\mathrm{0}\leqslant{x}\leqslant\mathrm{2}\:{and}\right. \\ $$$$\left.\mathrm{1}\leqslant{y}\leqslant\mathrm{3}\right\} \\ $$

Question Number 57319 Answers: 1 Comments: 1

calculate ∫∫_D e^(x−y) dxdy with D={(x,y)∈R^2 /∣x∣<1 and 0≤y≤1}

$${calculate}\:\int\int_{{D}} \:{e}^{{x}−{y}} \:{dxdy} \\ $$$${with}\:{D}=\left\{\left({x},{y}\right)\in{R}^{\mathrm{2}} \:/\mid{x}\mid<\mathrm{1}\:{and}\:\mathrm{0}\leqslant{y}\leqslant\mathrm{1}\right\} \\ $$

Question Number 57228 Answers: 0 Comments: 1

find f(x) =∫_1 ^2 ((ln(1+xt))/t^2 ) dt with x>0

$${find}\:{f}\left({x}\right)\:=\int_{\mathrm{1}} ^{\mathrm{2}} \:\frac{{ln}\left(\mathrm{1}+{xt}\right)}{{t}^{\mathrm{2}} }\:{dt}\:\:{with}\:{x}>\mathrm{0} \\ $$

Question Number 57227 Answers: 0 Comments: 0

let f(α)=∫_0 ^1 ((arctan(αx))/(1+αx^2 )) dx with α real 1) find f(α) interms of α 2) find the values of ∫_0 ^1 ((arctan(2x))/(1+2x^2 )) dx and ∫_0 ^1 ((arctan(4x))/(1+4x^2 ))dx

$${let}\:{f}\left(\alpha\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{arctan}\left(\alpha{x}\right)}{\mathrm{1}+\alpha{x}^{\mathrm{2}} }\:{dx}\:\:\:{with}\:\alpha\:{real} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{f}\left(\alpha\right)\:{interms}\:{of}\:\alpha \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{values}\:{of}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{arctan}\left(\mathrm{2}{x}\right)}{\mathrm{1}+\mathrm{2}{x}^{\mathrm{2}} }\:{dx}\:{and}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{arctan}\left(\mathrm{4}{x}\right)}{\mathrm{1}+\mathrm{4}{x}^{\mathrm{2}} }{dx} \\ $$

Question Number 57226 Answers: 0 Comments: 0

calculate A_n =∫_0 ^1 x^n (√((1−x)/(1+x)))dx with n integr natural

$${calculate}\:{A}_{{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}^{{n}} \sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}{dx}\:\:{with}\:{n}\:{integr}\:{natural} \\ $$

Question Number 57224 Answers: 1 Comments: 1

find the value of ∫_0 ^1 ((3t^2 −5t +1)/((t+1)(t+2)(2t+3)))dt

$${find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{3}{t}^{\mathrm{2}} −\mathrm{5}{t}\:+\mathrm{1}}{\left({t}+\mathrm{1}\right)\left({t}+\mathrm{2}\right)\left(\mathrm{2}{t}+\mathrm{3}\right)}{dt} \\ $$

Question Number 57225 Answers: 0 Comments: 2

1)calculate f(a) =∫_0 ^a ((2x−1)/((x^2 −x+3)(x^2 +1)))dx 1) calculate f(1)and f(2)

$$\left.\mathrm{1}\right){calculate}\:{f}\left({a}\right)\:=\int_{\mathrm{0}} ^{{a}} \:\:\:\frac{\mathrm{2}{x}−\mathrm{1}}{\left({x}^{\mathrm{2}} \:−{x}+\mathrm{3}\right)\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)}{dx} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}\left(\mathrm{1}\right){and}\:{f}\left(\mathrm{2}\right) \\ $$

Question Number 57241 Answers: 1 Comments: 0

∫((×(√(x+1)))/(x+2))dx

$$\int\frac{×\sqrt{\mathrm{x}+\mathrm{1}}}{\mathrm{x}+\mathrm{2}}\mathrm{dx} \\ $$

Question Number 57194 Answers: 0 Comments: 1

let A_n =∫_n ^n (([(√(x+1))]−[(√x)])/x) dx with n natural integr and n≥1 1) find A_n interms of n 2)find nature of the serie Σ A_n

$${let}\:\:{A}_{{n}} =\int_{{n}} ^{{n}} \:\frac{\left[\sqrt{{x}+\mathrm{1}}\right]−\left[\sqrt{{x}}\right]}{{x}}\:{dx}\:\:\:{with}\:{n}\:{natural}\:{integr}\:{and}\:{n}\geqslant\mathrm{1} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{A}_{{n}} \:{interms}\:{of}\:{n} \\ $$$$\left.\mathrm{2}\right){find}\:{nature}\:{of}\:{the}\:{serie}\:\Sigma\:{A}_{{n}} \\ $$

Question Number 57237 Answers: 0 Comments: 2

let f(x) =∫_0 ^(+∞) ((sin(xt^2 −1))/(t^4 +1)) dt 1) find a explicit form of f(x) 2) let g(x) =∫_0 ^∞ ((t^2 cos(xt^2 −1))/(t^4 +1)) dt find a explicit form of g(x) 3) calculate ∫_0 ^∞ ((sin(2t^2 −1))/(t^4 +1)) dt and ∫_0 ^∞ ((t^2 cos(3t^2 −1))/(t^4 +1)) dt .

$${let}\:{f}\left({x}\right)\:=\int_{\mathrm{0}} ^{+\infty} \:\:\frac{{sin}\left({xt}^{\mathrm{2}} −\mathrm{1}\right)}{{t}^{\mathrm{4}} \:+\mathrm{1}}\:{dt} \\ $$$$\left.\mathrm{1}\right)\:{find}\:\:{a}\:{explicit}\:{form}\:{of}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{let}\:{g}\left({x}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}^{\mathrm{2}} \:{cos}\left({xt}^{\mathrm{2}} −\mathrm{1}\right)}{{t}^{\mathrm{4}} \:+\mathrm{1}}\:{dt} \\ $$$${find}\:{a}\:{explicit}\:{form}\:{of}\:{g}\left({x}\right) \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{sin}\left(\mathrm{2}{t}^{\mathrm{2}} −\mathrm{1}\right)}{{t}^{\mathrm{4}} \:+\mathrm{1}}\:{dt}\:\:{and}\:\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{t}^{\mathrm{2}} \:{cos}\left(\mathrm{3}{t}^{\mathrm{2}} −\mathrm{1}\right)}{{t}^{\mathrm{4}} \:+\mathrm{1}}\:{dt}\:. \\ $$

Question Number 57236 Answers: 1 Comments: 1

clalculate A_n = ∫_0 ^1 t^(2n) (1−t)^n dt with n integr natural .

$${clalculate}\:{A}_{{n}} =\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{t}^{\mathrm{2}{n}} \left(\mathrm{1}−{t}\right)^{{n}} {dt}\:\:\:{with}\:{n}\:{integr}\:{natural}\:. \\ $$

Question Number 57235 Answers: 1 Comments: 1

calculate ∫_0 ^(π/2) ((cosx −sinx)/(√(cos^8 x +sin^8 x))) dx

$${calculate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{cosx}\:−{sinx}}{\sqrt{{cos}^{\mathrm{8}} {x}\:+{sin}^{\mathrm{8}} {x}}}\:{dx} \\ $$

Question Number 57233 Answers: 0 Comments: 1

find the value of ∫_0 ^(+∞) (x^4 /((1+x^2 +x^4 )^2 ))dx

$${find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{+\infty} \:\:\frac{{x}^{\mathrm{4}} }{\left(\mathrm{1}+{x}^{\mathrm{2}} \:+{x}^{\mathrm{4}} \right)^{\mathrm{2}} }{dx} \\ $$

Question Number 57231 Answers: 0 Comments: 1

find tbe value of ∫_(−∞) ^(+∞) ((x−3)/((x^2 +1)(x^2 −x +2)^2 )) dx

$${find}\:{tbe}\:{value}\:{of}\:\int_{−\infty} ^{+\infty} \:\:\frac{{x}−\mathrm{3}}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)\left({x}^{\mathrm{2}} −{x}\:+\mathrm{2}\right)^{\mathrm{2}} }\:{dx} \\ $$

Question Number 57230 Answers: 0 Comments: 0

1) find ∫ (dx/(x^2 (x+1)^3 (x^2 +1))) 2) calculate ∫_1 ^(√3) (dx/(x^2 (x+1)^3 (x^2 +1)))

$$\left.\mathrm{1}\right)\:{find}\:\int\:\:\frac{{dx}}{{x}^{\mathrm{2}} \left({x}+\mathrm{1}\right)^{\mathrm{3}} \left({x}^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\int_{\mathrm{1}} ^{\sqrt{\mathrm{3}}} \:\:\:\:\:\frac{{dx}}{{x}^{\mathrm{2}} \left({x}+\mathrm{1}\right)^{\mathrm{3}} \left({x}^{\mathrm{2}} \:+\mathrm{1}\right)} \\ $$

Question Number 57229 Answers: 1 Comments: 1

give ∫_0 ^1 (x^5 /(x^3 +1)) dx at form of serie

$${give}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{x}^{\mathrm{5}} }{{x}^{\mathrm{3}} \:+\mathrm{1}}\:{dx}\:{at}\:{form}\:{of}\:{serie} \\ $$

Question Number 59160 Answers: 0 Comments: 0

let f(x) =∫_0 ^∞ ((cos(xcosθ))/(x^2 +θ^2 )) dθ and g(x) =∫_0 ^∞ ((sin(xcosθ))/(x^2 +θ^2 )) dθ 1) find a explicit form of f(x) and g(x) 2) find the value of ∫_0 ^∞ ((cos(2cosθ))/(4+θ^2 )) dθ and ∫_0 ^∞ ((sin(2cosθ))/(4+θ^2 )) dθ 3) let u_n =f(n^2 ) study the serie Σ u_n

$${let}\:{f}\left({x}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{cos}\left({xcos}\theta\right)}{{x}^{\mathrm{2}} \:+\theta^{\mathrm{2}} }\:{d}\theta\:\:\:\:\:\:{and}\:\:{g}\left({x}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\frac{{sin}\left({xcos}\theta\right)}{{x}^{\mathrm{2}} \:+\theta^{\mathrm{2}} }\:{d}\theta \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{explicit}\:{form}\:{of}\:{f}\left({x}\right)\:{and}\:{g}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{cos}\left(\mathrm{2}{cos}\theta\right)}{\mathrm{4}+\theta^{\mathrm{2}} }\:{d}\theta\:\:{and}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{sin}\left(\mathrm{2}{cos}\theta\right)}{\mathrm{4}+\theta^{\mathrm{2}} }\:{d}\theta \\ $$$$\left.\mathrm{3}\right)\:{let}\:{u}_{{n}} ={f}\left({n}^{\mathrm{2}} \right)\:\:\:{study}\:\:{the}\:{serie}\:\Sigma\:{u}_{{n}} \\ $$

Question Number 57103 Answers: 0 Comments: 2

let A_n =∫∫_w_n e^(−x^2 −y^2 ) (√(x^2 +y^2 ))dxdy with w_n =[(1/n),n]×[(1/n),n] 1) calculate A_n interms of n 2) find lim_(n→+∞) A_n

$${let}\:{A}_{{n}} =\int\int_{{w}_{{n}} } \:{e}^{−{x}^{\mathrm{2}} −{y}^{\mathrm{2}} } \:\sqrt{{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} }{dxdy} \\ $$$${with}\:{w}_{{n}} =\left[\frac{\mathrm{1}}{{n}},{n}\right]×\left[\frac{\mathrm{1}}{{n}},{n}\right] \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{A}_{{n}} {interms}\:{of}\:{n} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{lim}_{{n}\rightarrow+\infty} \:{A}_{{n}} \\ $$

Question Number 57060 Answers: 0 Comments: 0

Question Number 56939 Answers: 1 Comments: 2

calculate ∫ (dx/((x+1)^3 (x^2 −3x +2))) 2) find the value of ∫_2 ^(+∞) (dx/((x+1)^3 (x^2 −3x+2)))

$${calculate}\:\int\:\:\:\:\frac{{dx}}{\left({x}+\mathrm{1}\right)^{\mathrm{3}} \left({x}^{\mathrm{2}} −\mathrm{3}{x}\:+\mathrm{2}\right)} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:\int_{\mathrm{2}} ^{+\infty} \:\:\frac{{dx}}{\left({x}+\mathrm{1}\right)^{\mathrm{3}} \left({x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}\right)} \\ $$

Question Number 56938 Answers: 0 Comments: 0

let A_n =∫∫_W_n e^(−xy) (√(x^2 +y^2 ))dxdy with W_n =[(1/n),n[×[(1/n),n[ 1) find A_n interms of n 2) determine lim_(n→+∞) A_n

$${let}\:{A}_{{n}} =\int\int_{{W}_{{n}} } {e}^{−{xy}} \sqrt{{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} }{dxdy}\:\:\:{with}\:{W}_{{n}} =\left[\frac{\mathrm{1}}{{n}},{n}\left[×\left[\frac{\mathrm{1}}{{n}},{n}\left[\right.\right.\right.\right. \\ $$$$\left.\mathrm{1}\right)\:{find}\:{A}_{{n}} {interms}\:{of}\:{n} \\ $$$$\left.\mathrm{2}\right)\:{determine}\:{lim}_{{n}\rightarrow+\infty} \:{A}_{{n}} \\ $$$$ \\ $$

Question Number 56937 Answers: 0 Comments: 0

1. calculate f(x) =∫_0 ^(π/4) ln(1+xtanθ)dθ 2. calculate ∫_0 ^1 f(x)dx

$$\mathrm{1}.\:{calculate}\:\:{f}\left({x}\right)\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{ln}\left(\mathrm{1}+{xtan}\theta\right){d}\theta \\ $$$$\mathrm{2}.\:\:{calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} {f}\left({x}\right){dx} \\ $$

Pg 243 Pg 244 Pg 245 Pg 246 Pg 247 Pg 248 Pg 249 Pg 250 Pg 251 Pg 252

Contact: info@tinkutara.com