
IntegrationQuestion and Answers: Page 217
Question Number 71801 Answers: 0 Comments: 0
Question Number 71799 Answers: 2 Comments: 0
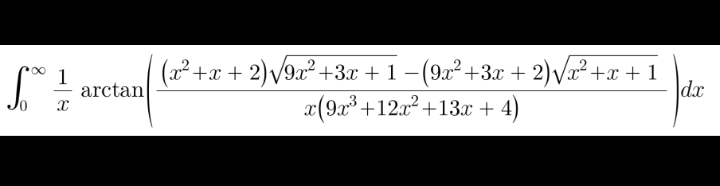
Question Number 71665 Answers: 1 Comments: 0
Question Number 71663 Answers: 1 Comments: 1
Question Number 71499 Answers: 1 Comments: 1

Question Number 71490 Answers: 2 Comments: 2

Question Number 71314 Answers: 2 Comments: 1
Question Number 71239 Answers: 2 Comments: 3
$$\int\frac{\mathrm{1}}{\mathrm{2cosx}−\mathrm{5sinx}−\mathrm{3}}\mathrm{dx} \\ $$
Question Number 71220 Answers: 0 Comments: 0

Question Number 71197 Answers: 0 Comments: 0

Question Number 71175 Answers: 1 Comments: 3
$$\int\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} +\mathrm{2016x}}\mathrm{dx} \\ $$
Question Number 71142 Answers: 0 Comments: 1
$${find}\:\int\sqrt{{x}\left({x}+\mathrm{1}\right)\left({x}+\mathrm{2}\right)}{dx} \\ $$
Question Number 71091 Answers: 0 Comments: 3
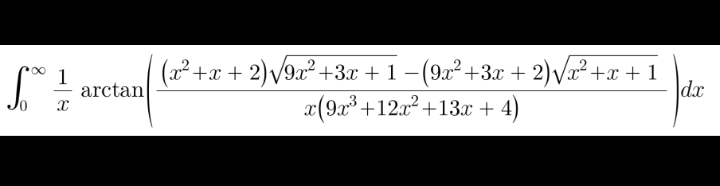
Question Number 71014 Answers: 0 Comments: 0
Question Number 70909 Answers: 1 Comments: 2

Question Number 70873 Answers: 1 Comments: 0
Question Number 70872 Answers: 0 Comments: 1
Question Number 70871 Answers: 0 Comments: 1
Question Number 70870 Answers: 0 Comments: 4
Question Number 70842 Answers: 0 Comments: 1
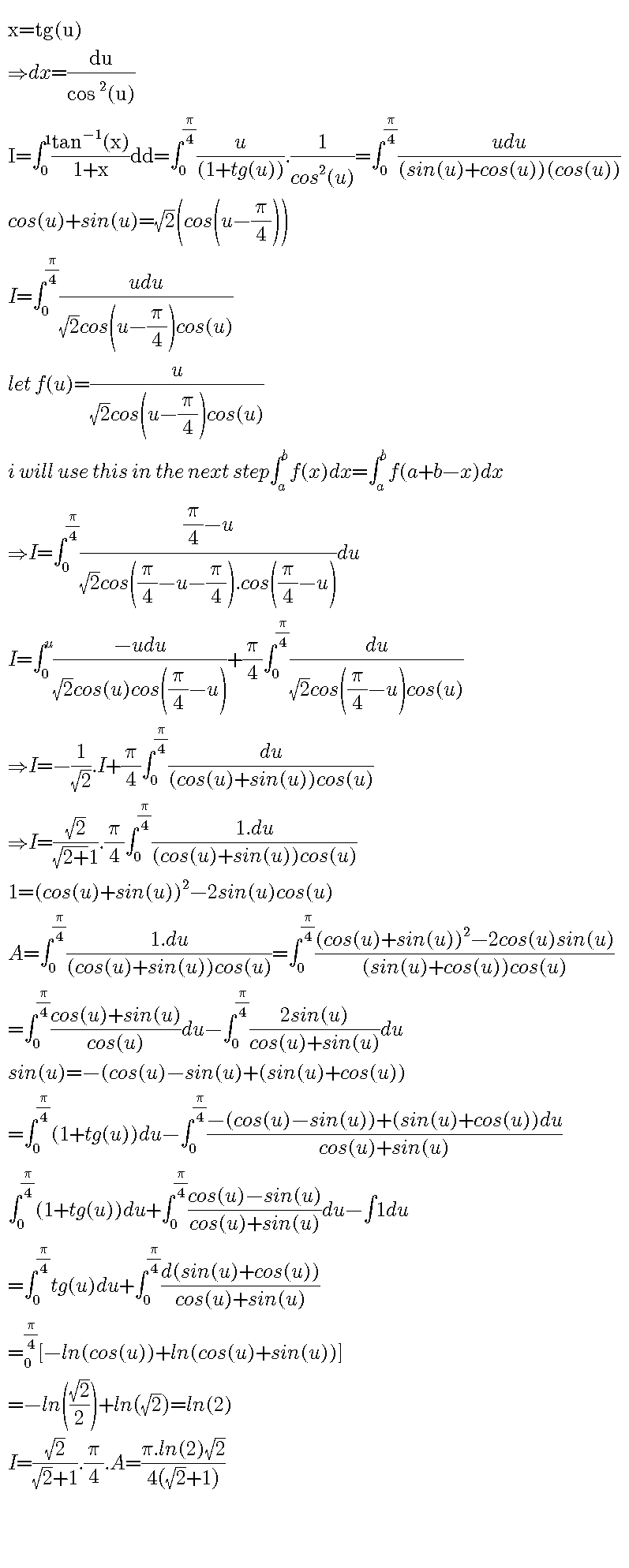
Question Number 70818 Answers: 1 Comments: 1
Question Number 70808 Answers: 1 Comments: 0
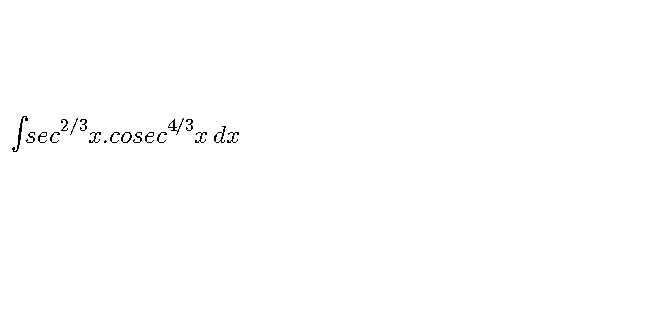
Question Number 70784 Answers: 1 Comments: 2

Question Number 70696 Answers: 1 Comments: 4
Question Number 70686 Answers: 2 Comments: 0
$${find}\:\int\:\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{6}} } \\ $$
Question Number 70651 Answers: 0 Comments: 3
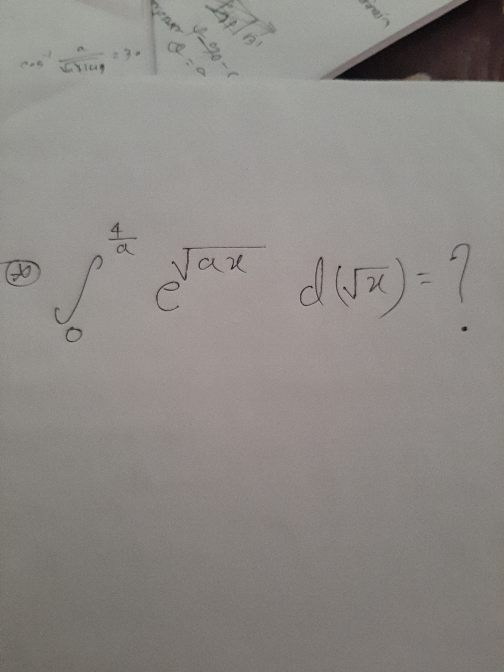
Pg 212 Pg 213 Pg 214 Pg 215 Pg 216 Pg 217 Pg 218 Pg 219 Pg 220 Pg 221
