
IntegrationQuestion and Answers: Page 194
Question Number 85813 Answers: 2 Comments: 1
$$\int\:\mathrm{x}^{\mathrm{2}} \:\sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\:\mathrm{dx}\:? \\ $$
Question Number 85391 Answers: 1 Comments: 5

Question Number 85384 Answers: 1 Comments: 1

Question Number 85383 Answers: 1 Comments: 0
Question Number 85362 Answers: 1 Comments: 0
Question Number 85360 Answers: 1 Comments: 0
Question Number 85355 Answers: 1 Comments: 4
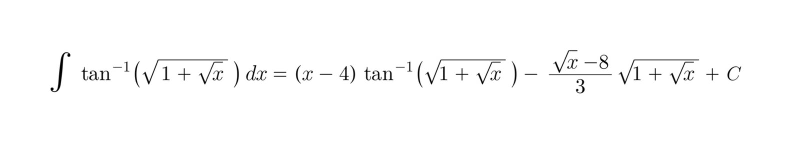
Question Number 85374 Answers: 0 Comments: 2
Question Number 85323 Answers: 1 Comments: 0
$$\int\frac{\mathrm{z}−\mathrm{3}}{\mathrm{5z}−\mathrm{10}}\mathrm{dz} \\ $$
Question Number 85321 Answers: 0 Comments: 0

Question Number 85319 Answers: 1 Comments: 0
$$\int\frac{\mathrm{2}+\mathrm{u}}{−\mathrm{u}^{\mathrm{2}} −\mathrm{u}}\mathrm{du} \\ $$
Question Number 85260 Answers: 1 Comments: 1
Question Number 85256 Answers: 0 Comments: 3
Question Number 85254 Answers: 0 Comments: 0

Question Number 85236 Answers: 1 Comments: 1
Question Number 85191 Answers: 2 Comments: 0
Question Number 85167 Answers: 0 Comments: 0
Question Number 85166 Answers: 1 Comments: 3
Question Number 85162 Answers: 0 Comments: 1
Question Number 85160 Answers: 1 Comments: 2
Question Number 85158 Answers: 0 Comments: 0
Question Number 85148 Answers: 0 Comments: 0
Question Number 85097 Answers: 1 Comments: 0
Question Number 85059 Answers: 1 Comments: 0
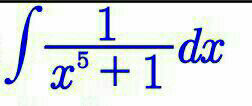
Question Number 85009 Answers: 1 Comments: 1
Question Number 84958 Answers: 0 Comments: 0

Pg 189 Pg 190 Pg 191 Pg 192 Pg 193 Pg 194 Pg 195 Pg 196 Pg 197 Pg 198
