
IntegrationQuestion and Answers: Page 167
Question Number 98445 Answers: 2 Comments: 0
Question Number 98444 Answers: 1 Comments: 0
Question Number 98428 Answers: 1 Comments: 0
Question Number 98426 Answers: 2 Comments: 0
Question Number 98423 Answers: 1 Comments: 0
Question Number 98382 Answers: 0 Comments: 2
Question Number 98338 Answers: 2 Comments: 0
$$\int{cos}\left({x}^{\mathrm{18}} \right)\:{dx} \\ $$$$ \\ $$
Question Number 98311 Answers: 1 Comments: 0
Question Number 98305 Answers: 2 Comments: 0
Question Number 98271 Answers: 2 Comments: 5
Question Number 98256 Answers: 2 Comments: 0
Question Number 98249 Answers: 0 Comments: 0
Question Number 98248 Answers: 0 Comments: 0
Question Number 98246 Answers: 1 Comments: 0
$$\int\frac{{x}}{{sin}^{\mathrm{2}} \left({x}−\mathrm{3}\right)}{dx} \\ $$
Question Number 98245 Answers: 0 Comments: 0
Question Number 98214 Answers: 2 Comments: 2
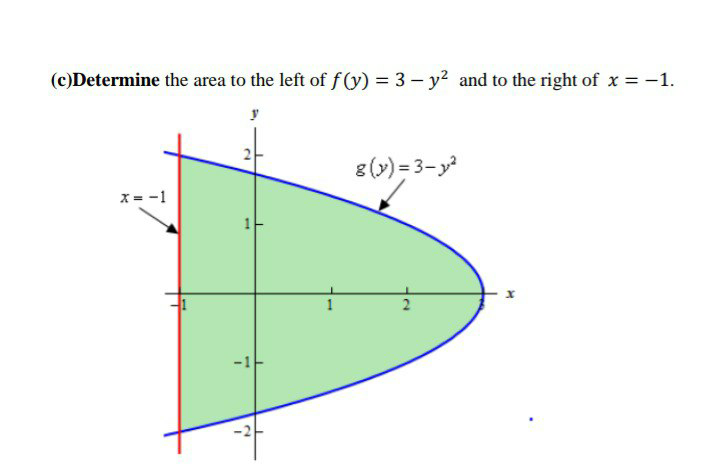
Question Number 98182 Answers: 2 Comments: 0
Question Number 98181 Answers: 0 Comments: 0
Question Number 98179 Answers: 2 Comments: 0
Question Number 98151 Answers: 2 Comments: 0
Question Number 98105 Answers: 2 Comments: 0
Question Number 98020 Answers: 1 Comments: 0
Question Number 98016 Answers: 1 Comments: 3
Question Number 97928 Answers: 1 Comments: 0
Question Number 97839 Answers: 3 Comments: 1
Question Number 97800 Answers: 3 Comments: 0
Pg 162 Pg 163 Pg 164 Pg 165 Pg 166 Pg 167 Pg 168 Pg 169 Pg 170 Pg 171
