
IntegrationQuestion and Answers: Page 162
Question Number 101597 Answers: 0 Comments: 3
$$\:\int\:\mathrm{ln}\:\left(\mathrm{1}+\:{e}^{{x}} \right)\:{dx}\:=\:.. \\ $$
Question Number 101585 Answers: 1 Comments: 0
Question Number 101860 Answers: 1 Comments: 1
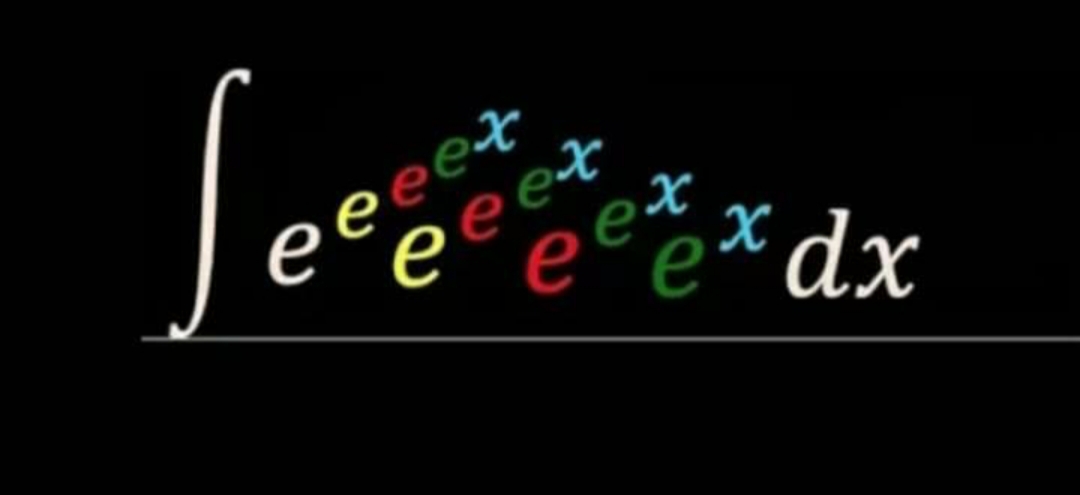
Question Number 101531 Answers: 1 Comments: 0
Question Number 101493 Answers: 1 Comments: 0
Question Number 101486 Answers: 0 Comments: 0

Question Number 101461 Answers: 1 Comments: 0
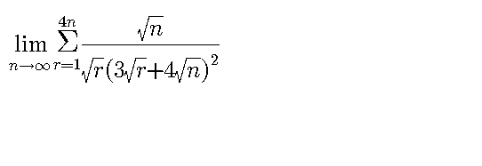
Question Number 101451 Answers: 2 Comments: 1

Question Number 105239 Answers: 1 Comments: 0
Question Number 101378 Answers: 2 Comments: 1
Question Number 101373 Answers: 0 Comments: 1
Question Number 101345 Answers: 0 Comments: 3
Question Number 101328 Answers: 0 Comments: 1
Question Number 101272 Answers: 1 Comments: 2
Question Number 101271 Answers: 0 Comments: 2
Question Number 101270 Answers: 0 Comments: 0
Question Number 101269 Answers: 1 Comments: 0
Question Number 101268 Answers: 1 Comments: 0
Question Number 101266 Answers: 0 Comments: 0
Question Number 101286 Answers: 0 Comments: 3
Question Number 101234 Answers: 0 Comments: 0
Question Number 101220 Answers: 1 Comments: 0
Question Number 101285 Answers: 0 Comments: 1
Question Number 101277 Answers: 2 Comments: 0
Question Number 101192 Answers: 1 Comments: 2
$$\int\:\frac{{x}}{\mathrm{1}+\mathrm{sin}\:{x}}\:{dx}\: \\ $$
Question Number 101178 Answers: 2 Comments: 0
Pg 157 Pg 158 Pg 159 Pg 160 Pg 161 Pg 162 Pg 163 Pg 164 Pg 165 Pg 166
