Question and Answers Forum

IntegrationQuestion and Answers: Page 154

Question Number 107245 Answers: 0 Comments: 0

♣ Question♣ Why ??? ....∫_0 ^( (π/2)) (√((((2^x −1)sin^3 (x))/((2^x +1)(sin^3 (x)+cos^3 (x)))) ))<(π/8) ....M.N....

$$\:\:\:\:\:\:\:\:\clubsuit\:\mathscr{Q}{uestion}\clubsuit \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{W}{hy}\:??? \\ $$$$....\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \sqrt{\frac{\left(\mathrm{2}^{{x}} −\mathrm{1}\right){sin}^{\mathrm{3}} \left({x}\right)}{\left(\mathrm{2}^{{x}} +\mathrm{1}\right)\left({sin}^{\mathrm{3}} \left({x}\right)+{cos}^{\mathrm{3}} \left({x}\right)\right)}\:}<\frac{\pi}{\mathrm{8}} \\ $$$$\:\:\:\:\:\:\:....\mathscr{M}.\mathscr{N}.... \\ $$

Question Number 107212 Answers: 4 Comments: 0

⊚bemath⊚ ∫ x^6 (√(1−x^2 )) dx ?

$$\:\:\:\circledcirc{bemath}\circledcirc \\ $$$$\int\:{x}^{\mathrm{6}} \:\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }\:{dx}\:? \\ $$

Question Number 107238 Answers: 3 Comments: 0

⊞bemath⊞ ∫ ((sin x)/(sin^3 x+cos^3 x)) dx ?

$$\:\:\:\:\:\:\:\boxplus{bemath}\boxplus \\ $$$$\int\:\frac{\mathrm{sin}\:{x}}{\mathrm{sin}\:^{\mathrm{3}} {x}+\mathrm{cos}\:^{\mathrm{3}} {x}}\:{dx}\:?\: \\ $$

Question Number 107197 Answers: 0 Comments: 0

⊵JS⊴ ∫ _0 ^( π/6) sin^2 (6x) cos^4 (3x) dx ? [ by using the Gamma function ]

$$\:\:\:\:\:\trianglerighteq\mathrm{JS}\trianglelefteq \\ $$$$\int\overset{\:\pi/\mathrm{6}} {\:}_{\mathrm{0}} \mathrm{sin}\:^{\mathrm{2}} \left(\mathrm{6x}\right)\:\mathrm{cos}\:^{\mathrm{4}} \left(\mathrm{3x}\right)\:\mathrm{dx}\:? \\ $$$$\left[\:\mathrm{by}\:\mathrm{using}\:\mathrm{the}\:\mathcal{G}\mathrm{amma}\:\mathrm{function}\:\right] \\ $$

Question Number 107193 Answers: 1 Comments: 0

∫_0 ^4 ((ln x)/(√(4x−x^2 ))) dx ?

$$\underset{\mathrm{0}} {\overset{\mathrm{4}} {\int}}\:\frac{\mathrm{ln}\:\mathrm{x}}{\sqrt{\mathrm{4x}−\mathrm{x}^{\mathrm{2}} }}\:\mathrm{dx}\:? \\ $$

Question Number 107184 Answers: 2 Comments: 0

@bemath@ ∫_0 ^2 x ((8−x^3 ))^(1/3) dx =?

$$\:\:\:\:\:\:\:@{bemath}@ \\ $$$$\underset{\mathrm{0}} {\overset{\mathrm{2}} {\int}}\:{x}\:\sqrt[{\mathrm{3}}]{\mathrm{8}−{x}^{\mathrm{3}} }\:{dx}\:=?\: \\ $$

Question Number 107162 Answers: 1 Comments: 1

Question Number 107141 Answers: 0 Comments: 0

∫(x^3 +x^6 )(((x^3 +2))^(1/3) )dx

$$\int\left({x}^{\mathrm{3}} +{x}^{\mathrm{6}} \right)\left(\sqrt[{\mathrm{3}}]{{x}^{\mathrm{3}} +\mathrm{2}}\right){dx} \\ $$

Question Number 107113 Answers: 0 Comments: 0

Question Number 107107 Answers: 1 Comments: 2

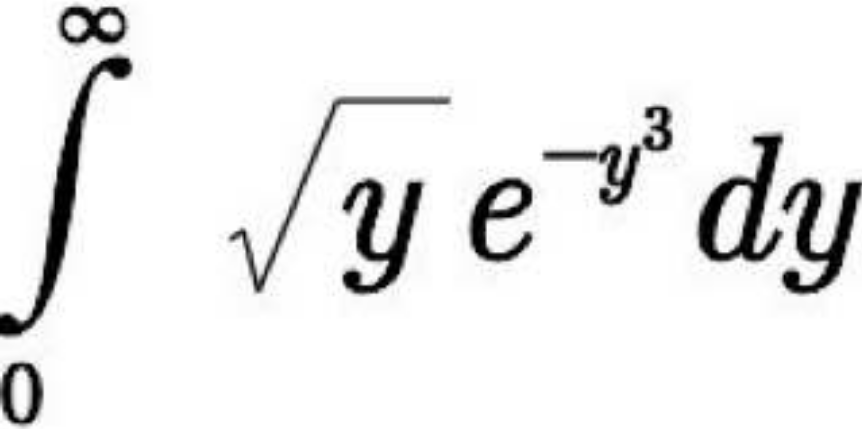
Question Number 107036 Answers: 2 Comments: 1

∫ (((√(1+x^2 )) dx)/x^4 ) ?

$$\int\:\frac{\sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\:\mathrm{dx}}{\mathrm{x}^{\mathrm{4}} }\:? \\ $$

Question Number 107002 Answers: 3 Comments: 0

∫_0 ^π ((cosx)/(√(2−sin^2 x)))dx

$$\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{cosx}}{\sqrt{\mathrm{2}−\mathrm{sin}^{\mathrm{2}} \mathrm{x}}}\mathrm{dx} \\ $$

Question Number 106964 Answers: 5 Comments: 4

∫_0 ^π ((sec^2 x)/(√(1−tan^2 x)))dx

$$\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{sec}^{\mathrm{2}} \mathrm{x}}{\sqrt{\mathrm{1}−\mathrm{tan}^{\mathrm{2}} \mathrm{x}}}\mathrm{dx} \\ $$

Question Number 106948 Answers: 4 Comments: 0

let f(a) =∫_0 ^π (dx/(a+cos^2 x)) with a>0 1) explicite f(a) 2)explicite g(a) =∫_0 ^π (dx/((a+cos^2 x)^2 )) 3) find tbe valued of intevrsls ∫_0 ^π (dx/(1+cos^2 x)) and ∫_0 ^π (dx/((1+cos^2 x)^2 ))

$$\mathrm{let}\:\mathrm{f}\left(\mathrm{a}\right)\:=\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dx}}{\mathrm{a}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\:\mathrm{with}\:\mathrm{a}>\mathrm{0} \\ $$$$\left.\mathrm{1}\right)\:\mathrm{explicite}\:\mathrm{f}\left(\mathrm{a}\right) \\ $$$$\left.\mathrm{2}\right)\mathrm{explicite}\:\mathrm{g}\left(\mathrm{a}\right)\:=\int_{\mathrm{0}} ^{\pi} \:\:\frac{\mathrm{dx}}{\left(\mathrm{a}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}\right)^{\mathrm{2}} } \\ $$$$\left.\mathrm{3}\right)\:\mathrm{find}\:\mathrm{tbe}\:\mathrm{valued}\:\mathrm{of}\:\mathrm{intevrsls} \\ $$$$\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dx}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\:\mathrm{and}\:\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dx}}{\left(\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}\right)^{\mathrm{2}} } \\ $$

Question Number 107051 Answers: 2 Comments: 0

∫_0 ^π ((cosx)/(2+sin^2 x))dx

$$\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{cosx}}{\mathrm{2}+\mathrm{sin}^{\mathrm{2}} \mathrm{x}}\mathrm{dx} \\ $$

Question Number 106932 Answers: 3 Comments: 0

∫_0 ^π (x/(1+cos^2 x))dx

$$\int_{\mathrm{0}} ^{\pi} \frac{\mathrm{x}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\mathrm{dx} \\ $$

Question Number 106908 Answers: 1 Comments: 0

Question Number 106899 Answers: 1 Comments: 0

calculate ∫_0 ^∞ ((tsin(2t))/(t^2 +4)) dt

$$\mathrm{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{tsin}\left(\mathrm{2t}\right)}{\mathrm{t}^{\mathrm{2}} +\mathrm{4}}\:\mathrm{dt} \\ $$

Question Number 106867 Answers: 2 Comments: 3

∀n∈(0, 1)∀x∈R : f(x) = (n/(n−1))x+n The given function gives a linear line that goes through points (0, n) and (1−n, 0). The function changes as n changes. What area is beneath the shape made as n goes from 0→1?

$$\forall{n}\in\left(\mathrm{0},\:\mathrm{1}\right)\forall{x}\in\mathbb{R}\::\:{f}\left({x}\right)\:=\:\frac{{n}}{{n}−\mathrm{1}}{x}+{n} \\ $$$$\mathrm{The}\:\mathrm{given}\:\mathrm{function}\:\mathrm{gives}\:\mathrm{a}\:\mathrm{linear}\:\mathrm{line}\:\mathrm{that} \\ $$$$\mathrm{goes}\:\mathrm{through}\:\mathrm{points}\:\left(\mathrm{0},\:{n}\right)\:\mathrm{and}\:\left(\mathrm{1}−{n},\:\mathrm{0}\right). \\ $$$$\mathrm{The}\:\mathrm{function}\:\mathrm{changes}\:\mathrm{as}\:{n}\:\mathrm{changes}. \\ $$$$\mathrm{What}\:\mathrm{area}\:\mathrm{is}\:\mathrm{beneath}\:\mathrm{the}\:\mathrm{shape}\:\mathrm{made}\:\mathrm{as} \\ $$$${n}\:\mathrm{goes}\:\mathrm{from}\:\mathrm{0}\rightarrow\mathrm{1}? \\ $$

Question Number 106830 Answers: 1 Comments: 7

∫(( dx)/((√(x^3 )) ^3 (√(1 +^4 (√x^3 ))))) = ?

$$\int\frac{\:{dx}}{\sqrt{{x}^{\mathrm{3}} \:}\:\:^{\mathrm{3}} \sqrt{\mathrm{1}\:+\:^{\mathrm{4}} \sqrt{{x}^{\mathrm{3}} }}}\:=\:? \\ $$

Question Number 106809 Answers: 2 Comments: 0

∫e^(2x) sine^x dx=??

$$\int{e}^{\mathrm{2}{x}} {sine}^{{x}} {dx}=?? \\ $$

Question Number 106808 Answers: 0 Comments: 0

∫tan(lnx)dx=???

$$\int{tan}\left({lnx}\right){dx}=??? \\ $$

Question Number 106807 Answers: 1 Comments: 0

∫sin(ln3x)dx=???