Question and Answers Forum

IntegrationQuestion and Answers: Page 151

Question Number 109097 Answers: 1 Comments: 0

((♭o♭hans)/(∼∼∼∼∼)) ∫_1 ^2 x sec^(−1) (x)dx=?

$$\:\:\frac{\boldsymbol{\flat{o}\flat{hans}}}{\sim\sim\sim\sim\sim} \\ $$$$\underset{\mathrm{1}} {\overset{\mathrm{2}} {\int}}{x}\:\mathrm{sec}^{−\mathrm{1}} \left({x}\right){dx}=? \\ $$

Question Number 109086 Answers: 1 Comments: 0

♭_→ o_→ ♭h_⊸ ans_⊸ (1) (x^2 e^(−(y/x)) +y^2 ) dx = xy dy (2)(((f(x))/x))′ = x^2 e^(−x^2 ) ; f(1) = (1/e) g(x) = (4/e^4 )∫_1 ^x e^t^2 f(t) dt . find f(2)−g(2)

$$\:\:\:\underset{\rightarrow} {\flat}\underset{\rightarrow} {{o}}\flat\underset{\multimap} {{h}an}\underset{\multimap} {{s}} \\ $$$$\left(\mathrm{1}\right)\:\left({x}^{\mathrm{2}} {e}^{−\frac{{y}}{{x}}} +{y}^{\mathrm{2}} \right)\:{dx}\:=\:{xy}\:{dy}\: \\ $$$$\left(\mathrm{2}\right)\left(\frac{{f}\left({x}\right)}{{x}}\right)'\:=\:{x}^{\mathrm{2}} {e}^{−{x}^{\mathrm{2}} } \:;\:{f}\left(\mathrm{1}\right)\:=\:\frac{\mathrm{1}}{{e}}\: \\ $$$$\:\:\:\:\:\:\:{g}\left({x}\right)\:=\:\frac{\mathrm{4}}{{e}^{\mathrm{4}} }\underset{\mathrm{1}} {\overset{{x}} {\int}}{e}^{{t}^{\mathrm{2}} } \:{f}\left({t}\right)\:{dt}\:.\:{find}\:{f}\left(\mathrm{2}\right)−{g}\left(\mathrm{2}\right) \\ $$

Question Number 109047 Answers: 1 Comments: 0

∫_(−2) ^∞ (x+2)^5 e^(−(x+2)) dx ∫_0 ^1 ((tan(x))/x)dx

$$\int_{−\mathrm{2}} ^{\infty} \left({x}+\mathrm{2}\right)^{\mathrm{5}} {e}^{−\left({x}+\mathrm{2}\right)} {dx} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{tan}\left({x}\right)}{{x}}{dx} \\ $$

Question Number 108998 Answers: 0 Comments: 0

Question Number 108990 Answers: 2 Comments: 0

Question Number 108921 Answers: 3 Comments: 0

a. ∫((sin^3 4x)/(cos^8 4x))dx b. ∫_(−(π/2)) ^(π/2) (x^2 e^(cosx) −2x)sinxdx

$$\mathrm{a}.\:\:\int\frac{\mathrm{sin}^{\mathrm{3}} \mathrm{4x}}{\mathrm{cos}^{\mathrm{8}} \mathrm{4x}}\mathrm{dx} \\ $$$$\mathrm{b}.\:\:\int_{−\frac{\pi}{\mathrm{2}}} ^{\frac{\pi}{\mathrm{2}}} \left(\mathrm{x}^{\mathrm{2}} \mathrm{e}^{\mathrm{cosx}} −\mathrm{2x}\right)\mathrm{sinxdx} \\ $$

Question Number 108908 Answers: 0 Comments: 0

Question Number 108954 Answers: 1 Comments: 5

Evaluate : Ω=∫_0 ^( 1) ∫_0 ^( 1) (1/(2−x^2 − y^2 )) dxdy=??? ★★♣♣★★

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathscr{E}{valuate}\:: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Omega=\int_{\mathrm{0}} ^{\:\mathrm{1}} \int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\mathrm{1}}{\mathrm{2}−{x}^{\mathrm{2}} \:−\:{y}^{\mathrm{2}} }\:{dxdy}=???\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\bigstar\bigstar\clubsuit\clubsuit\bigstar\bigstar \\ $$$$ \\ $$

Question Number 108893 Answers: 6 Comments: 0

(1)∫ (x^4 /(1−x^2 )) dx (2)∫_(−3) ^5 (√(∣x∣^3 )) dx (3) ∫_0 ^(π^2 /4) sin (√x) dx (4) ∫_(−∞) ^∞ e^(−2x^2 −5x−3) dx (5) x^3 y′′′−2x^2 y′′−2xy′+8y=0 (6)(x^4 +y^4 )dx+2x^3 y dy = 0 (7) (2(√(xy))−y)dx−xdy = 0

$$\left(\mathrm{1}\right)\int\:\frac{{x}^{\mathrm{4}} }{\mathrm{1}−{x}^{\mathrm{2}} }\:{dx}\: \\ $$$$\left(\mathrm{2}\right)\underset{−\mathrm{3}} {\overset{\mathrm{5}} {\int}}\sqrt{\mid{x}\mid^{\mathrm{3}} }\:{dx}\: \\ $$$$\left(\mathrm{3}\right)\:\underset{\mathrm{0}} {\overset{\frac{\pi^{\mathrm{2}} }{\mathrm{4}}} {\int}}\:\mathrm{sin}\:\sqrt{{x}}\:{dx}\: \\ $$$$\left(\mathrm{4}\right)\:\underset{−\infty} {\overset{\infty} {\int}}{e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{5}{x}−\mathrm{3}} \:{dx}\: \\ $$$$\left(\mathrm{5}\right)\:{x}^{\mathrm{3}} {y}'''−\mathrm{2}{x}^{\mathrm{2}} {y}''−\mathrm{2}{xy}'+\mathrm{8}{y}=\mathrm{0} \\ $$$$\left(\mathrm{6}\right)\left({x}^{\mathrm{4}} +{y}^{\mathrm{4}} \right){dx}+\mathrm{2}{x}^{\mathrm{3}} {y}\:{dy}\:=\:\mathrm{0} \\ $$$$\left(\mathrm{7}\right)\:\left(\mathrm{2}\sqrt{{xy}}−{y}\right){dx}−{xdy}\:=\:\mathrm{0} \\ $$

Question Number 108891 Answers: 1 Comments: 0

((bobHans)/∦) ∫ (((x^2 −2) dx)/((x^4 +5x^2 +4) arc tan (((x^2 +2)/x))))

$$\:\:\:\frac{\boldsymbol{{bob}}\mathbb{H}{ans}}{\nparallel} \\ $$$$\int\:\frac{\left({x}^{\mathrm{2}} −\mathrm{2}\right)\:{dx}}{\left({x}^{\mathrm{4}} +\mathrm{5}{x}^{\mathrm{2}} +\mathrm{4}\right)\:\mathrm{arc}\:\mathrm{tan}\:\left(\frac{{x}^{\mathrm{2}} +\mathrm{2}}{{x}}\right)} \\ $$

Question Number 108841 Answers: 1 Comments: 0

Question Number 108839 Answers: 0 Comments: 1

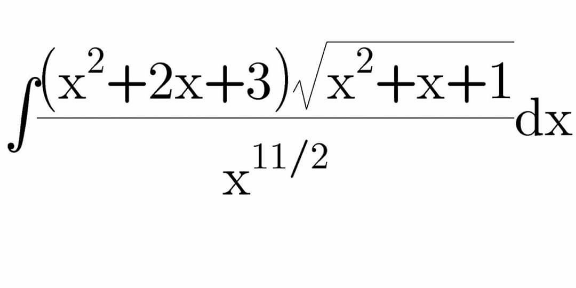
Question Number 108838 Answers: 0 Comments: 0

Question Number 108821 Answers: 1 Comments: 0

find ∫_0 ^∞ ((lnx)/((x^2 +1)^2 ))dx

$$\mathrm{find}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{lnx}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }\mathrm{dx} \\ $$

Question Number 108789 Answers: 1 Comments: 0

Question Number 108786 Answers: 1 Comments: 0

Question Number 108761 Answers: 2 Comments: 0

((⋮((Be)/(Math))⋮)/★) If ∫_(−1) ^( a) ((x+1)/((x+2)^4 )) = ((10)/(81)) , then the value of a−2 is ___

$$\:\:\:\frac{\vdots\frac{\mathcal{B}{e}}{\mathcal{M}{ath}}\vdots}{\bigstar} \\ $$$${If}\:\int_{−\mathrm{1}} ^{\:\:{a}} \:\frac{{x}+\mathrm{1}}{\left({x}+\mathrm{2}\right)^{\mathrm{4}} }\:=\:\frac{\mathrm{10}}{\mathrm{81}}\:,\:{then}\:{the}\:{value}\:{of} \\ $$$${a}−\mathrm{2}\:{is}\:\_\_\_ \\ $$

Question Number 108750 Answers: 2 Comments: 0

calculste ∫_0 ^∞ ((ln(x))/(x^2 −x+1))dx

$$\mathrm{calculste}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{ln}\left(\mathrm{x}\right)}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}}\mathrm{dx} \\ $$

Question Number 108749 Answers: 2 Comments: 0

calculste ∫_0 ^∞ ((ln(x))/((1+x)^4 )) dx

$$\mathrm{calculste}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{ln}\left(\mathrm{x}\right)}{\left(\mathrm{1}+\mathrm{x}\right)^{\mathrm{4}} }\:\mathrm{dx} \\ $$

Question Number 108748 Answers: 1 Comments: 0

Question Number 108738 Answers: 0 Comments: 0

please: ^∗ prove^∗ :::: 1.^(important) lim_(z→1) (ζ (z) −(1/(z−1)) )= γ (euler constant) 2. ^(important) ∫_0 ^( ∞) (cos(x)−(1/(1+x^2 )))(dx/x) =− γ .....M.N.....

$$\:\:\:\:\:\:\:\:{please}:\:\:\:\:\:^{\ast} \mathrm{prove}^{\ast} :::: \\ $$$$\:\:\:\:\:\mathrm{1}.^{\mathrm{important}} \:\:\:\:\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{1}} \left(\zeta\:\left(\mathrm{z}\right)\:−\frac{\mathrm{1}}{\mathrm{z}−\mathrm{1}}\:\right)=\:\gamma\:\:\:\left(\mathrm{euler}\:\mathrm{constant}\right) \\ $$$$\:\:\:\:\mathrm{2}.\:\overset{\mathrm{important}} {\:}\:\:\int_{\mathrm{0}} ^{\:\infty} \left(\mathrm{cos}\left(\mathrm{x}\right)−\frac{\mathrm{1}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\right)\frac{\mathrm{dx}}{\mathrm{x}}\:=−\:\gamma \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:.....\mathscr{M}.\mathscr{N}..... \\ $$$$\: \\ $$

Question Number 108723 Answers: 2 Comments: 1

Question Number 108710 Answers: 0 Comments: 1

calculate ∫_(−∞) ^∞ (((−1)^x^2 )/((x^2 +x+1)^2 ))dx

$$\mathrm{calculate}\:\:\int_{−\infty} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{\mathrm{x}^{\mathrm{2}} } }{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)^{\mathrm{2}} }\mathrm{dx} \\ $$

Question Number 108697 Answers: 1 Comments: 0

Question Number 108692 Answers: 2 Comments: 0

Question Number 108667 Answers: 4 Comments: 0

Pg 146 Pg 147 Pg 148 Pg 149 Pg 150 Pg 151 Pg 152 Pg 153 Pg 154 Pg 155

Contact: info@tinkutara.com