Question and Answers Forum

IntegrationQuestion and Answers: Page 150

Question Number 109891 Answers: 1 Comments: 0

((Δbe▽)/(math)) ∫ ((arc tan x)/((1+(1/x^2 )))) dx ?

$$\:\:\frac{\Delta{be}\bigtriangledown}{{math}} \\ $$$$\int\:\frac{\mathrm{arc}\:\mathrm{tan}\:{x}}{\left(\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }\right)}\:{dx}\:? \\ $$

Question Number 109884 Answers: 1 Comments: 1

Question Number 109872 Answers: 2 Comments: 1

((★be★)/(Math)) ∫ ((cos x dx)/(sin^2 x+4sin x−5)) ?

$$\:\:\frac{\bigstar{be}\bigstar}{\mathcal{M}{ath}} \\ $$$$\int\:\frac{\mathrm{cos}\:{x}\:{dx}}{\mathrm{sin}\:^{\mathrm{2}} {x}+\mathrm{4sin}\:{x}−\mathrm{5}}\:? \\ $$

Question Number 109849 Answers: 0 Comments: 0

Question Number 109839 Answers: 4 Comments: 1

((JS)/(≈♥≈)) (1) ∫ (((√(x+1))−(√(x−1)))/( (√(x+1))+(√(x−1)))) dx (2) ∫ ((√(tan x))/(1+(√(tan x)) )) dx

$$\:\frac{{JS}}{\approx\heartsuit\approx} \\ $$$$\left(\mathrm{1}\right)\:\int\:\frac{\sqrt{{x}+\mathrm{1}}−\sqrt{{x}−\mathrm{1}}}{\:\sqrt{{x}+\mathrm{1}}+\sqrt{{x}−\mathrm{1}}}\:{dx} \\ $$$$\left(\mathrm{2}\right)\:\int\:\frac{\sqrt{\mathrm{tan}\:{x}}}{\mathrm{1}+\sqrt{\mathrm{tan}\:{x}}\:}\:{dx}\: \\ $$

Question Number 109838 Answers: 1 Comments: 0

please prove::: ∫_0 ^( 1) (1/( (√(1−x))))log((x/(1−x)))dx =4log(2)

$$ \\ $$$$ \\ $$$$\:\:\:\:{please}\:{prove}::: \\ $$$$\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−{x}}}{log}\left(\frac{{x}}{\mathrm{1}−{x}}\right){dx}\:=\mathrm{4}{log}\left(\mathrm{2}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$ \\ $$$$ \\ $$

Question Number 109801 Answers: 0 Comments: 2

calculste ∫_0 ^1 (√(1+x^6 ))dx

$$\mathrm{calculste}\:\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{6}} }\mathrm{dx} \\ $$

Question Number 109794 Answers: 0 Comments: 0

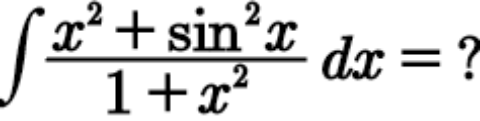
Question Number 109709 Answers: 2 Comments: 0

∫_(0 ) ^(π/4) ln(tanx+1)dx

$$\:\:\:\:\:\int_{\mathrm{0}\:} ^{\frac{\pi}{\mathrm{4}}} \mathrm{ln}\left(\mathrm{tanx}+\mathrm{1}\right)\mathrm{dx} \\ $$

Question Number 109658 Answers: 1 Comments: 0

Question Number 109848 Answers: 0 Comments: 0

Question Number 109642 Answers: 1 Comments: 0

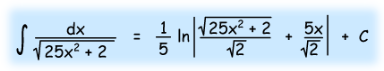
Question Number 109616 Answers: 1 Comments: 1

find ∫_0 ^1 (√(1+x^4 ))dx

$$\mathrm{find}\:\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{4}} }\mathrm{dx} \\ $$

Question Number 109546 Answers: 1 Comments: 2

If f(x) continue in [ 1,30] and ∫_6 ^(30) f(x)dx = 30, then ∫_1 ^9 f(3y+3)dy = __

$${If}\:{f}\left({x}\right)\:{continue}\:{in}\:\left[\:\mathrm{1},\mathrm{30}\right]\:{and}\: \\ $$$$\underset{\mathrm{6}} {\overset{\mathrm{30}} {\int}}{f}\left({x}\right){dx}\:=\:\mathrm{30},\:{then}\:\underset{\mathrm{1}} {\overset{\mathrm{9}} {\int}}{f}\left(\mathrm{3}{y}+\mathrm{3}\right){dy}\:=\:\_\_ \\ $$

Question Number 109506 Answers: 0 Comments: 0

Question Number 109509 Answers: 4 Comments: 0

((bemath)/(Σ_(i=cooll) ^(nice) (joss)_i )) ∫ ((x^2 dx)/( (√(x^2 +25))))

$$\:\:\frac{{bemath}}{\underset{{i}={cooll}} {\overset{{nice}} {\sum}}\left({joss}\right)_{{i}} }\: \\ $$$$ \\ $$$$\int\:\frac{{x}^{\mathrm{2}} \:{dx}}{\:\sqrt{{x}^{\mathrm{2}} +\mathrm{25}}} \\ $$

Question Number 109472 Answers: 4 Comments: 0

Question Number 109459 Answers: 0 Comments: 0

Question Number 109457 Answers: 3 Comments: 0

Question Number 109435 Answers: 1 Comments: 0

Question Number 109378 Answers: 4 Comments: 0

Question Number 109366 Answers: 0 Comments: 1

Question Number 109343 Answers: 2 Comments: 1

Question Number 109342 Answers: 0 Comments: 3

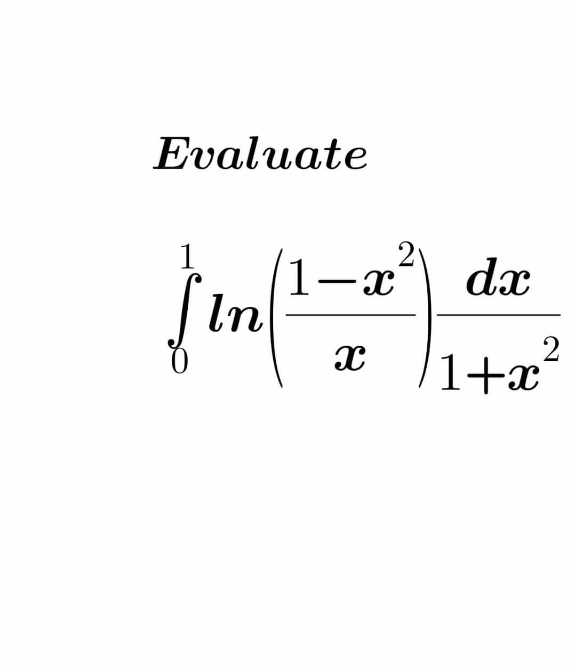
Question Number 109220 Answers: 0 Comments: 0

calculate I_n =∫_0 ^(2π) ((cos(nx))/(cosx +sinx))dx (n→natural)

$$\mathrm{calculate}\:\:\mathrm{I}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\frac{\mathrm{cos}\left(\mathrm{nx}\right)}{\mathrm{cosx}\:+\mathrm{sinx}}\mathrm{dx}\:\:\left(\mathrm{n}\rightarrow\mathrm{natural}\right) \\ $$

Question Number 109219 Answers: 0 Comments: 0

let f(x) =((sin(αx))/(sinx)) , 2π periodi even developp f at fourier serie

$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)\:=\frac{\mathrm{sin}\left(\alpha\mathrm{x}\right)}{\mathrm{sinx}}\:\:\:\:\:,\:\mathrm{2}\pi\:\mathrm{periodi}\:\mathrm{even} \\ $$$$\mathrm{developp}\:\mathrm{f}\:\mathrm{at}\:\mathrm{fourier}\:\mathrm{serie} \\ $$

Pg 145 Pg 146 Pg 147 Pg 148 Pg 149 Pg 150 Pg 151 Pg 152 Pg 153 Pg 154

Contact: info@tinkutara.com