
IntegrationQuestion and Answers: Page 149
Question Number 110749 Answers: 1 Comments: 0
Question Number 118674 Answers: 1 Comments: 0
Question Number 110551 Answers: 2 Comments: 0
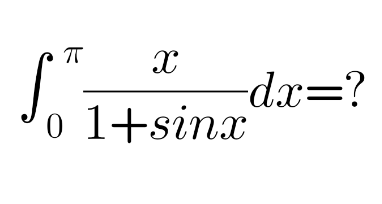
Question Number 110549 Answers: 2 Comments: 1

Question Number 110543 Answers: 1 Comments: 0

Question Number 110888 Answers: 3 Comments: 0
Question Number 110451 Answers: 1 Comments: 0
Question Number 110450 Answers: 1 Comments: 0
Question Number 110448 Answers: 1 Comments: 0
Question Number 110447 Answers: 1 Comments: 0
Question Number 110301 Answers: 0 Comments: 1

Question Number 110262 Answers: 0 Comments: 0
Question Number 110247 Answers: 1 Comments: 0
Question Number 110245 Answers: 1 Comments: 2
$${solve}\:\int\frac{{dx}}{\:\sqrt[{\mathrm{3}}]{{c}−\sqrt{{b}−{ax}}}} \\ $$
Question Number 110233 Answers: 0 Comments: 0

Question Number 110218 Answers: 1 Comments: 0

Question Number 110215 Answers: 1 Comments: 1
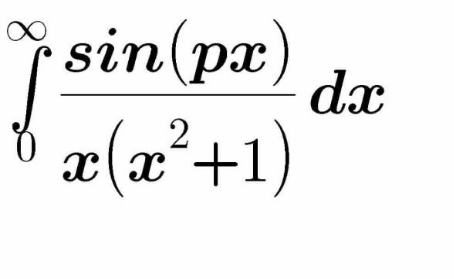
Question Number 110203 Answers: 1 Comments: 0

Question Number 110143 Answers: 2 Comments: 0
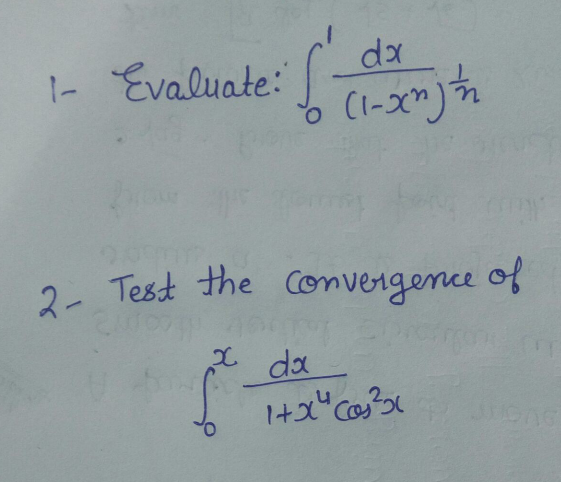
Question Number 110027 Answers: 2 Comments: 0

Question Number 109989 Answers: 1 Comments: 1

Question Number 109988 Answers: 0 Comments: 0
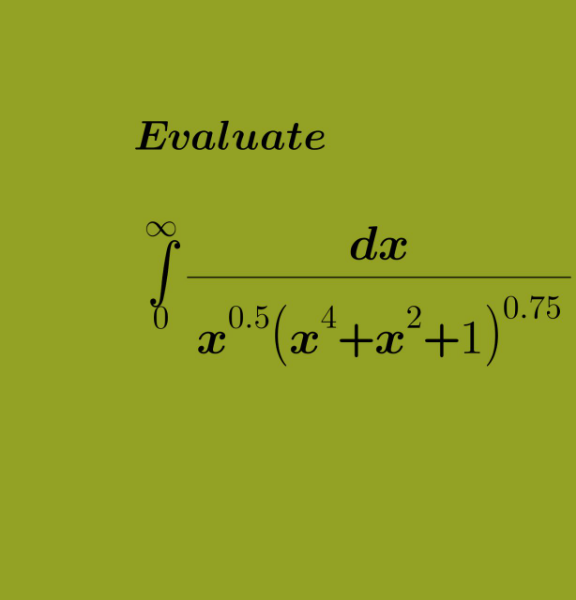
Question Number 109949 Answers: 1 Comments: 0

Question Number 109891 Answers: 1 Comments: 0
Question Number 109884 Answers: 1 Comments: 1

Question Number 109872 Answers: 2 Comments: 1
Pg 144 Pg 145 Pg 146 Pg 147 Pg 148 Pg 149 Pg 150 Pg 151 Pg 152 Pg 153
