
IntegrationQuestion and Answers: Page 132
Question Number 121886 Answers: 2 Comments: 1

Question Number 121875 Answers: 2 Comments: 0
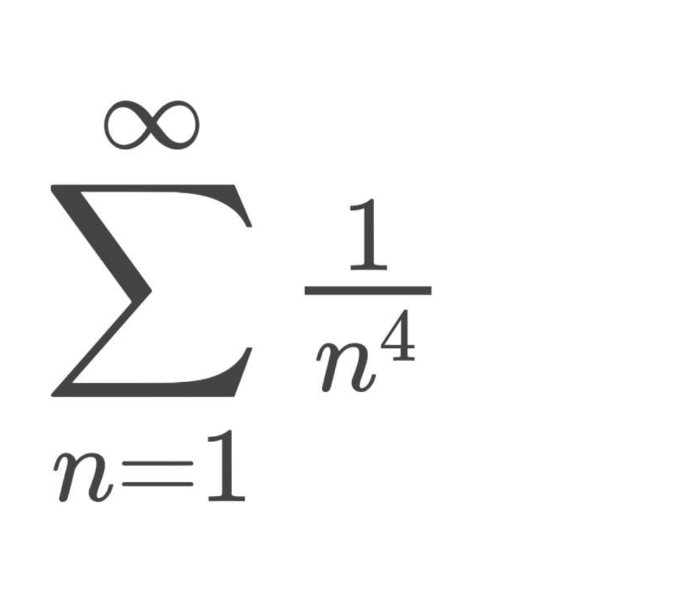
Question Number 121862 Answers: 1 Comments: 0
Question Number 121860 Answers: 0 Comments: 0
Question Number 121859 Answers: 2 Comments: 0
Question Number 121858 Answers: 0 Comments: 0
Question Number 121857 Answers: 0 Comments: 0
Question Number 121908 Answers: 0 Comments: 0

Question Number 121854 Answers: 0 Comments: 0

Question Number 121825 Answers: 1 Comments: 1
Question Number 121814 Answers: 0 Comments: 0
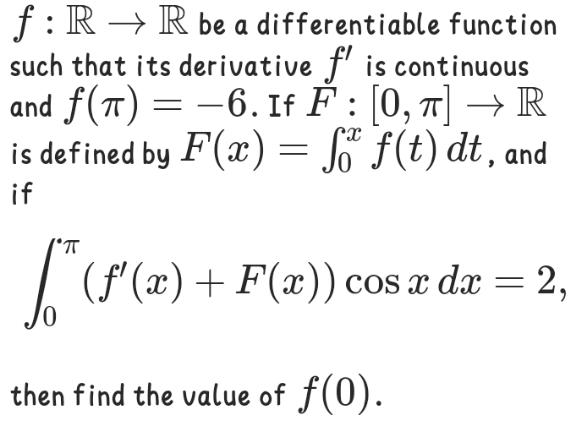
Question Number 121774 Answers: 0 Comments: 0
Question Number 121766 Answers: 0 Comments: 0
Question Number 121712 Answers: 1 Comments: 0
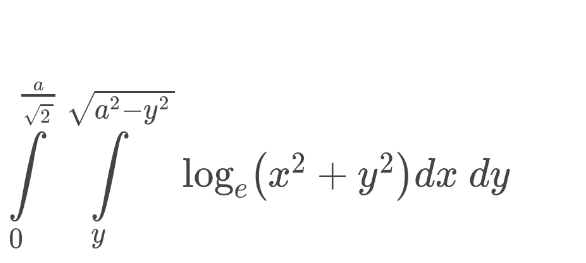
Question Number 121707 Answers: 1 Comments: 0
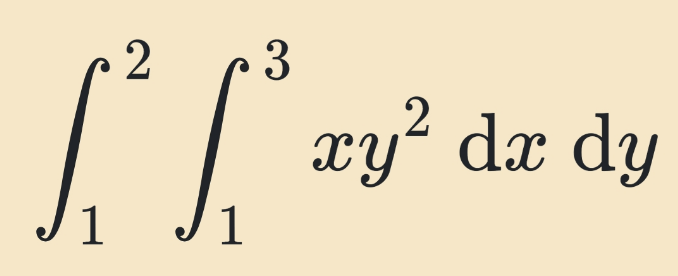
Question Number 121704 Answers: 1 Comments: 0
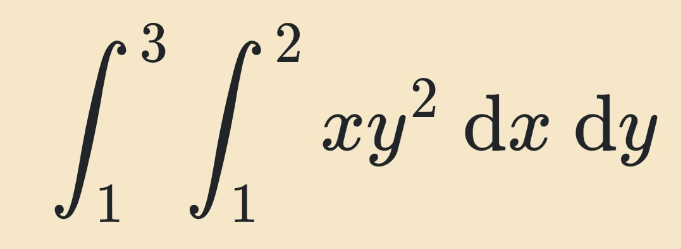
Question Number 121696 Answers: 2 Comments: 0
Question Number 121680 Answers: 1 Comments: 0
Question Number 121674 Answers: 3 Comments: 0
Question Number 121611 Answers: 1 Comments: 2
Question Number 121602 Answers: 2 Comments: 0

Question Number 121601 Answers: 4 Comments: 0
$$\:\:\int\:\frac{\mathrm{dx}}{\mathrm{x}\:\sqrt{\mathrm{3}+\mathrm{x}^{\mathrm{2}} }}\:? \\ $$
Question Number 121548 Answers: 1 Comments: 0
Question Number 121519 Answers: 1 Comments: 0
Question Number 121498 Answers: 1 Comments: 0
Question Number 121500 Answers: 3 Comments: 1
Pg 127 Pg 128 Pg 129 Pg 130 Pg 131 Pg 132 Pg 133 Pg 134 Pg 135 Pg 136
