Question and Answers Forum

IntegrationQuestion and Answers: Page 130

Question Number 122506 Answers: 1 Comments: 0

Question Number 122496 Answers: 2 Comments: 0

V=∫_0 ^3 ((x dx)/( (√(x+1))+(√(5x+1)))) T=∫_(−π/2) ^(π/2) (√(cos x−cos^3 x)) dx

$$\:\:{V}=\underset{\mathrm{0}} {\overset{\mathrm{3}} {\int}}\:\frac{{x}\:{dx}}{\:\sqrt{{x}+\mathrm{1}}+\sqrt{\mathrm{5}{x}+\mathrm{1}}} \\ $$$$\:{T}=\underset{−\pi/\mathrm{2}} {\overset{\pi/\mathrm{2}} {\int}}\sqrt{\mathrm{cos}\:{x}−\mathrm{cos}\:^{\mathrm{3}} {x}}\:{dx}\: \\ $$

Question Number 122469 Answers: 1 Comments: 5

Question Number 122461 Answers: 2 Comments: 2

∫ (x/((x^2 +a^2 )(x^3 +b^2 ))) ?

$$\:\int\:\frac{{x}}{\left({x}^{\mathrm{2}} +{a}^{\mathrm{2}} \right)\left({x}^{\mathrm{3}} +{b}^{\mathrm{2}} \right)}\:? \\ $$

Question Number 122432 Answers: 0 Comments: 0

Question Number 122430 Answers: 1 Comments: 0

∫ (x^3 /( (√(4−x^2 ))+x^2 −4)) dx

$$\:\int\:\frac{{x}^{\mathrm{3}} }{\:\sqrt{\mathrm{4}−{x}^{\mathrm{2}} }+{x}^{\mathrm{2}} −\mathrm{4}}\:{dx}\: \\ $$

Question Number 122397 Answers: 4 Comments: 0

a\∫((2x+1)/(1+x))(√((1−x)/(1+x)))dx c\∫(dx/( (√x)+(x)^(1/3) )) b\∫(dx/(x+(√(x−1)))) d\∫(dx/( (1+x)(√(1+x+x^2 ))))

$$\mathrm{a}\backslash\int\frac{\mathrm{2x}+\mathrm{1}}{\mathrm{1}+\mathrm{x}}\sqrt{\frac{\mathrm{1}−\mathrm{x}}{\mathrm{1}+\mathrm{x}}}\mathrm{dx}\:\:\:\:\:\:\:\:\mathrm{c}\backslash\int\frac{\mathrm{dx}}{\:\sqrt{\mathrm{x}}+\sqrt[{\mathrm{3}}]{\mathrm{x}}} \\ $$$$\mathrm{b}\backslash\int\frac{\mathrm{dx}}{\mathrm{x}+\sqrt{\mathrm{x}−\mathrm{1}}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{d}\backslash\int\frac{\mathrm{dx}}{\:\left(\mathrm{1}+\mathrm{x}\right)\sqrt{\mathrm{1}+\mathrm{x}+\mathrm{x}^{\mathrm{2}} }} \\ $$

Question Number 122366 Answers: 1 Comments: 0

Question Number 122349 Answers: 1 Comments: 1

Find the polynomial P(x) of least degree that has a maximum equal to 6 at x=1 and minimum equal to 2 at x=3.

$$\:\mathrm{Find}\:\mathrm{the}\:\mathrm{polynomial}\:\mathrm{P}\left(\mathrm{x}\right)\:\mathrm{of}\:\mathrm{least}\:\mathrm{degree} \\ $$$$\mathrm{that}\:\mathrm{has}\:\mathrm{a}\:\mathrm{maximum}\:\mathrm{equal}\:\mathrm{to}\:\mathrm{6}\:\mathrm{at}\:\mathrm{x}=\mathrm{1} \\ $$$$\mathrm{and}\:\mathrm{minimum}\:\mathrm{equal}\:\mathrm{to}\:\mathrm{2}\:\mathrm{at}\:\mathrm{x}=\mathrm{3}.\: \\ $$

Question Number 122330 Answers: 2 Comments: 0

...advanced calculus... prove that : Re(∫_0 ^( (π/2)) sin^3 (x)ln(ln(cos(x)))dx) =^? ((ln(3)−2γ)/3) ✓

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:...{advanced}\:\:{calculus}... \\ $$$$\:\:{prove}\:{that}\:: \\ $$$$\:\:\:\mathscr{R}{e}\left(\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} {sin}^{\mathrm{3}} \left({x}\right){ln}\left({ln}\left({cos}\left({x}\right)\right)\right){dx}\right) \\ $$$$\:\:\:\:\:\:\:\overset{?} {=}\frac{{ln}\left(\mathrm{3}\right)−\mathrm{2}\gamma}{\mathrm{3}}\:\checkmark \\ $$

Question Number 122323 Answers: 1 Comments: 1

calculate A_n =∫_0 ^∞ (dx/((x^2 +1)(x^2 +2)....(x^2 +n))) wth n integr natural and n≥1

$$\mathrm{calculate}\:\:\mathrm{A}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{dx}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} +\mathrm{2}\right)....\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{n}\right)} \\ $$$$\mathrm{wth}\:\mathrm{n}\:\mathrm{integr}\:\mathrm{natural}\:\mathrm{and}\:\mathrm{n}\geqslant\mathrm{1} \\ $$

Question Number 122298 Answers: 1 Comments: 0

...nice calculus... prove that : Σ_(n=1 ) ^∞ {((ζ(2n+1)−1)/(n+1))}=−γ+ln(2)✓ ..m.n.1970..

$$\:\:\:\:\:...{nice}\:\:{calculus}... \\ $$$$\:\:{prove}\:\:{that}\::\:\: \\ $$$$\:\:\:\:\:\:\:\underset{{n}=\mathrm{1}\:} {\overset{\infty} {\sum}}\left\{\frac{\zeta\left(\mathrm{2}{n}+\mathrm{1}\right)−\mathrm{1}}{{n}+\mathrm{1}}\right\}=−\gamma+{ln}\left(\mathrm{2}\right)\checkmark \\ $$$$\:\:\:..{m}.{n}.\mathrm{1970}.. \\ $$

Question Number 122290 Answers: 1 Comments: 1

... nice calculus... calculate :: Ω=∫_0 ^( 1) x^2 (ψ(1+x)−ψ(2−x))dx=??? .m.n.1970.

$$\:\:\:\:\:...\:\:{nice}\:\:{calculus}... \\ $$$$\:\:\:{calculate}\::: \\ $$$$\:\:\:\Omega=\int_{\mathrm{0}} ^{\:\mathrm{1}} {x}^{\mathrm{2}} \left(\psi\left(\mathrm{1}+{x}\right)−\psi\left(\mathrm{2}−{x}\right)\right){dx}=??? \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:.{m}.{n}.\mathrm{1970}. \\ $$

Question Number 122274 Answers: 2 Comments: 0

∫ ((√(1−(√x)))/( (√(1+(√x))))) dx ?

$$\:\:\:\int\:\frac{\sqrt{\mathrm{1}−\sqrt{{x}}}}{\:\sqrt{\mathrm{1}+\sqrt{{x}}}}\:{dx}\:?\: \\ $$

Question Number 122205 Answers: 1 Comments: 2

find ∫ (dx/(x(x+1)(√(x^2 +x))))

$$\mathrm{find}\:\int\:\:\frac{\mathrm{dx}}{\mathrm{x}\left(\mathrm{x}+\mathrm{1}\right)\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}}} \\ $$

Question Number 122186 Answers: 2 Comments: 0

Obtain a reduction formulae for I_n = ∫_0 ^1 (ln x)^n dx find I_2 = ∫_0 ^1 (ln x)^2 dx

$$\mathrm{Obtain}\:\mathrm{a}\:\mathrm{reduction}\:\mathrm{formulae}\:\mathrm{for}\: \\ $$$$\:\:\:{I}_{{n}} \:=\:\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\left(\mathrm{ln}\:{x}\right)^{{n}} {dx}\: \\ $$$$\mathrm{find}\:{I}_{\mathrm{2}} =\:\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\left(\mathrm{ln}\:{x}\right)^{\mathrm{2}} \:{dx} \\ $$

Question Number 122160 Answers: 1 Comments: 0

Question Number 122159 Answers: 3 Comments: 0

... nice calculus... prove that:: Ω=∫_0 ^( (π/2)) {tan^(−1) (ptan(x))−tan^(−1) (qtan(x))}(tan(x)+cot(x))dx =(π/2) log((p/q)) ( p , q >0 ) m.n.

$$\:\:\:\:\:...\:{nice}\:\:{calculus}... \\ $$$$\:\:\:{prove}\:\:{that}:: \\ $$$$\Omega=\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \left\{{tan}^{−\mathrm{1}} \left({ptan}\left({x}\right)\right)−{tan}^{−\mathrm{1}} \left({qtan}\left({x}\right)\right)\right\}\left({tan}\left({x}\right)+{cot}\left({x}\right)\right){dx} \\ $$$$=\frac{\pi}{\mathrm{2}}\:{log}\left(\frac{{p}}{{q}}\right)\:\:\:\left(\:\:\:\:{p}\:,\:{q}\:>\mathrm{0}\:\:\:\right) \\ $$$$\:\:\:\:{m}.{n}. \\ $$

Question Number 122157 Answers: 2 Comments: 1

find ∫_(−1) ^1 (√(1+x^4 ))dx

$$\mathrm{find}\:\int_{−\mathrm{1}} ^{\mathrm{1}} \sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{4}} }\mathrm{dx} \\ $$

Question Number 122137 Answers: 1 Comments: 0

Question Number 122120 Answers: 2 Comments: 0

∫_0 ^3 (1/( (√y))).e^y dy

$$\underset{\mathrm{0}} {\overset{\mathrm{3}} {\int}}\frac{\mathrm{1}}{\:\sqrt{{y}}}.{e}^{{y}} {dy} \\ $$

Question Number 122109 Answers: 2 Comments: 0

Question Number 122108 Answers: 1 Comments: 0

∫_1 ^2 ((ln (x))/x^2 ) dx ?

$$\:\:\:\underset{\mathrm{1}} {\overset{\mathrm{2}} {\int}}\:\frac{\mathrm{ln}\:\left({x}\right)}{{x}^{\mathrm{2}} }\:{dx}\:? \\ $$

Question Number 122098 Answers: 1 Comments: 1

Question Number 122044 Answers: 0 Comments: 3

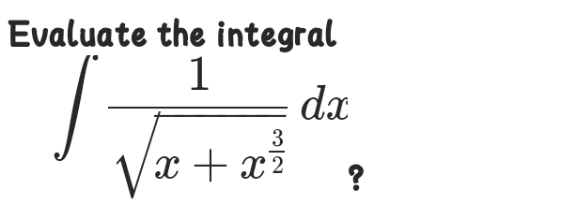
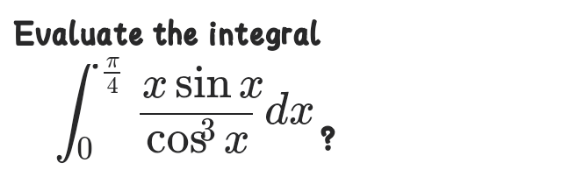
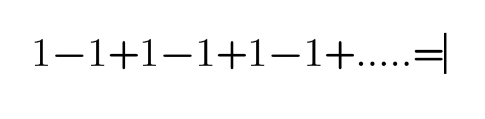
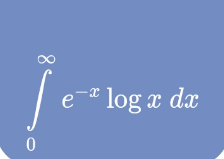
Question Number 122035 Answers: 1 Comments: 0

...nice calculus... prove that : ∫_0 ^( 1) ((ln^2 (1+x))/x)dx=^(??) ((ζ(3))/4) .m.n.

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:...{nice}\:{calculus}... \\ $$$$\:\:\:\:\:\:\:\:{prove}\:\:{that}\:: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{{ln}^{\mathrm{2}} \left(\mathrm{1}+{x}\right)}{{x}}{dx}\overset{??} {=}\frac{\zeta\left(\mathrm{3}\right)}{\mathrm{4}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:.{m}.{n}. \\ $$

Pg 125 Pg 126 Pg 127 Pg 128 Pg 129 Pg 130 Pg 131 Pg 132 Pg 133 Pg 134

Contact: info@tinkutara.com