
IntegrationQuestion and Answers: Page 128
Question Number 123703 Answers: 1 Comments: 0
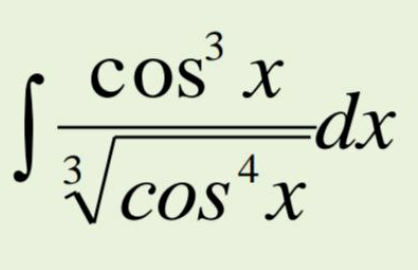
Question Number 123676 Answers: 2 Comments: 0
Question Number 123674 Answers: 2 Comments: 0
Question Number 123673 Answers: 0 Comments: 0
Question Number 123687 Answers: 1 Comments: 0
Question Number 123639 Answers: 1 Comments: 0
Question Number 123552 Answers: 4 Comments: 0
Question Number 123550 Answers: 3 Comments: 0
Question Number 123513 Answers: 3 Comments: 1
Question Number 123495 Answers: 1 Comments: 0
Question Number 123494 Answers: 2 Comments: 0
Question Number 123462 Answers: 1 Comments: 0
Question Number 123456 Answers: 0 Comments: 0
Question Number 123452 Answers: 4 Comments: 1
$$\int\frac{{dx}}{\:\left({x}^{\mathrm{2}} +{n}\right)\sqrt{{x}^{\mathrm{2}} +{a}}} \\ $$
Question Number 123454 Answers: 1 Comments: 0
Question Number 123526 Answers: 3 Comments: 2
Question Number 123387 Answers: 0 Comments: 2
Question Number 123386 Answers: 1 Comments: 0
Question Number 123361 Answers: 0 Comments: 1
$${please}\:{find}\:{the}\:\int\frac{{e}^{{x}} }{{x}}{dx} \\ $$
Question Number 123352 Answers: 0 Comments: 0
Question Number 123331 Answers: 1 Comments: 0
Question Number 123261 Answers: 1 Comments: 0
Question Number 123255 Answers: 1 Comments: 0
Question Number 123253 Answers: 0 Comments: 0
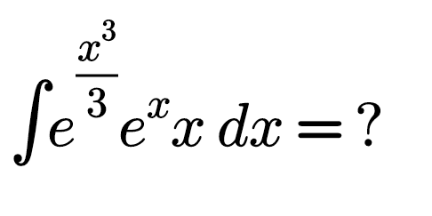
Question Number 123234 Answers: 3 Comments: 1
$$\:\:\int\:\sqrt{{x}^{\mathrm{2}} −\mathrm{4}{x}+\mathrm{5}}\:{dx}\: \\ $$
Question Number 123177 Answers: 0 Comments: 0
Pg 123 Pg 124 Pg 125 Pg 126 Pg 127 Pg 128 Pg 129 Pg 130 Pg 131 Pg 132
