Question and Answers Forum

All Questions Topic List

IntegrationQuestion and Answers: Page 114

Question Number 129763 Answers: 2 Comments: 0

prove ∫_(−∞) ^(+∞) (1/(1+e^x^2 ))dx=(√π) (1−(√2) )ξ((1/2))

$${prove} \\ $$$$\int_{−\infty} ^{+\infty} \frac{\mathrm{1}}{\mathrm{1}+{e}^{{x}^{\mathrm{2}} } }{dx}=\sqrt{\pi}\:\left(\mathrm{1}−\sqrt{\mathrm{2}}\:\right)\xi\left(\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$

Question Number 129696 Answers: 1 Comments: 5

nice old question by sir m?th+et?s ∫_0 ^∞ cos((x^3 /3)+tx)dx

$${nice}\:{old}\:{question}\:{by}\:{sir}\:{m}?{th}+{et}?{s}\: \\ $$$$\int_{\mathrm{0}} ^{\infty} {cos}\left(\frac{{x}^{\mathrm{3}} }{\mathrm{3}}+{tx}\right){dx} \\ $$$$ \\ $$

Question Number 129794 Answers: 3 Comments: 0

∫ (x^2 −1)(x+1)^(−2/3) dx ?

$$\:\:\int\:\left(\mathrm{x}^{\mathrm{2}} −\mathrm{1}\right)\left(\mathrm{x}+\mathrm{1}\right)^{−\mathrm{2}/\mathrm{3}} \:\mathrm{dx}\:? \\ $$

Question Number 129688 Answers: 1 Comments: 0

complex analysis ∮_C ((ϱ^(2z) +sinz^2 )/((z−2)(z−3)))dz C:∣Z∣=5

$${complex}\:{analysis} \\ $$$$\oint_{{C}} \frac{\varrho^{\mathrm{2}{z}} +{sinz}^{\mathrm{2}} }{\left({z}−\mathrm{2}\right)\left({z}−\mathrm{3}\right)}{dz}\:\:\:{C}:\mid{Z}\mid=\mathrm{5} \\ $$

Question Number 129684 Answers: 3 Comments: 0

... advanced calculus... prove that: ∫_0 ^( π) cos(tan(x)−cot(x))dx=(π/e^2 )

$$\:\:\:\:\:\:\:\:\:\:\:\:\:...\:{advanced}\:\:{calculus}... \\ $$$$\:\:{prove}\:\:{that}: \\ $$$$\:\:\int_{\mathrm{0}} ^{\:\pi} {cos}\left({tan}\left({x}\right)−{cot}\left({x}\right)\right){dx}=\frac{\pi}{{e}^{\mathrm{2}} } \\ $$$$ \\ $$

Question Number 129651 Answers: 0 Comments: 0

... nice calculus... evaluate :: Σ_(n=1) ^∞ (((−1)^n )/(n^2 +1)) (((sin(n))/n))^2 =?

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:...\:{nice}\:\:\:{calculus}... \\ $$$$\:\:\:\:{evaluate}\::: \\ $$$$\:\:\:\:\:\:\:\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}^{\mathrm{2}} +\mathrm{1}}\:\left(\frac{{sin}\left({n}\right)}{{n}}\right)^{\mathrm{2}} =? \\ $$$$ \\ $$

Question Number 129645 Answers: 1 Comments: 0

ϝ = ∫ (dx/(1+x^(12) )) .

$$\:\:\:\:\digamma\:=\:\int\:\frac{{dx}}{\mathrm{1}+{x}^{\mathrm{12}} }\:. \\ $$

Question Number 129646 Answers: 3 Comments: 0

ϝ = ∫ (dx/(x^(2n+1) (x^n −1)))

$$\:\:\digamma\:=\:\int\:\frac{{dx}}{{x}^{\mathrm{2}{n}+\mathrm{1}} \left({x}^{{n}} −\mathrm{1}\right)} \\ $$

Question Number 129635 Answers: 3 Comments: 1

∫(dx/((x^2 +1)^2 ))=? Σ_(k=1) ^n (1/(k(k+1)(2k+1)))=?

$$\:\:\:\:\: \\ $$$$ \\ $$$$\:\:\:\:\:\:\int\frac{\boldsymbol{{dx}}}{\left(\boldsymbol{{x}}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }=? \\ $$$$\underset{\boldsymbol{{k}}=\mathrm{1}} {\overset{\boldsymbol{{n}}} {\sum}}\frac{\mathrm{1}}{\boldsymbol{{k}}\left(\boldsymbol{{k}}+\mathrm{1}\right)\left(\mathrm{2}\boldsymbol{{k}}+\mathrm{1}\right)}=? \\ $$

Question Number 129576 Answers: 1 Comments: 0

please, how to show that f : [0 , a] × R_+ → R (x, y) e^(−xy) sin x is integrable ???

$$\boldsymbol{\mathrm{please}},\:\boldsymbol{\mathrm{how}}\:\boldsymbol{\mathrm{to}}\:\boldsymbol{\mathrm{show}}\:\boldsymbol{\mathrm{that}}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{{f}}\::\:\left[\mathrm{0}\:,\:\boldsymbol{{a}}\right]\:×\:\mathbb{R}_{+} \:\rightarrow\:\mathbb{R} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\boldsymbol{{x}},\:\boldsymbol{{y}}\right)\:\:\:\:\:\:\: \:\:\boldsymbol{{e}}^{−\boldsymbol{{xy}}} \:\boldsymbol{{sin}}\:\boldsymbol{{x}}\: \\ $$$$\boldsymbol{\mathrm{is}}\:\boldsymbol{\mathrm{integrable}}\:???\: \\ $$

Question Number 129564 Answers: 2 Comments: 0

V = ∫ ((sin x)/(sin (x+θ))) dx

$$\:\mathcal{V}\:=\:\int\:\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{sin}\:\left(\mathrm{x}+\theta\right)}\:\mathrm{dx}\: \\ $$

Question Number 129562 Answers: 1 Comments: 0

calculate ∫_0 ^∞ (dx/((x^6 +1)^2 ))

$$\mathrm{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{dx}}{\left(\mathrm{x}^{\mathrm{6}} +\mathrm{1}\right)^{\mathrm{2}} } \\ $$

Question Number 129558 Answers: 1 Comments: 0

...modern ∗∗∗∗∗∗∗∗∗∗ algebra ... ::: if ′′ G ′′ be a finite group and O (G)=pq , where ′′ p , q ′′ are two prime numbers (p > q ) then prove that: G has at most one subgroup of order ′′ p ′′ . written and compiled by ...♣m.n.july.1970♣....

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:...{modern}\:\ast\ast\ast\ast\ast\ast\ast\ast\ast\ast\:{algebra}\:...\: \\ $$$$\:\:\:\:\:\:\:\::::\:\:{if}\:\:''\:{G}\:''\:{be}\:{a}\:{finite}\:{group}\:{and} \\ $$$$\:\:{O}\:\left({G}\right)={pq}\:\:,\:\:{where}\:''\:{p}\:,\:{q}\:''\:{are}\:{two} \\ $$$$\:\:{prime}\:\:{numbers}\:\left({p}\:>\:{q}\:\right)\:{then}\:{prove}\:{that}: \\ $$$$\:\:{G}\:\:{has}\:\:{at}\:{most}\:{one}\:{subgroup}\:{of}\:{order}\:''\:{p}\:''\:. \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{written}\:{and}\:{compiled}\:{by} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:...\clubsuit{m}.{n}.{july}.\mathrm{1970}\clubsuit.... \\ $$

Question Number 129594 Answers: 0 Comments: 1

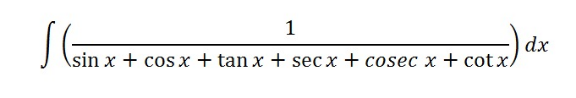
Question Number 129519 Answers: 1 Comments: 0

∫ cos (y^3 )dy

$$\int\:{cos}\:\left({y}^{\mathrm{3}} \right){dy} \\ $$

Question Number 129503 Answers: 0 Comments: 2

Question Number 129418 Answers: 3 Comments: 0

...advsnced calculus.... calculate: Ω=∫_0 ^( ∞) e^(−(√x) ) ln(1+(1/( (√x) )))dx

$$\:\:\:\:\:\:\:\:\:\:\:\:...{advsnced}\:\:\:\:\:\:{calculus}....\:\: \\ $$$$ \\ $$$$\:\:\:{calculate}:\:\Omega=\int_{\mathrm{0}} ^{\:\infty} {e}^{−\sqrt{{x}}\:} {ln}\left(\mathrm{1}+\frac{\mathrm{1}}{\:\sqrt{{x}}\:}\right){dx} \\ $$$$ \\ $$

Question Number 129377 Answers: 2 Comments: 0

∫(( (√x))/( (√(x-1))))dx = ...

$$\int\frac{\:\sqrt{\boldsymbol{\mathrm{x}}}}{\:\sqrt{\boldsymbol{\mathrm{x}}-\mathrm{1}}}\mathrm{dx}\:=\:... \\ $$

Question Number 129370 Answers: 1 Comments: 0

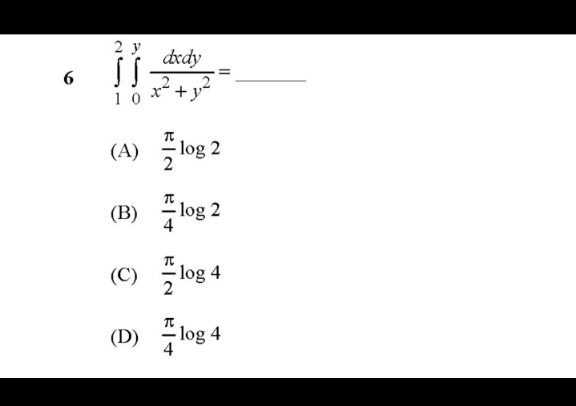
Question Number 129318 Answers: 1 Comments: 0

... laplace transformation.. L (te^(−t) ⌊t⌋)=? note : ⌊x⌋ is floor of ′′ x ′′... ..............

$$\:\:\:\:\:...\:\:{laplace}\:\:\:\:\:{transformation}.. \\ $$$$\:\:\:\:\mathscr{L}\:\:\left({te}^{−{t}} \lfloor{t}\rfloor\right)=? \\ $$$$\:\:\:{note}\::\:\lfloor{x}\rfloor\:{is}\:{floor}\:{of}\:''\:{x}\:''... \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:.............. \\ $$$$ \\ $$

Question Number 129277 Answers: 0 Comments: 0

O = ∫_( 0) ^( 1) ((arctan (((3X+3)/(1−2X−X^2 ))))/(1+X^2 )) dX

$$\:\mathrm{O}\:=\:\int_{\:\mathrm{0}} ^{\:\mathrm{1}} \:\frac{\mathrm{arctan}\:\left(\frac{\mathrm{3X}+\mathrm{3}}{\mathrm{1}−\mathrm{2X}−\mathrm{X}^{\mathrm{2}} }\right)}{\mathrm{1}+\mathrm{X}^{\mathrm{2}} }\:\mathrm{dX} \\ $$

Question Number 129275 Answers: 0 Comments: 2

∫e^x^x dx??????

$$\int\mathrm{e}^{\mathrm{x}^{\mathrm{x}} } \mathrm{dx}?????? \\ $$

Question Number 129274 Answers: 1 Comments: 0

∫ ((cos^2 x+cos x)/(1+sin x+cos x)) dx ?

$$\:\int\:\frac{\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}+\mathrm{cos}\:\mathrm{x}}{\mathrm{1}+\mathrm{sin}\:\mathrm{x}+\mathrm{cos}\:\mathrm{x}}\:\mathrm{dx}\:? \\ $$

Question Number 129273 Answers: 0 Comments: 0

please answer my question

$$\mathrm{please}\:\mathrm{answer}\:\mathrm{my}\:\mathrm{question} \\ $$

Question Number 129269 Answers: 1 Comments: 0

∫_1 ^a ((4(√x) +k)/( (√x) +1)) = 4a+3 . Find the value of ∫_1 ^( a) (1/( (√x) +1)) dx .

$$\:\int_{\mathrm{1}} ^{{a}} \:\frac{\mathrm{4}\sqrt{{x}}\:+{k}}{\:\sqrt{{x}}\:+\mathrm{1}}\:=\:\mathrm{4}{a}+\mathrm{3}\:.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{value} \\ $$$$\mathrm{of}\:\int_{\mathrm{1}} ^{\:{a}} \frac{\mathrm{1}}{\:\sqrt{{x}}\:+\mathrm{1}}\:{dx}\:. \\ $$

Question Number 129251 Answers: 3 Comments: 1

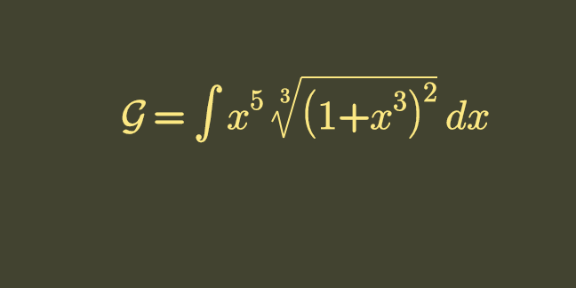
Pg 109 Pg 110 Pg 111 Pg 112 Pg 113 Pg 114 Pg 115 Pg 116 Pg 117 Pg 118

Terms of Service

Contact: info@tinkutara.com