Question and Answers Forum

IntegrationQuestion and Answers: Page 104

Question Number 134182 Answers: 1 Comments: 0

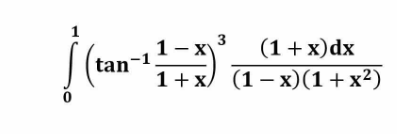
Question Number 134058 Answers: 1 Comments: 0

J = ∫ (dx/(1+tan x+csc x+cot x+sec x))

$$\mathcal{J}\:=\:\int\:\frac{{dx}}{\mathrm{1}+\mathrm{tan}\:{x}+\mathrm{csc}\:{x}+\mathrm{cot}\:{x}+\mathrm{sec}\:{x}} \\ $$

Question Number 134039 Answers: 0 Comments: 0

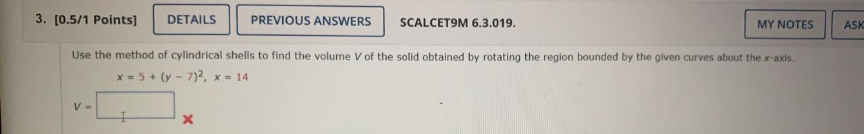
Question Number 134038 Answers: 1 Comments: 0

Question Number 134016 Answers: 1 Comments: 0

?prove :Σ_(n=1) ^∞ (((−1)^(n−1) H_(2n) )/(2n+1))=(π/8)ln(2)..

$$ \\ $$$$\:\:\:\:\:\:?{prove}\::\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} {H}_{\mathrm{2}{n}} }{\mathrm{2}{n}+\mathrm{1}}=\frac{\pi}{\mathrm{8}}{ln}\left(\mathrm{2}\right).. \\ $$

Question Number 133973 Answers: 1 Comments: 0

Given { ((f(x)=((x+(√(x^2 +(1/(27))))))^(1/3) +((x−(√(x^2 +(1/(27))))))^(1/3) )),((g(x)=x^3 +x+1)) :} Find ∫_0 ^4 (g○f○g)(x) dx .

$$\:\mathrm{Given}\:\begin{cases}{\mathrm{f}\left(\mathrm{x}\right)=\sqrt[{\mathrm{3}}]{\mathrm{x}+\sqrt{\mathrm{x}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{27}}}}+\sqrt[{\mathrm{3}}]{\mathrm{x}−\sqrt{\mathrm{x}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{27}}}}}\\{\mathrm{g}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{3}} +\mathrm{x}+\mathrm{1}}\end{cases} \\ $$$$\mathrm{Find}\:\underset{\mathrm{0}} {\overset{\mathrm{4}} {\int}}\:\left(\mathrm{g}\circ\mathrm{f}\circ\mathrm{g}\right)\left(\mathrm{x}\right)\:\mathrm{dx}\:. \\ $$

Question Number 133972 Answers: 1 Comments: 0

H = ∫ (((2x−1)^7 )/((2x+1)^9 )) dx

$$\mathscr{H}\:=\:\int\:\frac{\left(\mathrm{2x}−\mathrm{1}\right)^{\mathrm{7}} }{\left(\mathrm{2x}+\mathrm{1}\right)^{\mathrm{9}} }\:\mathrm{dx}\: \\ $$

Question Number 133963 Answers: 1 Comments: 0

Y = ∫ (dx/( ((1+x^6 ))^(1/6) ))?

$$\mathcal{Y}\:=\:\int\:\frac{{dx}}{\:\sqrt[{\mathrm{6}}]{\mathrm{1}+{x}^{\mathrm{6}} }}? \\ $$

Question Number 133957 Answers: 2 Comments: 0

1)decompose F(x)=(1/((x+1)^5 (2x−1)^4 )) 2) find ∫_1 ^∞ F(x)dx

$$\left.\mathrm{1}\right)\mathrm{decompose}\:\:\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\left(\mathrm{x}+\mathrm{1}\right)^{\mathrm{5}} \left(\mathrm{2x}−\mathrm{1}\right)^{\mathrm{4}} } \\ $$$$\left.\mathrm{2}\right)\:\mathrm{find}\:\int_{\mathrm{1}} ^{\infty} \:\mathrm{F}\left(\mathrm{x}\right)\mathrm{dx} \\ $$

Question Number 133943 Answers: 0 Comments: 0

.......advanced calculus...... prove that: 𝛗= ∫_0 ^( ∞) ((cos(x^2 )−cos(x))/x)dx=(γ/2) γ: euler−mascheroni constant...

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:.......{advanced}\:\:\:\:\:{calculus}...... \\ $$$$\:\:\:\:\:\:{prove}\:\:{that}: \\ $$$$\:\:\:\:\:\:\boldsymbol{\phi}=\:\:\int_{\mathrm{0}} ^{\:\infty} \frac{{cos}\left({x}^{\mathrm{2}} \right)−{cos}\left({x}\right)}{{x}}{dx}=\frac{\gamma}{\mathrm{2}} \\ $$$$\:\:\:\gamma:\:{euler}−{mascheroni}\:{constant}... \\ $$

Question Number 133911 Answers: 1 Comments: 0

A=∫ ((cos x)/(sin 5x+sin x)) dx ?

$$\mathcal{A}=\int\:\frac{\mathrm{cos}\:\mathrm{x}}{\mathrm{sin}\:\mathrm{5x}+\mathrm{sin}\:\mathrm{x}}\:\mathrm{dx}\:? \\ $$

Question Number 133910 Answers: 0 Comments: 0

De^ montrer que; Σ_(n=1) ^∞ (((−1)^n )/(1+n^4 ))=(1/2)[(π/( (√2))) ((sin((π/( (√2))))cosh((π/( (√2))))+sinh((π/( (√2))))cos((π/( (√2)))))/(sinh^2 ((π/( (√2))))+sin^2 ((π/( (√2))))))]

$$\:\:\:\:\mathcal{D}\acute {\mathrm{e}montrer}\:\mathrm{que}; \\ $$$$\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{1}+\mathrm{n}^{\mathrm{4}} }=\frac{\mathrm{1}}{\mathrm{2}}\left[\frac{\pi}{\:\sqrt{\mathrm{2}}}\:\frac{\mathrm{sin}\left(\frac{\pi}{\:\sqrt{\mathrm{2}}}\right)\mathrm{cosh}\left(\frac{\pi}{\:\sqrt{\mathrm{2}}}\right)+\mathrm{sinh}\left(\frac{\pi}{\:\sqrt{\mathrm{2}}}\right)\mathrm{cos}\left(\frac{\pi}{\:\sqrt{\mathrm{2}}}\right)}{\mathrm{sinh}^{\mathrm{2}} \left(\frac{\pi}{\:\sqrt{\mathrm{2}}}\right)+\mathrm{sin}^{\mathrm{2}} \left(\frac{\pi}{\:\sqrt{\mathrm{2}}}\right)}\right] \\ $$

Question Number 133907 Answers: 0 Comments: 0

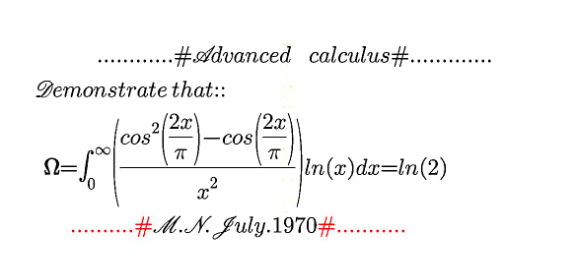
Question Number 133938 Answers: 1 Comments: 0

Question Number 133825 Answers: 1 Comments: 0

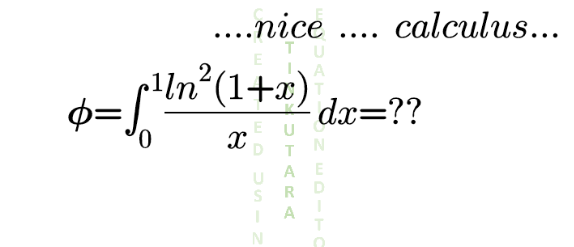
Question Number 133791 Answers: 2 Comments: 0

......advanced integral.... prove that :: 𝛗=∫_0 ^( ∞) (((1−e^(−ϕx) )/(1+e^(ϕx) )) )(dx/x) =?? ϕ: = Golden ratio...

$$\:\:\:\:\:\:\:\:\:\:\:\:......{advanced}\:\:\:\:{integral}.... \\ $$$$\:\:\:{prove}\:\:{that}\::: \\ $$$$\:\:\:\:\:\:\:\:\:\boldsymbol{\phi}=\int_{\mathrm{0}} ^{\:\infty} \left(\frac{\mathrm{1}−{e}^{−\varphi{x}} }{\mathrm{1}+{e}^{\varphi{x}} }\:\right)\frac{{dx}}{{x}}\:=?? \\ $$$$\:\:\:\varphi:\:=\:{Golden}\:{ratio}... \\ $$$$ \\ $$

Question Number 133786 Answers: 1 Comments: 0

A = ∫_0 ^( 1) sin^(−1) (((x^2 +1)/( (√(2x^4 +2)))) ) dx =?

$$\mathcal{A}\:=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \mathrm{sin}^{−\mathrm{1}} \left(\frac{{x}^{\mathrm{2}} +\mathrm{1}}{\:\sqrt{\mathrm{2}{x}^{\mathrm{4}} +\mathrm{2}}}\:\right)\:{dx}\:=? \\ $$

Question Number 133951 Answers: 3 Comments: 0

V = ∫ ln (x+(√(1+x^2 )) ) dx

$$\:\mathcal{V}\:=\:\int\:\mathrm{ln}\:\left(\mathrm{x}+\sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\:\right)\:\mathrm{dx}\: \\ $$

Question Number 133719 Answers: 1 Comments: 1

Question Number 133725 Answers: 0 Comments: 7

Question Number 133674 Answers: 2 Comments: 0

∫ (√(1+(√(1+(√x))))) dx =?

$$\int\:\sqrt{\mathrm{1}+\sqrt{\mathrm{1}+\sqrt{\mathrm{x}}}}\:\mathrm{dx}\:=?\: \\ $$

Question Number 133591 Answers: 6 Comments: 0

∫_0 ^1 x^2 (√(1−x^2 )) dx ?

$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\mathrm{x}^{\mathrm{2}} \:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\:\mathrm{dx}\:?\: \\ $$

Question Number 133563 Answers: 1 Comments: 0

∫_0 ^∞ [sin (1/x)−(1/π)sin ((π/x))] dx

$$\:\underset{\mathrm{0}} {\overset{\infty} {\int}}\:\left[\mathrm{sin}\:\frac{\mathrm{1}}{\mathrm{x}}−\frac{\mathrm{1}}{\pi}\mathrm{sin}\:\left(\frac{\pi}{\mathrm{x}}\right)\right]\:\mathrm{dx} \\ $$

Question Number 133541 Answers: 1 Comments: 2

∫_0 ^3 (√(9−x^2 )) dx =? (a)13.5 (b)21 (c)22.5 (d)1.8 (e) 30

$$\underset{\mathrm{0}} {\overset{\mathrm{3}} {\int}}\sqrt{\mathrm{9}−\mathrm{x}^{\mathrm{2}} }\:\mathrm{dx}\:=? \\ $$$$\left(\mathrm{a}\right)\mathrm{13}.\mathrm{5}\:\:\:\:\:\:\left(\mathrm{b}\right)\mathrm{21}\:\:\:\:\:\:\left(\mathrm{c}\right)\mathrm{22}.\mathrm{5} \\ $$$$\left(\mathrm{d}\right)\mathrm{1}.\mathrm{8}\:\:\:\:\:\:\:\:\:\left(\mathrm{e}\right)\:\mathrm{30} \\ $$

Question Number 133540 Answers: 0 Comments: 0

∫ (cosec x)^(11456) (cot x)^(11456) dx ?

$$\int\:\left(\mathrm{cosec}\:\mathrm{x}\right)^{\mathrm{11456}} \:\left(\mathrm{cot}\:\mathrm{x}\right)^{\mathrm{11456}} \:\mathrm{dx}\:? \\ $$

Question Number 133538 Answers: 0 Comments: 0

calculate ∫∫_([1,2]^2 ) (√(x^2 +3y^2 ))e^(−(x^2 +3y^2 )) dxdy

$$\mathrm{calculate}\:\int\int_{\left[\mathrm{1},\mathrm{2}\right]^{\mathrm{2}} } \:\:\:\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{3y}^{\mathrm{2}} }\mathrm{e}^{−\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{3y}^{\mathrm{2}} \right)} \mathrm{dxdy} \\ $$

Pg 99 Pg 100 Pg 101 Pg 102 Pg 103 Pg 104 Pg 105 Pg 106 Pg 107 Pg 108

Contact: info@tinkutara.com