
Previous in Permutation and Combination Next in Permutation and Combination
Question Number 158529 by mr W last updated on 11/Nov/21

$${In}\:{how}\:{many}\:{ways}\:{can}\:\mathrm{30}\:{students} \\ $$$${be}\:{distributed}\:{to}\:\mathrm{10}\:{schools},\:{if} \\ $$$$\mathrm{1}.\:{each}\:{school}\:{should}\:{get}\:{at}\:{least}\:{one} \\ $$$$\:\:\:\:\:{student}. \\ $$$$\mathrm{2}.\:{no}\:{restriction} \\ $$
Answered by cortano last updated on 06/Nov/21
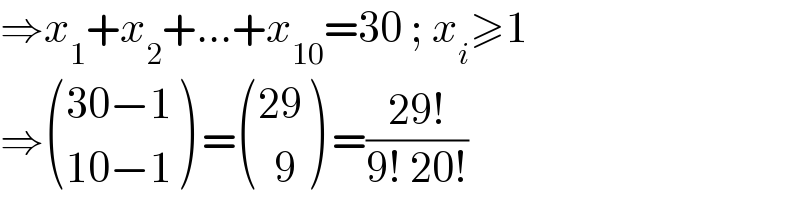
$$\Rightarrow{x}_{\mathrm{1}} +{x}_{\mathrm{2}} +...+{x}_{\mathrm{10}} =\mathrm{30}\:;\:{x}_{{i}} \geqslant\mathrm{1} \\ $$$$\Rightarrow\begin{pmatrix}{\mathrm{30}−\mathrm{1}}\\{\mathrm{10}−\mathrm{1}}\end{pmatrix}\:=\begin{pmatrix}{\mathrm{29}}\\{\:\:\mathrm{9}}\end{pmatrix}\:=\frac{\mathrm{29}!}{\mathrm{9}!\:\mathrm{20}!} \\ $$
Commented by mr W last updated on 07/Nov/21

$${this}\:{is}\:{only}\:{true},\:{when}\:\mathrm{30}\:\left({identical}\right) \\ $$$${computers}\:{are}\:{distributed}\:{to}\:\mathrm{10} \\ $$$${schools}.\:{but}\:{here}\:{the}\:\mathrm{30}\:{students} \\ $$$${are}\:{different}\:{objects},\:{so}\:{the}\:{answer} \\ $$$${is}\:{not}\:{correct}. \\ $$
Commented by ajfour last updated on 06/Nov/21
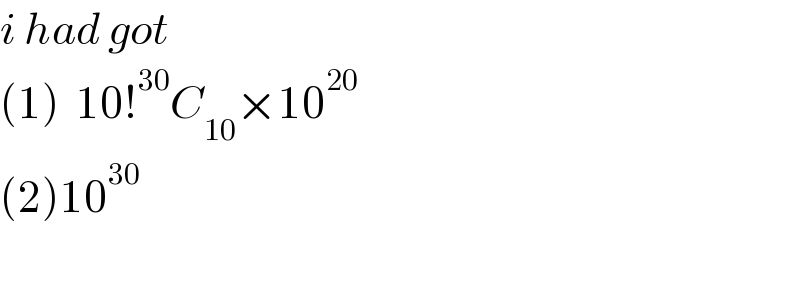
$${i}\:{had}\:{got}\:\: \\ $$$$\left(\mathrm{1}\right)\:\:\mathrm{10}!^{\mathrm{30}} {C}_{\mathrm{10}} ×\mathrm{10}^{\mathrm{20}} \\ $$$$\left(\mathrm{2}\right)\mathrm{10}^{\mathrm{30}} \\ $$$$ \\ $$
Commented by mr W last updated on 06/Nov/21

$${the}\:{result}\:{for}\:\left(\mathrm{1}\right)\:{seems}\:{to}\:{be}\:{logic}, \\ $$$${but}\:{it}\:{is}\:{in}\:{fact}\:{not}\:{correct},\:{because} \\ $$$${it}\:{is}\:{larger}\:{than}\:{the}\:{answer}\:{to}\:\left(\mathrm{2}\right), \\ $$$${and}\:{this}\:{can}\:{not}\:{be}\:{true},\:{since} \\ $$$${the}\:{ways}\:{with}\:{restriction}\:{must}\:{be}\: \\ $$$${less}\:{than}\:{the}\:{ways}\:{without}\:{restriction}. \\ $$$${so}\:{where}\:{is}\:{the}\:{error}? \\ $$
Commented by mr W last updated on 11/Nov/21

$${correct}\:{solution}\:{see}\:{Q}\mathrm{158955} \\ $$
