
Question Number 97483 by Rio Michael last updated on 08/Jun/20

$$\mathrm{In}\:\mathrm{each}\:\mathrm{week}\:\mathrm{the}\:\mathrm{growth}\:\mathrm{of}\:\mathrm{a}\:\mathrm{plant}\:\mathrm{is}\:\mathrm{two}−\mathrm{thirds} \\ $$$$\mathrm{the}\:\mathrm{growth}\:\mathrm{of}\:\mathrm{the}\:\mathrm{previous}\:\mathrm{week}. \\ $$$$\mathrm{The}\:\mathrm{plant}\:\mathrm{grows}\:\mathrm{12}\:\mathrm{cm}\:\mathrm{in}\:\mathrm{the}\:\mathrm{first}\:\mathrm{week}. \\ $$$$\left(\mathrm{a}\right)\:\mathrm{Calculate}\:\mathrm{the}\:\mathrm{growth}\:\mathrm{of}\:\mathrm{the}\:\mathrm{plant}\:\mathrm{in}\: \\ $$$$\left(\mathrm{b}\right)\:\mathrm{the}\:\mathrm{limiting}\:\mathrm{height}\:\mathrm{of}\:\mathrm{the}\:\mathrm{pant} \\ $$
Commented by bobhans last updated on 08/Jun/20
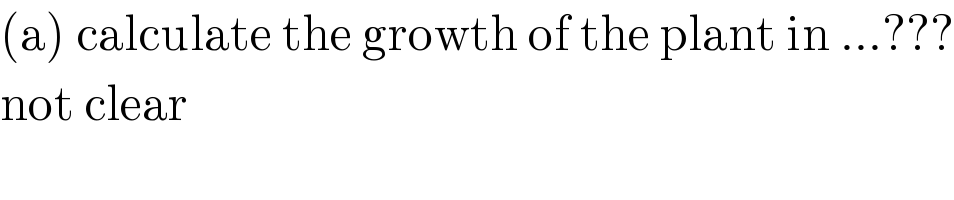
$$\left(\mathrm{a}\right)\:\mathrm{calculate}\:\mathrm{the}\:\mathrm{growth}\:\mathrm{of}\:\mathrm{the}\:\mathrm{plant}\:\mathrm{in}\:...??? \\ $$$$\mathrm{not}\:\mathrm{clear} \\ $$
Commented by Rio Michael last updated on 08/Jun/20
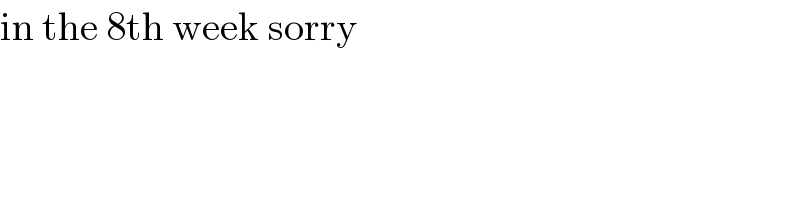
$$\mathrm{in}\:\mathrm{the}\:\mathrm{8th}\:\mathrm{week}\:\mathrm{sorry} \\ $$
Commented by bobhans last updated on 08/Jun/20
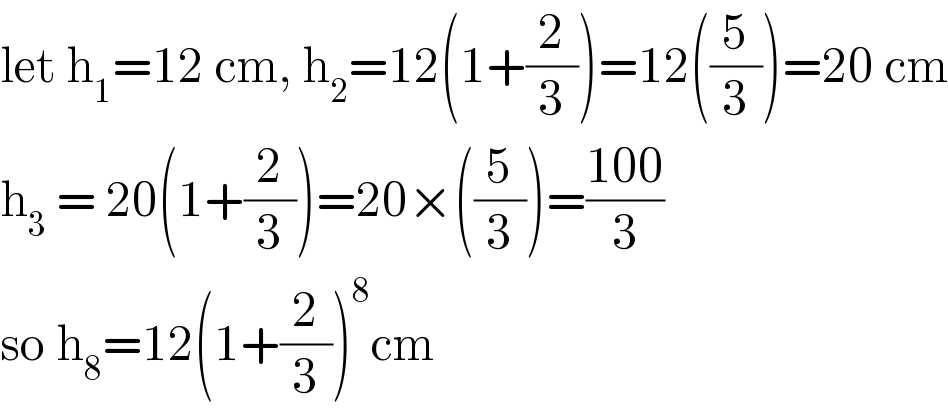
$$\mathrm{let}\:\mathrm{h}_{\mathrm{1}} =\mathrm{12}\:\mathrm{cm},\:\mathrm{h}_{\mathrm{2}} =\mathrm{12}\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}\right)=\mathrm{12}\left(\frac{\mathrm{5}}{\mathrm{3}}\right)=\mathrm{20}\:\mathrm{cm} \\ $$$$\mathrm{h}_{\mathrm{3}} \:=\:\mathrm{20}\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}\right)=\mathrm{20}×\left(\frac{\mathrm{5}}{\mathrm{3}}\right)=\frac{\mathrm{100}}{\mathrm{3}} \\ $$$$\mathrm{so}\:\mathrm{h}_{\mathrm{8}} =\mathrm{12}\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{8}} \mathrm{cm} \\ $$
Commented by mr W last updated on 08/Jun/20
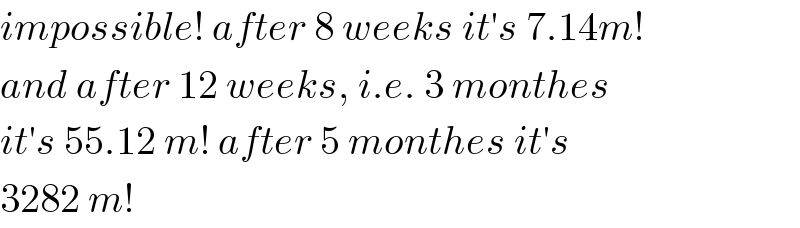
$${impossible}!\:{after}\:\mathrm{8}\:{weeks}\:{it}'{s}\:\mathrm{7}.\mathrm{14}{m}! \\ $$$${and}\:{after}\:\mathrm{12}\:{weeks},\:{i}.{e}.\:\mathrm{3}\:{monthes} \\ $$$${it}'{s}\:\mathrm{55}.\mathrm{12}\:{m}!\:{after}\:\mathrm{5}\:{monthes}\:{it}'{s} \\ $$$$\mathrm{3282}\:{m}! \\ $$
Answered by mr W last updated on 09/Jun/20
![week 1: growth 12, height 12 week 2: growth 12×(2/3), height 12(1+(2/3)) week 3: growth 12×((2/3))^2 , height 12[1+(2/3)+((2/3))^2 ] ... week n: growth 12×((2/3))^(n−1) , height 12[1+(2/3)+((2/3))^2 +..+((2/3))^(n−1) ] height after n weeks: h_n =12[1+(2/3)+((2/3))^2 +..+((2/3))^(n−1) ]=((12[1−((2/3))^n ])/(1−(2/3))) ⇒h_n =36[1−((2/3))^n ] h_8 =36[1−((2/3))^8 ]=34.6 cm h_(max) =lim_(n→∞) h_n =36 cm](Q97580.png)
$${week}\:\mathrm{1}:\:{growth}\:\mathrm{12},\:{height}\:\mathrm{12} \\ $$$${week}\:\mathrm{2}:\:{growth}\:\mathrm{12}×\frac{\mathrm{2}}{\mathrm{3}},\:{height}\:\mathrm{12}\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}\right) \\ $$$${week}\:\mathrm{3}:\:{growth}\:\mathrm{12}×\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{2}} ,\:{height}\:\mathrm{12}\left[\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}+\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{2}} \right] \\ $$$$... \\ $$$${week}\:{n}:\:{growth}\:\mathrm{12}×\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{{n}−\mathrm{1}} ,\:{height}\:\mathrm{12}\left[\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}+\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{2}} +..+\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{{n}−\mathrm{1}} \right] \\ $$$${height}\:{after}\:{n}\:{weeks}:\:\:{h}_{{n}} =\mathrm{12}\left[\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}+\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{2}} +..+\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{{n}−\mathrm{1}} \right]=\frac{\mathrm{12}\left[\mathrm{1}−\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{{n}} \right]}{\mathrm{1}−\frac{\mathrm{2}}{\mathrm{3}}} \\ $$$$\Rightarrow{h}_{{n}} =\mathrm{36}\left[\mathrm{1}−\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{{n}} \right] \\ $$$${h}_{\mathrm{8}} =\mathrm{36}\left[\mathrm{1}−\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{8}} \right]=\mathrm{34}.\mathrm{6}\:{cm} \\ $$$$ \\ $$$${h}_{{max}} =\underset{{n}\rightarrow\infty} {\mathrm{lim}}{h}_{{n}} =\mathrm{36}\:{cm} \\ $$
