
Question Number 182530 by HeferH last updated on 10/Dec/22
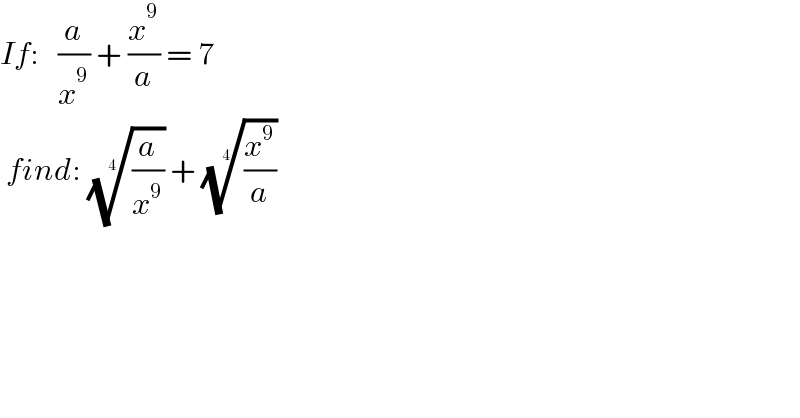
$${If}:\:\:\:\frac{{a}}{{x}^{\mathrm{9}} }\:+\:\frac{{x}^{\mathrm{9}} }{{a}}\:=\:\mathrm{7} \\ $$$$\:{find}:\:\sqrt[{\mathrm{4}}]{\frac{{a}}{{x}^{\mathrm{9}} }}\:+\:\sqrt[{\mathrm{4}}]{\frac{{x}^{\mathrm{9}} }{{a}}} \\ $$
Answered by mr W last updated on 10/Dec/22
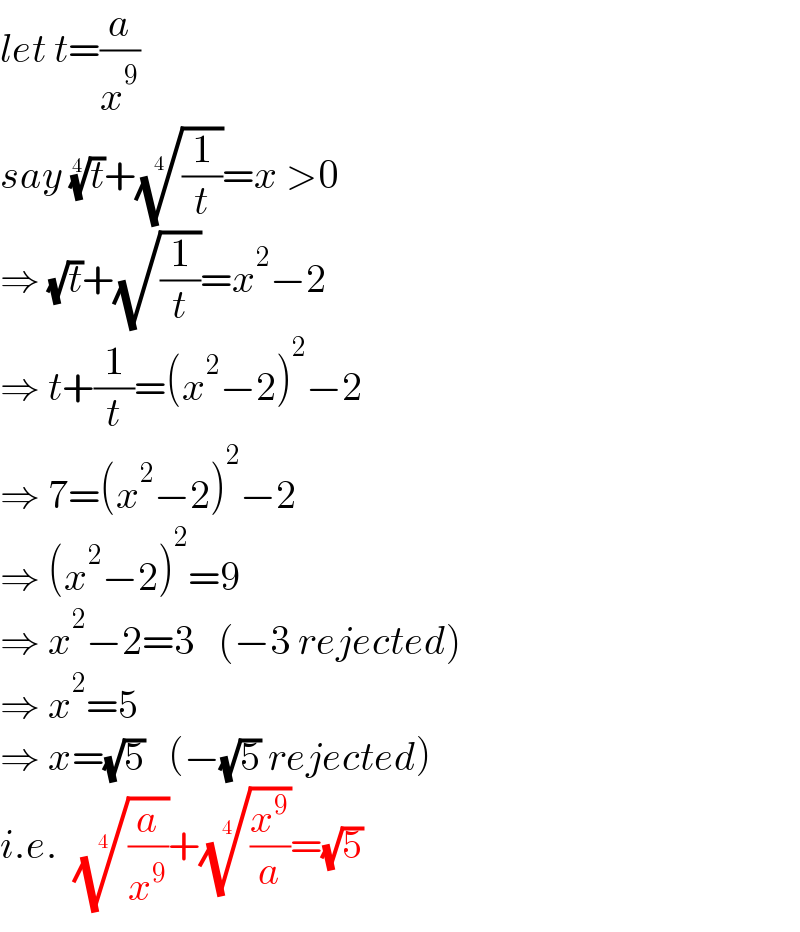
$${let}\:{t}=\frac{{a}}{{x}^{\mathrm{9}} } \\ $$$${say}\:\sqrt[{\mathrm{4}}]{{t}}+\sqrt[{\mathrm{4}}]{\frac{\mathrm{1}}{{t}}}={x}\:>\mathrm{0} \\ $$$$\Rightarrow\:\sqrt{{t}}+\sqrt{\frac{\mathrm{1}}{{t}}}={x}^{\mathrm{2}} −\mathrm{2} \\ $$$$\Rightarrow\:{t}+\frac{\mathrm{1}}{{t}}=\left({x}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{2}} −\mathrm{2} \\ $$$$\Rightarrow\:\mathrm{7}=\left({x}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{2}} −\mathrm{2} \\ $$$$\Rightarrow\:\left({x}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{2}} =\mathrm{9} \\ $$$$\Rightarrow\:{x}^{\mathrm{2}} −\mathrm{2}=\mathrm{3}\:\:\:\left(−\mathrm{3}\:{rejected}\right) \\ $$$$\Rightarrow\:{x}^{\mathrm{2}} =\mathrm{5} \\ $$$$\Rightarrow\:{x}=\sqrt{\mathrm{5}}\:\:\:\left(−\sqrt{\mathrm{5}}\:{rejected}\right) \\ $$$${i}.{e}.\:\:\sqrt[{\mathrm{4}}]{\frac{{a}}{{x}^{\mathrm{9}} }}+\sqrt[{\mathrm{4}}]{\frac{{x}^{\mathrm{9}} }{{a}}}=\sqrt{\mathrm{5}} \\ $$
Answered by Frix last updated on 10/Dec/22
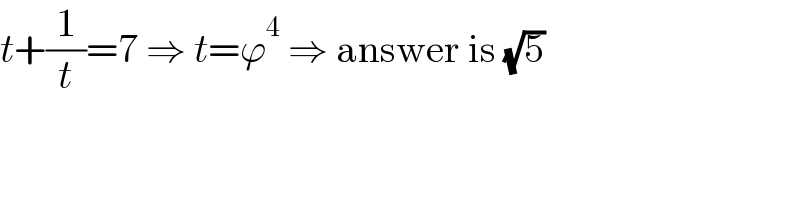
$${t}+\frac{\mathrm{1}}{{t}}=\mathrm{7}\:\Rightarrow\:{t}=\varphi^{\mathrm{4}} \:\Rightarrow\:\mathrm{answer}\:\mathrm{is}\:\sqrt{\mathrm{5}} \\ $$
Commented by manxsol last updated on 11/Dec/22
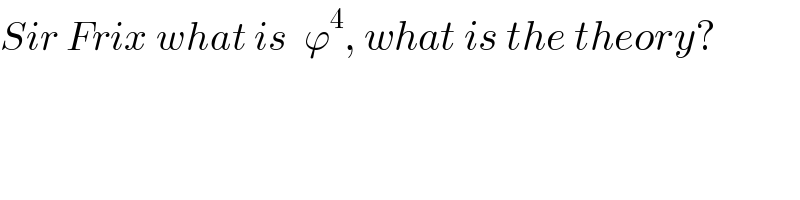
$${Sir}\:{Frix}\:{what}\:{is}\:\:\varphi^{\mathrm{4}} ,\:{what}\:{is}\:{the}\:{theory}? \\ $$
Commented by Frix last updated on 11/Dec/22
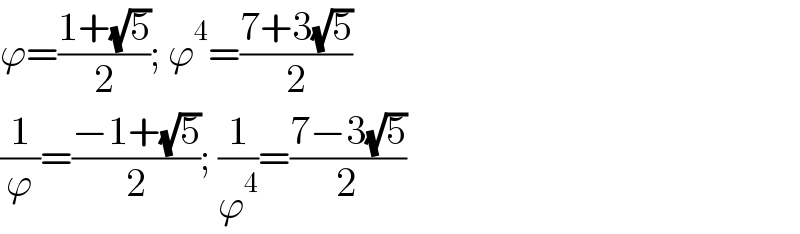
$$\varphi=\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}};\:\varphi^{\mathrm{4}} =\frac{\mathrm{7}+\mathrm{3}\sqrt{\mathrm{5}}}{\mathrm{2}} \\ $$$$\frac{\mathrm{1}}{\varphi}=\frac{−\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}};\:\frac{\mathrm{1}}{\varphi^{\mathrm{4}} }=\frac{\mathrm{7}−\mathrm{3}\sqrt{\mathrm{5}}}{\mathrm{2}} \\ $$
Commented by manxsol last updated on 12/Dec/22
$${thanks},{Sir}\:{Frix}\:{is}\:{golden}\:{number}.\:{check}\:{my}\:{next}\:{question} \\ $$
Answered by Ar Brandon last updated on 11/Dec/22
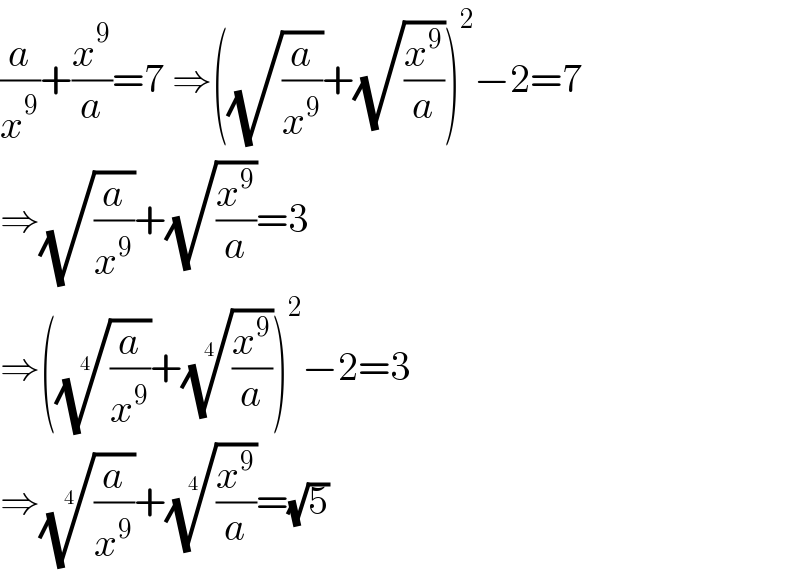
$$\frac{{a}}{{x}^{\mathrm{9}} }+\frac{{x}^{\mathrm{9}} }{{a}}=\mathrm{7}\:\Rightarrow\left(\sqrt{\frac{{a}}{{x}^{\mathrm{9}} }}+\sqrt{\frac{{x}^{\mathrm{9}} }{{a}}}\right)^{\mathrm{2}} −\mathrm{2}=\mathrm{7} \\ $$$$\Rightarrow\sqrt{\frac{{a}}{{x}^{\mathrm{9}} }}+\sqrt{\frac{{x}^{\mathrm{9}} }{{a}}}=\mathrm{3} \\ $$$$\Rightarrow\left(\sqrt[{\mathrm{4}}]{\frac{{a}}{{x}^{\mathrm{9}} }}+\sqrt[{\mathrm{4}}]{\frac{{x}^{\mathrm{9}} }{{a}}}\right)^{\mathrm{2}} −\mathrm{2}=\mathrm{3} \\ $$$$\Rightarrow\sqrt[{\mathrm{4}}]{\frac{{a}}{{x}^{\mathrm{9}} }}+\sqrt[{\mathrm{4}}]{\frac{{x}^{\mathrm{9}} }{{a}}}=\sqrt{\mathrm{5}} \\ $$
