
Question Number 204545 by hardmath last updated on 21/Feb/24
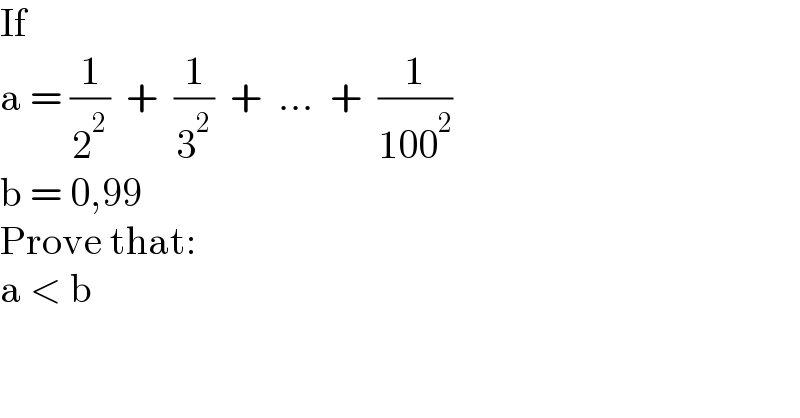
$$\mathrm{If} \\ $$$$\mathrm{a}\:=\:\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }\:\:+\:\:\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }\:\:+\:\:...\:\:+\:\:\frac{\mathrm{1}}{\mathrm{100}^{\mathrm{2}} } \\ $$$$\mathrm{b}\:=\:\mathrm{0},\mathrm{99} \\ $$$$\mathrm{Prove}\:\mathrm{that}: \\ $$$$\mathrm{a}\:<\:\mathrm{b} \\ $$
Answered by mr W last updated on 21/Feb/24
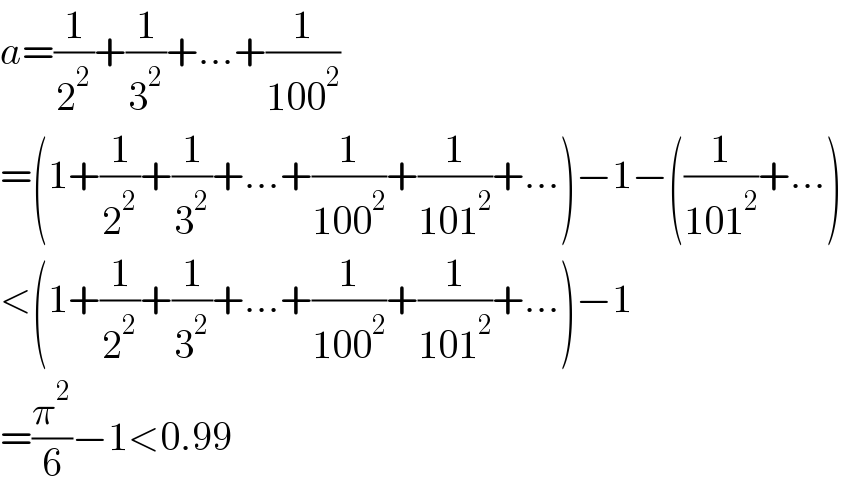
$${a}=\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }+...+\frac{\mathrm{1}}{\mathrm{100}^{\mathrm{2}} } \\ $$$$=\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }+...+\frac{\mathrm{1}}{\mathrm{100}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{101}^{\mathrm{2}} }+...\right)−\mathrm{1}−\left(\frac{\mathrm{1}}{\mathrm{101}^{\mathrm{2}} }+...\right) \\ $$$$<\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }+...+\frac{\mathrm{1}}{\mathrm{100}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{101}^{\mathrm{2}} }+...\right)−\mathrm{1} \\ $$$$=\frac{\pi^{\mathrm{2}} }{\mathrm{6}}−\mathrm{1}<\mathrm{0}.\mathrm{99} \\ $$
Answered by witcher3 last updated on 21/Feb/24
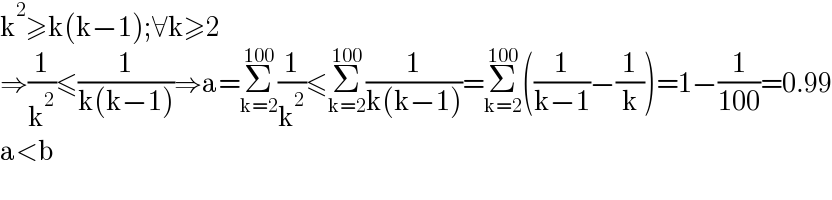
$$\mathrm{k}^{\mathrm{2}} \geqslant\mathrm{k}\left(\mathrm{k}−\mathrm{1}\right);\forall\mathrm{k}\geqslant\mathrm{2} \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{k}^{\mathrm{2}} }\leqslant\frac{\mathrm{1}}{\mathrm{k}\left(\mathrm{k}−\mathrm{1}\right)}\Rightarrow\mathrm{a}=\underset{\mathrm{k}=\mathrm{2}} {\overset{\mathrm{100}} {\sum}}\frac{\mathrm{1}}{\mathrm{k}^{\mathrm{2}} }\leqslant\underset{\mathrm{k}=\mathrm{2}} {\overset{\mathrm{100}} {\sum}}\frac{\mathrm{1}}{\mathrm{k}\left(\mathrm{k}−\mathrm{1}\right)}=\underset{\mathrm{k}=\mathrm{2}} {\overset{\mathrm{100}} {\sum}}\left(\frac{\mathrm{1}}{\mathrm{k}−\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{k}}\right)=\mathrm{1}−\frac{\mathrm{1}}{\mathrm{100}}=\mathrm{0}.\mathrm{99} \\ $$$$\mathrm{a}<\mathrm{b} \\ $$
Commented by mr W last updated on 21/Feb/24
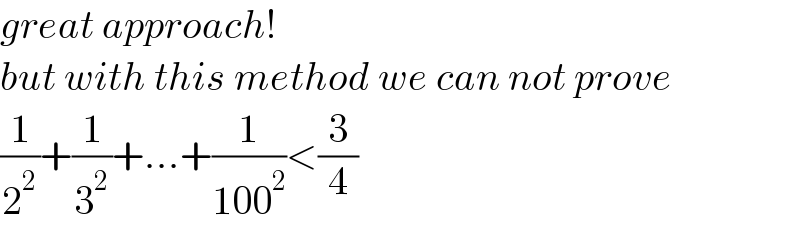
$${great}\:{approach}! \\ $$$${but}\:{with}\:{this}\:{method}\:{we}\:{can}\:{not}\:{prove} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }+...+\frac{\mathrm{1}}{\mathrm{100}^{\mathrm{2}} }<\frac{\mathrm{3}}{\mathrm{4}} \\ $$
Commented by witcher3 last updated on 22/Feb/24
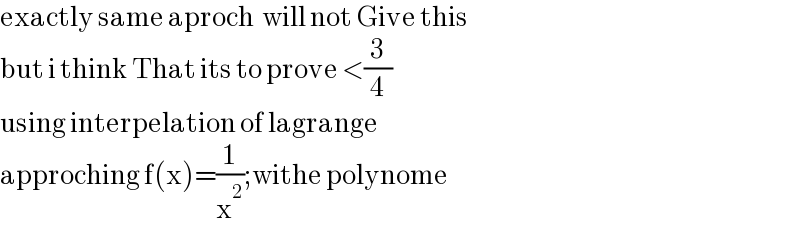
$$\mathrm{exactly}\:\mathrm{same}\:\mathrm{aproch}\:\:\mathrm{will}\:\mathrm{not}\:\mathrm{Give}\:\mathrm{this} \\ $$$$\mathrm{but}\:\mathrm{i}\:\mathrm{think}\:\mathrm{That}\:\mathrm{its}\:\mathrm{to}\:\mathrm{prove}\:<\frac{\mathrm{3}}{\mathrm{4}} \\ $$$$\mathrm{using}\:\mathrm{interpelation}\:\mathrm{of}\:\mathrm{lagrange} \\ $$$$\mathrm{approching}\:\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} };\mathrm{withe}\:\mathrm{polynome} \\ $$
Commented by mr W last updated on 22/Feb/24

$${thanks}\:{alot}! \\ $$
Commented by hardmath last updated on 23/Feb/24
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{dear}\:\mathrm{professors} \\ $$
