
Question Number 25344 by Rasheed.Sindhi last updated on 08/Dec/17

$$\mathrm{If}\:\mathrm{A}\:\mathrm{and}\:\mathrm{B}\:\mathrm{are}\:\mathrm{two}\:\mathrm{points}\:\mathrm{on}\:\mathrm{a} \\ $$$$\mathrm{circle}\:\mathrm{of}\:\mathrm{radius}\:\mathrm{r},\:\mathrm{then}\:\mathrm{prove}\:\mathrm{that} \\ $$$$\mathrm{m}\overline {\mathrm{AB}}\leqslant\mathrm{2r}. \\ $$
Answered by jota+ last updated on 08/Dec/17
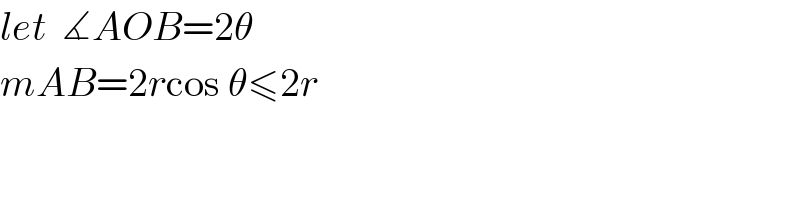
$${let}\:\:\measuredangle{AOB}=\mathrm{2}\theta \\ $$$${mAB}=\mathrm{2}{r}\mathrm{cos}\:\theta\leqslant\mathrm{2}{r} \\ $$
Commented by Rasheed.Sindhi last updated on 08/Dec/17

$$\mathcal{T}{h}^{\alpha} {nk}\:{U}! \\ $$
