Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 203545 by Mastermind last updated on 21/Jan/24

If A,B,C are finite sets whose elements are from the same universal set U and n(A) denotes the number of element in the set A (a) Show by means of venn diagram that n(A ∪ B) = n(A) + n(B) − n(A ∩ B) (b) Using the fact that (A ∪ B ∪ C) = (A∪B)∪C =A∪(B∪C) deduce an expression for (A∪B∪C) (c) If n(A∪B)= n(A∩B), what can be said about A and B? How did you reach your conclusion. Thank you in advance

$$\mathrm{If}\:\mathrm{A},\mathrm{B},\mathrm{C}\:\mathrm{are}\:\mathrm{finite}\:\mathrm{sets}\:\mathrm{whose}\:\mathrm{elements}\:\mathrm{are}\: \\ $$$$\mathrm{from}\:\mathrm{the}\:\mathrm{same}\:\mathrm{universal}\:\mathrm{set}\:\mathrm{U}\:\mathrm{and}\:\mathrm{n}\left(\mathrm{A}\right)\: \\ $$$$\mathrm{denotes}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{element}\:\mathrm{in}\:\mathrm{the}\:\mathrm{set}\:\mathrm{A} \\ $$$$\left(\mathrm{a}\right)\:\mathrm{Show}\:\mathrm{by}\:\mathrm{means}\:\mathrm{of}\:\mathrm{venn}\:\mathrm{diagram}\:\mathrm{that} \\ $$$$\mathrm{n}\left(\mathrm{A}\:\cup\:\mathrm{B}\right)\:=\:\mathrm{n}\left(\mathrm{A}\right)\:+\:\mathrm{n}\left(\mathrm{B}\right)\:−\:\mathrm{n}\left(\mathrm{A}\:\cap\:\mathrm{B}\right) \\ $$$$\left(\mathrm{b}\right)\:\mathrm{Using}\:\mathrm{the}\:\mathrm{fact}\:\mathrm{that}\:\left(\mathrm{A}\:\cup\:\mathrm{B}\:\cup\:\mathrm{C}\right)\:=\:\left(\mathrm{A}\cup\mathrm{B}\right)\cup\mathrm{C} \\ $$$$=\mathrm{A}\cup\left(\mathrm{B}\cup\mathrm{C}\right)\:\mathrm{deduce}\:\mathrm{an}\:\mathrm{expression}\:\mathrm{for} \\ $$$$\left(\mathrm{A}\cup\mathrm{B}\cup\mathrm{C}\right) \\ $$$$\left(\mathrm{c}\right)\:\mathrm{If}\:\mathrm{n}\left(\mathrm{A}\cup\mathrm{B}\right)=\:\mathrm{n}\left(\mathrm{A}\cap\mathrm{B}\right),\:\:\mathrm{what}\:\mathrm{can}\:\mathrm{be}\:\mathrm{said} \\ $$$$\mathrm{about}\:\mathrm{A}\:\mathrm{and}\:\mathrm{B}?\:\mathrm{How}\:\mathrm{did}\:\mathrm{you}\:\mathrm{reach}\:\mathrm{your}\:\mathrm{conclusion}. \\ $$$$ \\ $$$$ \\ $$$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{in}\:\mathrm{advance} \\ $$

Answered by deleteduser1 last updated on 21/Jan/24

b) n∣(A∪B)∪C∣=n[P∪C] where P=A∪B n(P∪C)=n(P)+n(C)−n(P∩C) =n(A)+n(B)+n(C)−n(A∩B)−n(P∩C) P∩C=(A∩B)∩C=(A∩C)∪(B∩C) n(PnC)=n(A∩C)+n(B∩C)−n[(A∩C)∩(B∩C)] ⇒n(A∪B∪C)=n(A)+n(B)+(C)−n(A∩B) −n(A∩C)−n(B∩C)+n(A∩B∩C) c) Suppose one of the sets ,say A, is not a subset of the other,B. Then,∃ a_1 ∈A s.t. a_1 ∉B ⇒n(A∪B)>n(A∩B)⇒A⊆B⇒n(A∩B)=n(A) Suppose B\A ≠∅⇒n(B\A)=k≥1 then n(A∪B)=n(A)+n(B)−n(A∩B)=n(B) =n(B\A)+n(A)=k+n(A)>n(A∩B) ⇒n(B\A)=∅⇒A=B

$$\left.{b}\right)\:{n}\mid\left({A}\cup{B}\right)\cup{C}\mid={n}\left[{P}\cup{C}\right]\:{where}\:{P}={A}\cup{B} \\ $$$${n}\left({P}\cup{C}\right)={n}\left({P}\right)+{n}\left({C}\right)−{n}\left({P}\cap{C}\right) \\ $$$$={n}\left({A}\right)+{n}\left({B}\right)+{n}\left({C}\right)−{n}\left({A}\cap{B}\right)−{n}\left({P}\cap{C}\right) \\ $$$${P}\cap{C}=\left({A}\cap{B}\right)\cap{C}=\left({A}\cap{C}\right)\cup\left({B}\cap{C}\right) \\ $$$${n}\left({PnC}\right)={n}\left({A}\cap{C}\right)+{n}\left({B}\cap{C}\right)−{n}\left[\left({A}\cap{C}\right)\cap\left({B}\cap{C}\right)\right] \\ $$$$\Rightarrow{n}\left({A}\cup{B}\cup{C}\right)={n}\left({A}\right)+{n}\left({B}\right)+\left({C}\right)−{n}\left({A}\cap{B}\right) \\ $$$$−{n}\left({A}\cap{C}\right)−{n}\left({B}\cap{C}\right)+{n}\left({A}\cap{B}\cap{C}\right) \\ $$$$ \\ $$$$\left.{c}\right)\:{Suppose}\:{one}\:{of}\:{the}\:{sets}\:,{say}\:{A},\:{is}\:{not}\:{a}\:{subset} \\ $$$${of}\:{the}\:{other},{B}.\:{Then},\exists\:{a}_{\mathrm{1}} \in{A}\:{s}.{t}.\:{a}_{\mathrm{1}} \notin{B} \\ $$$$\Rightarrow{n}\left({A}\cup{B}\right)>{n}\left({A}\cap{B}\right)\Rightarrow{A}\subseteq{B}\Rightarrow{n}\left({A}\cap{B}\right)={n}\left({A}\right) \\ $$$${Suppose}\:{B}\backslash{A}\:\neq\emptyset\Rightarrow{n}\left({B}\backslash{A}\right)={k}\geqslant\mathrm{1} \\ $$$$\:{then}\:{n}\left({A}\cup{B}\right)={n}\left({A}\right)+{n}\left({B}\right)−{n}\left({A}\cap{B}\right)={n}\left({B}\right) \\ $$$$={n}\left({B}\backslash{A}\right)+{n}\left({A}\right)={k}+{n}\left({A}\right)>{n}\left({A}\cap{B}\right) \\ $$$$\Rightarrow{n}\left({B}\backslash{A}\right)=\emptyset\Rightarrow{A}={B} \\ $$

Commented by Mastermind last updated on 21/Jan/24

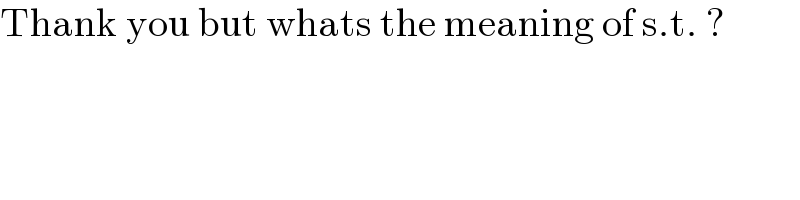
Thank you but whats the meaning of s.t. ?

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{but}\:\mathrm{whats}\:\mathrm{the}\:\mathrm{meaning}\:\mathrm{of}\:\mathrm{s}.\mathrm{t}.\:? \\ $$

Commented by Mastermind last updated on 21/Jan/24

besides i could not identify solution a and solution b

$$\mathrm{besides}\:\mathrm{i}\:\mathrm{could}\:\mathrm{not}\:\mathrm{identify}\:\mathrm{solution}\:\mathrm{a}\:\mathrm{and}\:\mathrm{solution}\:\mathrm{b} \\ $$

Commented by deleteduser1 last updated on 21/Jan/24

such that

$${such}\:{that} \\ $$

Commented by Mastermind last updated on 21/Jan/24

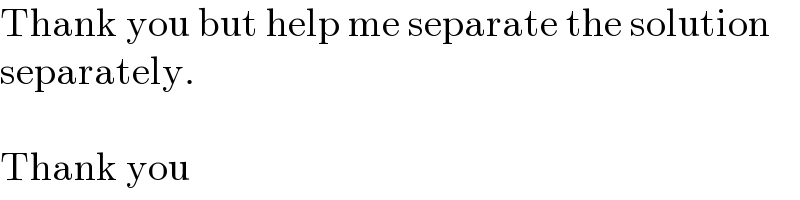
Thank you but help me separate the solution separately. Thank you

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{but}\:\mathrm{help}\:\mathrm{me}\:\mathrm{separate}\:\mathrm{the}\:\mathrm{solution} \\ $$$$\mathrm{separately}. \\ $$$$ \\ $$$$\mathrm{Thank}\:\mathrm{you} \\ $$

Commented by Mastermind last updated on 21/Jan/24

Where is the solution A ?

$$\mathrm{Where}\:\mathrm{is}\:\mathrm{the}\:\mathrm{solution}\:\mathrm{A}\:? \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com