Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 123681 by ZiYangLee last updated on 27/Nov/20

How many 3-digits positive integers are there such the sum of the digits is 10?

$$\mathrm{How}\:\mathrm{many}\:\mathrm{3}-\mathrm{digits}\:\mathrm{positive}\:\mathrm{integers} \\ $$$$\mathrm{are}\:\mathrm{there}\:\mathrm{such}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{digits}\: \\ $$$$\mathrm{is}\:\mathrm{10}? \\ $$

Answered by mr W last updated on 27/Nov/20

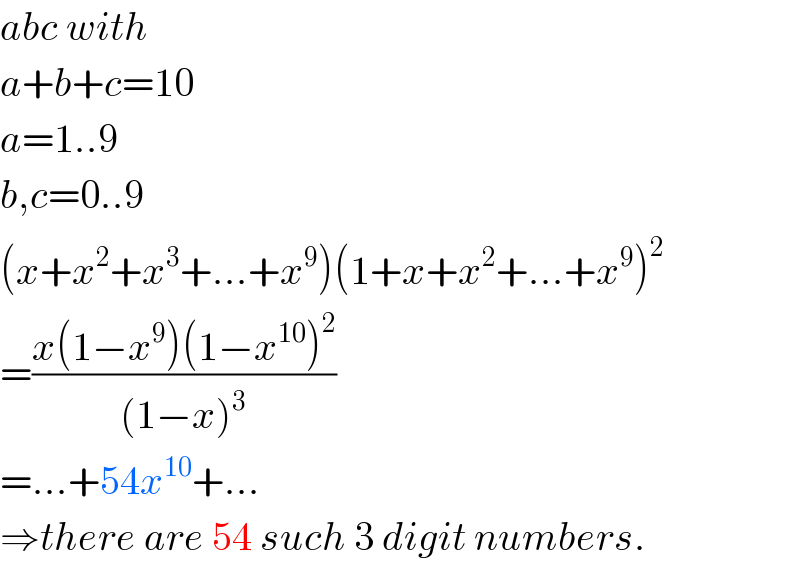
abc with a+b+c=10 a=1..9 b,c=0..9 (x+x^2 +x^3 +...+x^9 )(1+x+x^2 +...+x^9 )^2 =((x(1−x^9 )(1−x^(10) )^2 )/((1−x)^3 )) =...+54x^(10) +... ⇒there are 54 such 3 digit numbers.

$${abc}\:{with} \\ $$$${a}+{b}+{c}=\mathrm{10} \\ $$$${a}=\mathrm{1}..\mathrm{9} \\ $$$${b},{c}=\mathrm{0}..\mathrm{9} \\ $$$$\left({x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} +...+{x}^{\mathrm{9}} \right)\left(\mathrm{1}+{x}+{x}^{\mathrm{2}} +...+{x}^{\mathrm{9}} \right)^{\mathrm{2}} \\ $$$$=\frac{{x}\left(\mathrm{1}−{x}^{\mathrm{9}} \right)\left(\mathrm{1}−{x}^{\mathrm{10}} \right)^{\mathrm{2}} }{\left(\mathrm{1}−{x}\right)^{\mathrm{3}} } \\ $$$$=...+\mathrm{54}{x}^{\mathrm{10}} +... \\ $$$$\Rightarrow{there}\:{are}\:\mathrm{54}\:{such}\:\mathrm{3}\:{digit}\:{numbers}. \\ $$

Commented by malwan last updated on 27/Nov/20

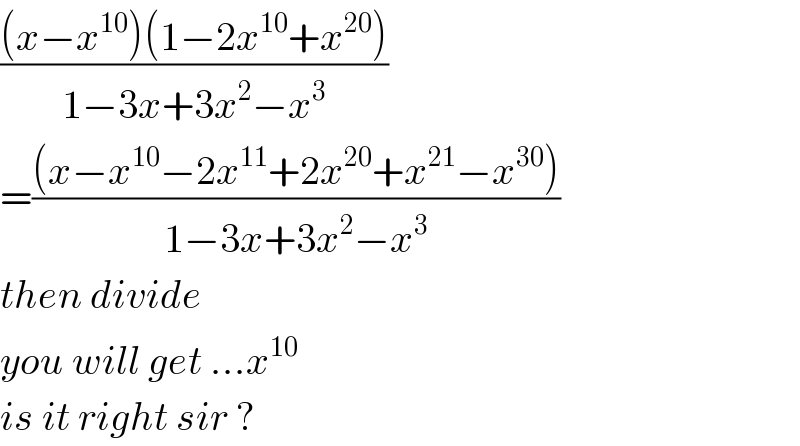
(((x−x^(10) )(1−2x^(10) +x^(20) ))/(1−3x+3x^2 −x^3 )) =(((x−x^(10) −2x^(11) +2x^(20) +x^(21) −x^(30) ))/(1−3x+3x^2 −x^3 )) then divide you will get ...x^(10) is it right sir ?

$$\frac{\left({x}−{x}^{\mathrm{10}} \right)\left(\mathrm{1}−\mathrm{2}{x}^{\mathrm{10}} +{x}^{\mathrm{20}} \right)}{\mathrm{1}−\mathrm{3}{x}+\mathrm{3}{x}^{\mathrm{2}} −{x}^{\mathrm{3}} } \\ $$$$=\frac{\left({x}−{x}^{\mathrm{10}} −\mathrm{2}{x}^{\mathrm{11}} +\mathrm{2}{x}^{\mathrm{20}} +{x}^{\mathrm{21}} −{x}^{\mathrm{30}} \right)}{\mathrm{1}−\mathrm{3}{x}+\mathrm{3}{x}^{\mathrm{2}} −{x}^{\mathrm{3}} } \\ $$$${then}\:{divide}\: \\ $$$${you}\:{will}\:{get}\:...{x}^{\mathrm{10}} \\ $$$${is}\:{it}\:{right}\:{sir}\:? \\ $$

Commented by mr W last updated on 27/Nov/20

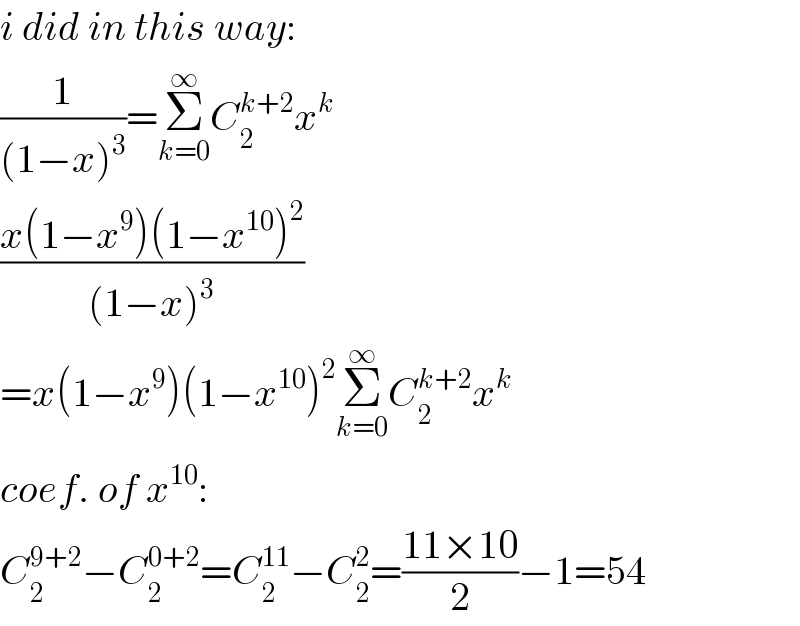
i did in this way: (1/((1−x)^3 ))=Σ_(k=0) ^∞ C_2 ^(k+2) x^k ((x(1−x^9 )(1−x^(10) )^2 )/((1−x)^3 )) =x(1−x^9 )(1−x^(10) )^2 Σ_(k=0) ^∞ C_2 ^(k+2) x^k coef. of x^(10) : C_2 ^(9+2) −C_2 ^(0+2) =C_2 ^(11) −C_2 ^2 =((11×10)/2)−1=54

$${i}\:{did}\:{in}\:{this}\:{way}: \\ $$$$\frac{\mathrm{1}}{\left(\mathrm{1}−{x}\right)^{\mathrm{3}} }=\underset{{k}=\mathrm{0}} {\overset{\infty} {\sum}}{C}_{\mathrm{2}} ^{{k}+\mathrm{2}} {x}^{{k}} \\ $$$$\frac{{x}\left(\mathrm{1}−{x}^{\mathrm{9}} \right)\left(\mathrm{1}−{x}^{\mathrm{10}} \right)^{\mathrm{2}} }{\left(\mathrm{1}−{x}\right)^{\mathrm{3}} } \\ $$$$={x}\left(\mathrm{1}−{x}^{\mathrm{9}} \right)\left(\mathrm{1}−{x}^{\mathrm{10}} \right)^{\mathrm{2}} \underset{{k}=\mathrm{0}} {\overset{\infty} {\sum}}{C}_{\mathrm{2}} ^{{k}+\mathrm{2}} {x}^{{k}} \\ $$$${coef}.\:{of}\:{x}^{\mathrm{10}} : \\ $$$${C}_{\mathrm{2}} ^{\mathrm{9}+\mathrm{2}} −{C}_{\mathrm{2}} ^{\mathrm{0}+\mathrm{2}} ={C}_{\mathrm{2}} ^{\mathrm{11}} −{C}_{\mathrm{2}} ^{\mathrm{2}} =\frac{\mathrm{11}×\mathrm{10}}{\mathrm{2}}−\mathrm{1}=\mathrm{54} \\ $$

Commented by malwan last updated on 28/Nov/20

fantastic sir thank you

$${fantastic}\:{sir} \\ $$$${thank}\:{you} \\ $$

Answered by floor(10²Eta[1]) last updated on 27/Nov/20

109 118 127 136 145 154 163 172 181 190 (10 cases) 208 217 226 235 244 253 262 271 280 (9 cases) 307 316 325 334 343 352 361 370 (8 cases) ... 901 910 (2 cases) total cases: 10+9+8+...+2=54

$$\mathrm{109} \\ $$$$\mathrm{118} \\ $$$$\mathrm{127} \\ $$$$\mathrm{136} \\ $$$$\mathrm{145} \\ $$$$\mathrm{154} \\ $$$$\mathrm{163} \\ $$$$\mathrm{172} \\ $$$$\mathrm{181} \\ $$$$\mathrm{190} \\ $$$$\left(\mathrm{10}\:\mathrm{cases}\right) \\ $$$$\mathrm{208} \\ $$$$\mathrm{217} \\ $$$$\mathrm{226} \\ $$$$\mathrm{235} \\ $$$$\mathrm{244} \\ $$$$\mathrm{253} \\ $$$$\mathrm{262} \\ $$$$\mathrm{271} \\ $$$$\mathrm{280} \\ $$$$\left(\mathrm{9}\:\mathrm{cases}\right) \\ $$$$\mathrm{307} \\ $$$$\mathrm{316} \\ $$$$\mathrm{325} \\ $$$$\mathrm{334} \\ $$$$\mathrm{343} \\ $$$$\mathrm{352} \\ $$$$\mathrm{361} \\ $$$$\mathrm{370} \\ $$$$\left(\mathrm{8}\:\mathrm{cases}\right) \\ $$$$... \\ $$$$\mathrm{901} \\ $$$$\mathrm{910} \\ $$$$\left(\mathrm{2}\:\mathrm{cases}\right) \\ $$$$\mathrm{total}\:\mathrm{cases}:\:\mathrm{10}+\mathrm{9}+\mathrm{8}+...+\mathrm{2}=\mathrm{54} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com