Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 132753 by liberty last updated on 16/Feb/21

Given x,y ∈R , x,y≠ 0 Find the maximum and minimum value of ((xy−4y^2 )/(x^2 +4y^2 ))

$$\mathrm{Given}\:\mathrm{x},\mathrm{y}\:\in\mathbb{R}\:,\:\mathrm{x},\mathrm{y}\neq\:\mathrm{0} \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{maximum}\:\mathrm{and}\:\mathrm{minimum} \\ $$$$\mathrm{value}\:\mathrm{of}\:\frac{\mathrm{xy}−\mathrm{4y}^{\mathrm{2}} }{\mathrm{x}^{\mathrm{2}} +\mathrm{4y}^{\mathrm{2}} }\: \\ $$

Commented by mr W last updated on 16/Feb/21

min=−(((√5)+2)/4) max=(((√5)−2)/4)

$${min}=−\frac{\sqrt{\mathrm{5}}+\mathrm{2}}{\mathrm{4}} \\ $$$${max}=\frac{\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{4}} \\ $$

Commented by liberty last updated on 16/Feb/21

step?

$$\mathrm{step}? \\ $$

Answered by EDWIN88 last updated on 16/Feb/21

let z=f(x,y) = ((xy−4y^2 )/(x^2 +4y^2 )) (1) (∂z/∂x) = ((y(x^2 +4y^2 )−2x(xy−4y^2 ))/((x^2 +4y^2 )^2 )) = 0 we get x^2 y+4y^3 −2x^2 y+8xy^2 = 0...(i) 4y^3 −x^2 y+8xy^2 = 0 (2) (∂z/∂y) = (((x−8y)(x^2 +4y^2 )−8y(xy−4y^2 ))/((x^2 +4y^2 )^2 ))= 0 we get x^3 +4xy^2 −8x^2 y−32y^3 −8xy^2 +32y^3 = 0 x^3 −4xy^2 −8xy = 0 or x^2 −4y^2 −8y = 0 ...(ii) adding (1) and (2) ⇒ 8xy−8y = 0 ⇒x = 1 and 4y^2 +8y−1=0 y = −1± ((√5)/2) then critical point is A(1,−1+((√5)/2) ) and B = (1,−1−((√5)/2)) for A⇒ z=((y(x−4y))/(x^2 +4y^2 )) = (((√5)−2)/4) ≈ 0.05901 for B⇒z=((−(√5)−2)/4) ≈ −1.05901

$$\mathrm{let}\:\mathrm{z}=\mathrm{f}\left(\mathrm{x},\mathrm{y}\right)\:=\:\frac{\mathrm{xy}−\mathrm{4y}^{\mathrm{2}} }{\mathrm{x}^{\mathrm{2}} +\mathrm{4y}^{\mathrm{2}} } \\ $$$$\left(\mathrm{1}\right)\:\frac{\partial\mathrm{z}}{\partial\mathrm{x}}\:=\:\frac{\mathrm{y}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{4y}^{\mathrm{2}} \right)−\mathrm{2x}\left(\mathrm{xy}−\mathrm{4y}^{\mathrm{2}} \right)}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{4y}^{\mathrm{2}} \right)^{\mathrm{2}} }\:=\:\mathrm{0} \\ $$$$\:\mathrm{we}\:\mathrm{get}\:\mathrm{x}^{\mathrm{2}} \mathrm{y}+\mathrm{4y}^{\mathrm{3}} −\mathrm{2x}^{\mathrm{2}} \mathrm{y}+\mathrm{8xy}^{\mathrm{2}} =\:\mathrm{0}...\left(\mathrm{i}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{4y}^{\mathrm{3}} −\mathrm{x}^{\mathrm{2}} \mathrm{y}+\mathrm{8xy}^{\mathrm{2}} \:=\:\mathrm{0} \\ $$$$\left(\mathrm{2}\right)\:\frac{\partial\mathrm{z}}{\partial\mathrm{y}}\:=\:\frac{\left(\mathrm{x}−\mathrm{8y}\right)\left(\mathrm{x}^{\mathrm{2}} +\mathrm{4y}^{\mathrm{2}} \right)−\mathrm{8y}\left(\mathrm{xy}−\mathrm{4y}^{\mathrm{2}} \right)}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{4y}^{\mathrm{2}} \right)^{\mathrm{2}} }=\:\mathrm{0} \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{x}^{\mathrm{3}} +\mathrm{4xy}^{\mathrm{2}} −\mathrm{8x}^{\mathrm{2}} \mathrm{y}−\mathrm{32y}^{\mathrm{3}} −\mathrm{8xy}^{\mathrm{2}} +\mathrm{32y}^{\mathrm{3}} \:=\:\mathrm{0} \\ $$$$\:\:\:\:\mathrm{x}^{\mathrm{3}} −\mathrm{4xy}^{\mathrm{2}} −\mathrm{8xy}\:=\:\mathrm{0}\:\mathrm{or}\:\mathrm{x}^{\mathrm{2}} −\mathrm{4y}^{\mathrm{2}} −\mathrm{8y}\:=\:\mathrm{0}\:...\left(\mathrm{ii}\right) \\ $$$$\mathrm{adding}\:\left(\mathrm{1}\right)\:\mathrm{and}\:\left(\mathrm{2}\right) \\ $$$$\Rightarrow\:\mathrm{8xy}−\mathrm{8y}\:=\:\mathrm{0}\:\Rightarrow\mathrm{x}\:=\:\mathrm{1}\:\:\mathrm{and}\:\mathrm{4y}^{\mathrm{2}} +\mathrm{8y}−\mathrm{1}=\mathrm{0} \\ $$$$\:\mathrm{y}\:=\:−\mathrm{1}\pm\:\frac{\sqrt{\mathrm{5}}}{\mathrm{2}}\:\mathrm{then}\:\mathrm{critical}\:\mathrm{point}\:\mathrm{is}\: \\ $$$$\mathrm{A}\left(\mathrm{1},−\mathrm{1}+\frac{\sqrt{\mathrm{5}}}{\mathrm{2}}\:\right)\:\mathrm{and}\:\mathrm{B}\:=\:\left(\mathrm{1},−\mathrm{1}−\frac{\sqrt{\mathrm{5}}}{\mathrm{2}}\right) \\ $$$$\mathrm{for}\:\mathrm{A}\Rightarrow\:\mathrm{z}=\frac{\mathrm{y}\left(\mathrm{x}−\mathrm{4y}\right)}{\mathrm{x}^{\mathrm{2}} +\mathrm{4y}^{\mathrm{2}} }\:=\:\frac{\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{4}}\:\approx\:\mathrm{0}.\mathrm{05901} \\ $$$$\mathrm{for}\:\mathrm{B}\Rightarrow\mathrm{z}=\frac{−\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{4}}\:\approx\:−\mathrm{1}.\mathrm{05901} \\ $$

Commented by EDWIN88 last updated on 16/Feb/21

yes sir. thank you

$$\mathrm{yes}\:\mathrm{sir}.\:\mathrm{thank}\:\mathrm{you} \\ $$

Commented by liberty last updated on 16/Feb/21

waw...

$$\mathrm{waw}... \\ $$

Commented by liberty last updated on 16/Feb/21

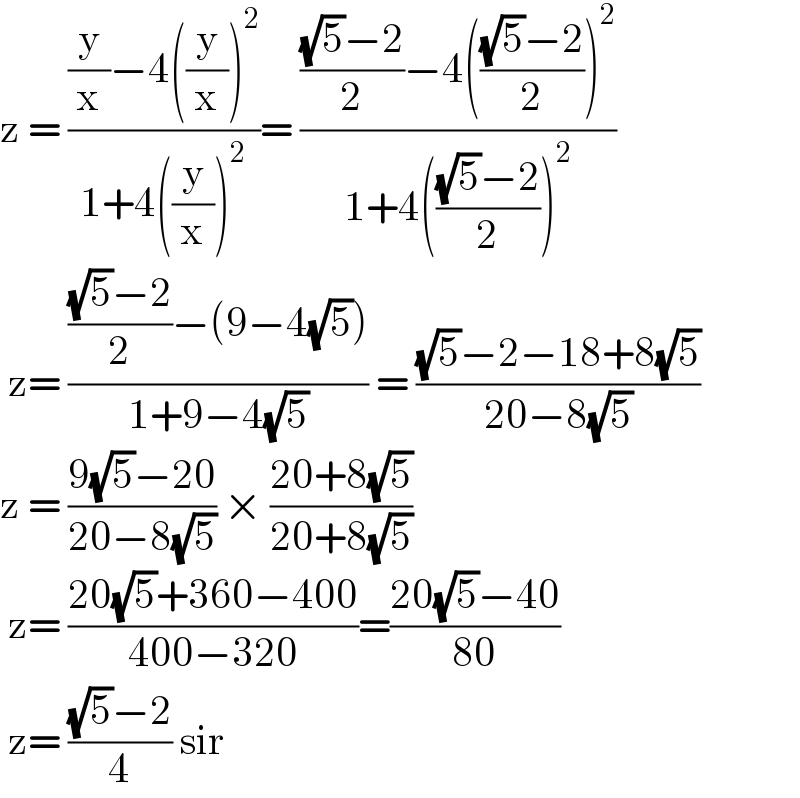
z = (((y/x)−4((y/x))^2 )/(1+4((y/x))^2 ))= (((((√5)−2)/2)−4((((√5)−2)/2))^2 )/(1+4((((√5)−2)/2))^2 )) z= (((((√5)−2)/2)−(9−4(√5)))/(1+9−4(√5))) = (((√5)−2−18+8(√5))/(20−8(√5))) z = ((9(√5)−20)/(20−8(√5))) × ((20+8(√5))/(20+8(√5))) z= ((20(√5)+360−400)/(400−320))=((20(√5)−40)/(80)) z= (((√5)−2)/4) sir

$$\mathrm{z}\:=\:\frac{\frac{\mathrm{y}}{\mathrm{x}}−\mathrm{4}\left(\frac{\mathrm{y}}{\mathrm{x}}\right)^{\mathrm{2}} }{\mathrm{1}+\mathrm{4}\left(\frac{\mathrm{y}}{\mathrm{x}}\right)^{\mathrm{2}} }=\:\frac{\frac{\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{2}}−\mathrm{4}\left(\frac{\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{2}}\right)^{\mathrm{2}} }{\mathrm{1}+\mathrm{4}\left(\frac{\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{2}}\right)^{\mathrm{2}} } \\ $$$$\:\mathrm{z}=\:\frac{\frac{\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{2}}−\left(\mathrm{9}−\mathrm{4}\sqrt{\mathrm{5}}\right)}{\mathrm{1}+\mathrm{9}−\mathrm{4}\sqrt{\mathrm{5}}}\:=\:\frac{\sqrt{\mathrm{5}}−\mathrm{2}−\mathrm{18}+\mathrm{8}\sqrt{\mathrm{5}}}{\mathrm{20}−\mathrm{8}\sqrt{\mathrm{5}}} \\ $$$$\mathrm{z}\:=\:\frac{\mathrm{9}\sqrt{\mathrm{5}}−\mathrm{20}}{\mathrm{20}−\mathrm{8}\sqrt{\mathrm{5}}}\:×\:\frac{\mathrm{20}+\mathrm{8}\sqrt{\mathrm{5}}}{\mathrm{20}+\mathrm{8}\sqrt{\mathrm{5}}} \\ $$$$\:\mathrm{z}=\:\frac{\mathrm{20}\sqrt{\mathrm{5}}+\mathrm{360}−\mathrm{400}}{\mathrm{400}−\mathrm{320}}=\frac{\mathrm{20}\sqrt{\mathrm{5}}−\mathrm{40}}{\mathrm{80}} \\ $$$$\:\mathrm{z}=\:\frac{\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{4}}\:\mathrm{sir} \\ $$

Answered by mr W last updated on 16/Feb/21

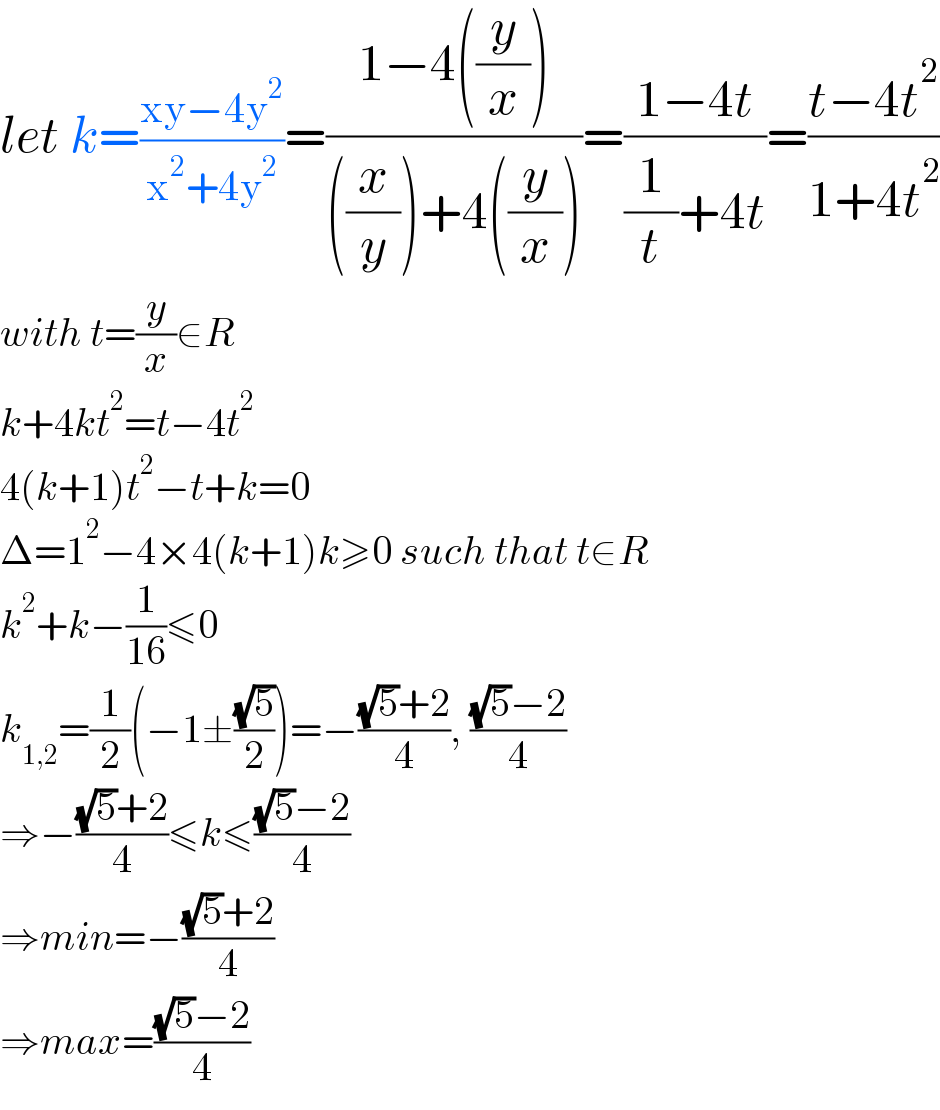
let k=((xy−4y^2 )/(x^2 +4y^2 ))=((1−4((y/x)))/(((x/y))+4((y/x))))=((1−4t)/((1/t)+4t))=((t−4t^2 )/(1+4t^2 )) with t=(y/x)∈R k+4kt^2 =t−4t^2 4(k+1)t^2 −t+k=0 Δ=1^2 −4×4(k+1)k≥0 such that t∈R k^2 +k−(1/(16))≤0 k_(1,2) =(1/2)(−1±((√5)/2))=−(((√5)+2)/4), (((√5)−2)/4) ⇒−(((√5)+2)/4)≤k≤(((√5)−2)/4) ⇒min=−(((√5)+2)/4) ⇒max=(((√5)−2)/4)

$${let}\:{k}=\frac{\mathrm{xy}−\mathrm{4y}^{\mathrm{2}} }{\mathrm{x}^{\mathrm{2}} +\mathrm{4y}^{\mathrm{2}} }=\frac{\mathrm{1}−\mathrm{4}\left(\frac{{y}}{{x}}\right)}{\left(\frac{{x}}{{y}}\right)+\mathrm{4}\left(\frac{{y}}{{x}}\right)}=\frac{\mathrm{1}−\mathrm{4}{t}}{\frac{\mathrm{1}}{{t}}+\mathrm{4}{t}}=\frac{{t}−\mathrm{4}{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{4}{t}^{\mathrm{2}} } \\ $$$${with}\:{t}=\frac{{y}}{{x}}\in{R} \\ $$$${k}+\mathrm{4}{kt}^{\mathrm{2}} ={t}−\mathrm{4}{t}^{\mathrm{2}} \\ $$$$\mathrm{4}\left({k}+\mathrm{1}\right){t}^{\mathrm{2}} −{t}+{k}=\mathrm{0} \\ $$$$\Delta=\mathrm{1}^{\mathrm{2}} −\mathrm{4}×\mathrm{4}\left({k}+\mathrm{1}\right){k}\geqslant\mathrm{0}\:{such}\:{that}\:{t}\in{R} \\ $$$${k}^{\mathrm{2}} +{k}−\frac{\mathrm{1}}{\mathrm{16}}\leqslant\mathrm{0} \\ $$$${k}_{\mathrm{1},\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{2}}\left(−\mathrm{1}\pm\frac{\sqrt{\mathrm{5}}}{\mathrm{2}}\right)=−\frac{\sqrt{\mathrm{5}}+\mathrm{2}}{\mathrm{4}},\:\frac{\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{4}} \\ $$$$\Rightarrow−\frac{\sqrt{\mathrm{5}}+\mathrm{2}}{\mathrm{4}}\leqslant{k}\leqslant\frac{\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{4}} \\ $$$$\Rightarrow{min}=−\frac{\sqrt{\mathrm{5}}+\mathrm{2}}{\mathrm{4}} \\ $$$$\Rightarrow{max}=\frac{\sqrt{\mathrm{5}}−\mathrm{2}}{\mathrm{4}} \\ $$

Commented by liberty last updated on 16/Feb/21

thank you sir

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com