Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 158259 by cortano last updated on 01/Nov/21

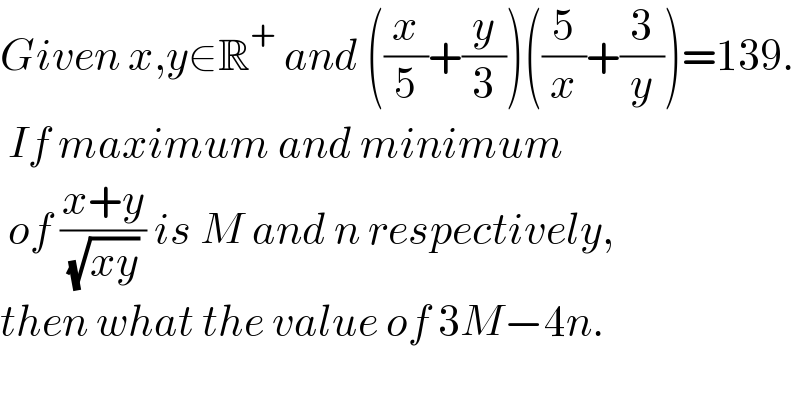
Given x,y∈R^+ and ((x/5)+(y/3))((5/x)+(3/y))=139. If maximum and minimum of ((x+y)/( (√(xy)) )) is M and n respectively, then what the value of 3M−4n.

$${Given}\:{x},{y}\in\mathbb{R}^{+} \:{and}\:\left(\frac{{x}}{\mathrm{5}}+\frac{{y}}{\mathrm{3}}\right)\left(\frac{\mathrm{5}}{{x}}+\frac{\mathrm{3}}{{y}}\right)=\mathrm{139}. \\ $$$$\:{If}\:{maximum}\:{and}\:{minimum} \\ $$$$\:{of}\:\frac{{x}+{y}}{\:\sqrt{{xy}}\:}\:{is}\:{M}\:{and}\:{n}\:{respectively}, \\ $$$${then}\:{what}\:{the}\:{value}\:{of}\:\mathrm{3}{M}−\mathrm{4}{n}. \\ $$

Commented by tounghoungko last updated on 01/Nov/21

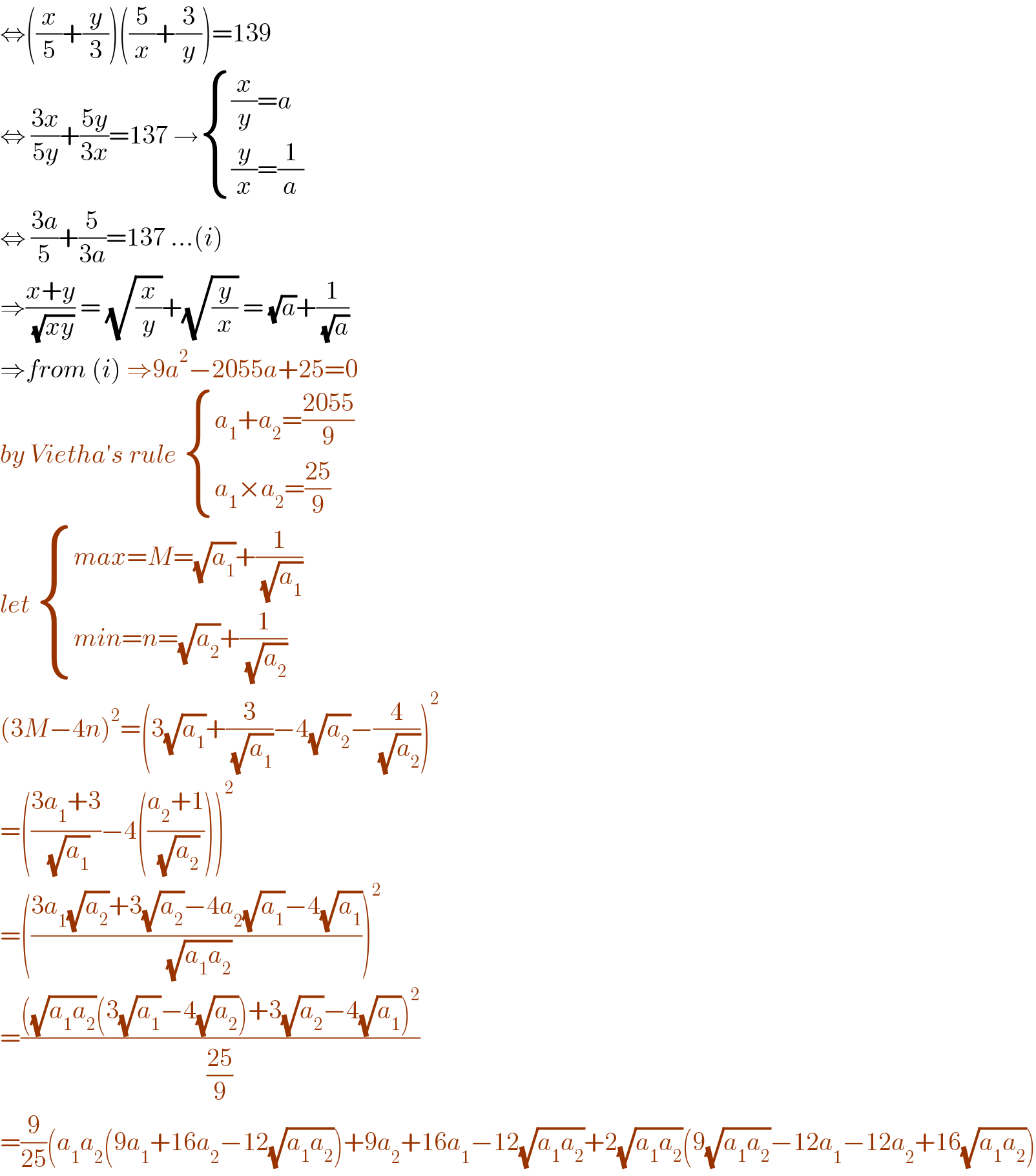
⇔((x/5)+(y/3))((5/x)+(3/y))=139 ⇔ ((3x)/(5y))+((5y)/(3x))=137 → { (((x/y)=a)),(((y/x)=(1/a))) :} ⇔ ((3a)/5)+(5/(3a))=137 ...(i) ⇒((x+y)/( (√(xy)))) = (√(x/y))+(√(y/x)) = (√a)+(1/( (√a))) ⇒from (i) ⇒9a^2 −2055a+25=0 by Vietha′s rule { ((a_1 +a_2 =((2055)/9))),((a_1 ×a_2 =((25)/9))) :} let { ((max=M=(√a_1 )+(1/( (√a_1 ))))),((min=n=(√a_2 )+(1/( (√a_2 ))) )) :} (3M−4n)^2 =(3(√a_1 )+(3/( (√a_1 )))−4(√a_2 )−(4/( (√a_2 ))))^2 =(((3a_1 +3)/( (√a_1 )))−4(((a_2 +1)/( (√a_2 )))))^2 =(((3a_1 (√a_2 )+3(√a_2 )−4a_2 (√a_1 )−4(√a_1 ))/( (√(a_1 a_2 )))))^2 =((((√(a_1 a_2 ))(3(√a_1 )−4(√a_2 ))+3(√a_2 )−4(√a_1 ))^2 )/((25)/9)) =(9/(25))(a_1 a_2 (9a_1 +16a_2 −12(√(a_1 a_2 )))+9a_2 +16a_1 −12(√(a_1 a_2 ))+2(√(a_1 a_2 ))(9(√(a_1 a_2 ))−12a_1 −12a_2 +16(√(a_1 a_2 )))

$$\Leftrightarrow\left(\frac{{x}}{\mathrm{5}}+\frac{{y}}{\mathrm{3}}\right)\left(\frac{\mathrm{5}}{{x}}+\frac{\mathrm{3}}{{y}}\right)=\mathrm{139} \\ $$$$\Leftrightarrow\:\frac{\mathrm{3}{x}}{\mathrm{5}{y}}+\frac{\mathrm{5}{y}}{\mathrm{3}{x}}=\mathrm{137}\:\rightarrow\begin{cases}{\frac{{x}}{{y}}={a}}\\{\frac{{y}}{{x}}=\frac{\mathrm{1}}{{a}}}\end{cases} \\ $$$$\Leftrightarrow\:\frac{\mathrm{3}{a}}{\mathrm{5}}+\frac{\mathrm{5}}{\mathrm{3}{a}}=\mathrm{137}\:...\left({i}\right) \\ $$$$\Rightarrow\frac{{x}+{y}}{\:\sqrt{{xy}}}\:=\:\sqrt{\frac{{x}}{{y}}}+\sqrt{\frac{{y}}{{x}}}\:=\:\sqrt{{a}}+\frac{\mathrm{1}}{\:\sqrt{{a}}} \\ $$$$\Rightarrow{from}\:\left({i}\right)\:\Rightarrow\mathrm{9}{a}^{\mathrm{2}} −\mathrm{2055}{a}+\mathrm{25}=\mathrm{0} \\ $$$${by}\:{Vietha}'{s}\:{rule}\:\begin{cases}{{a}_{\mathrm{1}} +{a}_{\mathrm{2}} =\frac{\mathrm{2055}}{\mathrm{9}}}\\{{a}_{\mathrm{1}} ×{a}_{\mathrm{2}} =\frac{\mathrm{25}}{\mathrm{9}}}\end{cases} \\ $$$${let}\:\begin{cases}{{max}={M}=\sqrt{{a}_{\mathrm{1}} }+\frac{\mathrm{1}}{\:\sqrt{{a}_{\mathrm{1}} }}}\\{{min}={n}=\sqrt{{a}_{\mathrm{2}} }+\frac{\mathrm{1}}{\:\sqrt{{a}_{\mathrm{2}} }}\:}\end{cases} \\ $$$$\left(\mathrm{3}{M}−\mathrm{4}{n}\right)^{\mathrm{2}} =\left(\mathrm{3}\sqrt{{a}_{\mathrm{1}} }+\frac{\mathrm{3}}{\:\sqrt{{a}_{\mathrm{1}} }}−\mathrm{4}\sqrt{{a}_{\mathrm{2}} }−\frac{\mathrm{4}}{\:\sqrt{{a}_{\mathrm{2}} }}\right)^{\mathrm{2}} \\ $$$$=\left(\frac{\mathrm{3}{a}_{\mathrm{1}} +\mathrm{3}}{\:\sqrt{{a}_{\mathrm{1}} }}−\mathrm{4}\left(\frac{{a}_{\mathrm{2}} +\mathrm{1}}{\:\sqrt{{a}_{\mathrm{2}} }}\right)\right)^{\mathrm{2}} \\ $$$$=\left(\frac{\mathrm{3}{a}_{\mathrm{1}} \sqrt{{a}_{\mathrm{2}} }+\mathrm{3}\sqrt{{a}_{\mathrm{2}} }−\mathrm{4}{a}_{\mathrm{2}} \sqrt{{a}_{\mathrm{1}} }−\mathrm{4}\sqrt{{a}_{\mathrm{1}} }}{\:\sqrt{{a}_{\mathrm{1}} {a}_{\mathrm{2}} }}\right)^{\mathrm{2}} \\ $$$$=\frac{\left(\sqrt{{a}_{\mathrm{1}} {a}_{\mathrm{2}} }\left(\mathrm{3}\sqrt{{a}_{\mathrm{1}} }−\mathrm{4}\sqrt{{a}_{\mathrm{2}} }\right)+\mathrm{3}\sqrt{{a}_{\mathrm{2}} }−\mathrm{4}\sqrt{{a}_{\mathrm{1}} }\right)^{\mathrm{2}} }{\frac{\mathrm{25}}{\mathrm{9}}} \\ $$$$=\frac{\mathrm{9}}{\mathrm{25}}\left({a}_{\mathrm{1}} {a}_{\mathrm{2}} \left(\mathrm{9}{a}_{\mathrm{1}} +\mathrm{16}{a}_{\mathrm{2}} −\mathrm{12}\sqrt{{a}_{\mathrm{1}} {a}_{\mathrm{2}} }\right)+\mathrm{9}{a}_{\mathrm{2}} +\mathrm{16}{a}_{\mathrm{1}} −\mathrm{12}\sqrt{{a}_{\mathrm{1}} {a}_{\mathrm{2}} }+\mathrm{2}\sqrt{{a}_{\mathrm{1}} {a}_{\mathrm{2}} }\left(\mathrm{9}\sqrt{{a}_{\mathrm{1}} {a}_{\mathrm{2}} }−\mathrm{12}{a}_{\mathrm{1}} −\mathrm{12}{a}_{\mathrm{2}} +\mathrm{16}\sqrt{{a}_{\mathrm{1}} {a}_{\mathrm{2}} }\right)\right. \\ $$

Answered by mr W last updated on 01/Nov/21

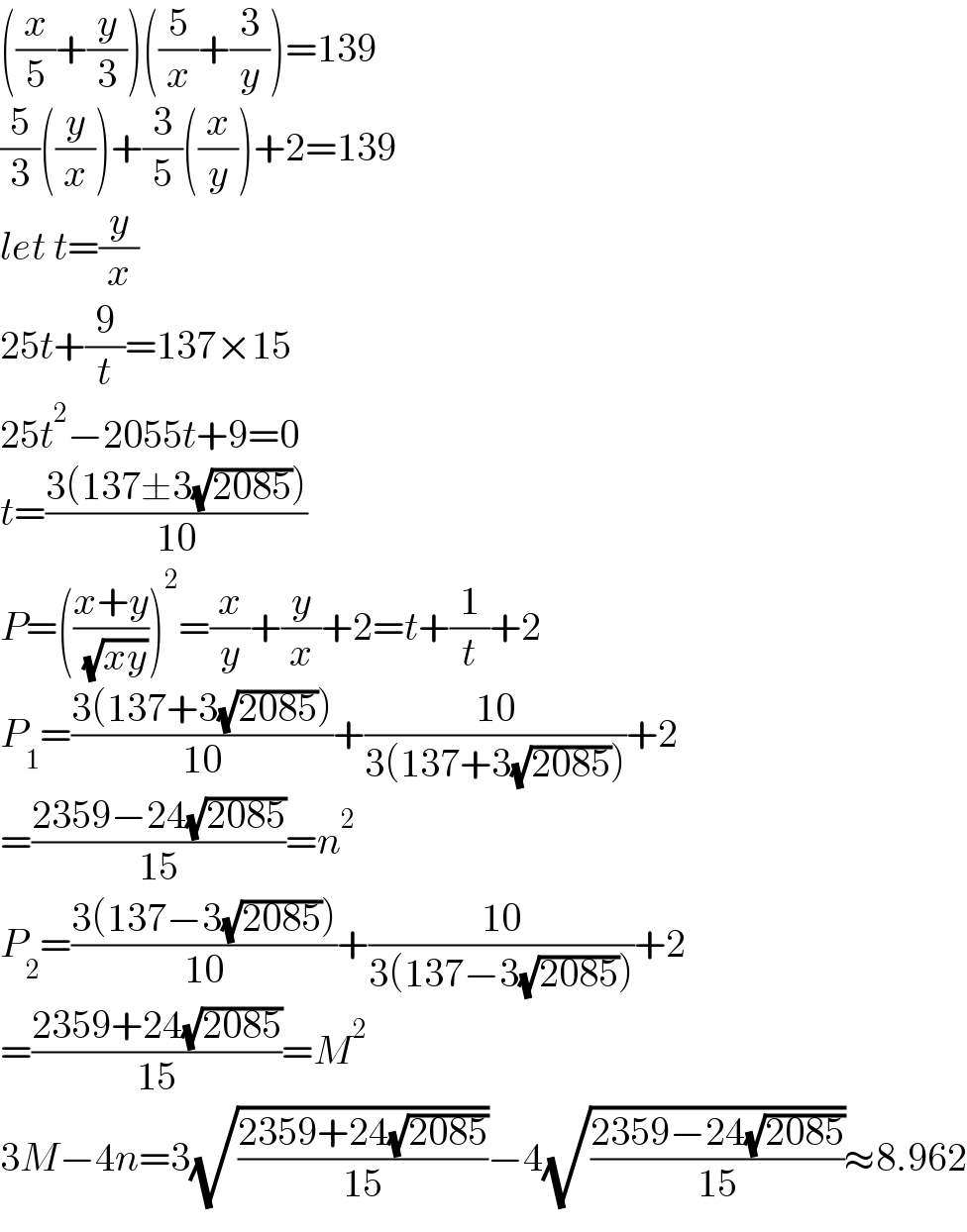
((x/5)+(y/3))((5/x)+(3/y))=139 (5/3)((y/x))+(3/5)((x/y))+2=139 let t=(y/x) 25t+(9/t)=137×15 25t^2 −2055t+9=0 t=((3(137±3(√(2085))))/(10)) P=(((x+y)/( (√(xy)))))^2 =(x/y)+(y/x)+2=t+(1/t)+2 P_1 =((3(137+3(√(2085))))/(10))+((10)/(3(137+3(√(2085)))))+2 =((2359−24(√(2085)))/(15))=n^2 P_2 =((3(137−3(√(2085))))/(10))+((10)/(3(137−3(√(2085)))))+2 =((2359+24(√(2085)))/(15))=M^2 3M−4n=3(√((2359+24(√(2085)))/(15)))−4(√((2359−24(√(2085)))/(15)))≈8.962

$$\left(\frac{{x}}{\mathrm{5}}+\frac{{y}}{\mathrm{3}}\right)\left(\frac{\mathrm{5}}{{x}}+\frac{\mathrm{3}}{{y}}\right)=\mathrm{139} \\ $$$$\frac{\mathrm{5}}{\mathrm{3}}\left(\frac{{y}}{{x}}\right)+\frac{\mathrm{3}}{\mathrm{5}}\left(\frac{{x}}{{y}}\right)+\mathrm{2}=\mathrm{139} \\ $$$${let}\:{t}=\frac{{y}}{{x}} \\ $$$$\mathrm{25}{t}+\frac{\mathrm{9}}{{t}}=\mathrm{137}×\mathrm{15} \\ $$$$\mathrm{25}{t}^{\mathrm{2}} −\mathrm{2055}{t}+\mathrm{9}=\mathrm{0} \\ $$$${t}=\frac{\mathrm{3}\left(\mathrm{137}\pm\mathrm{3}\sqrt{\mathrm{2085}}\right)}{\mathrm{10}} \\ $$$${P}=\left(\frac{{x}+{y}}{\:\sqrt{{xy}}}\right)^{\mathrm{2}} =\frac{{x}}{{y}}+\frac{{y}}{{x}}+\mathrm{2}={t}+\frac{\mathrm{1}}{{t}}+\mathrm{2} \\ $$$${P}_{\mathrm{1}} =\frac{\mathrm{3}\left(\mathrm{137}+\mathrm{3}\sqrt{\mathrm{2085}}\right)}{\mathrm{10}}+\frac{\mathrm{10}}{\mathrm{3}\left(\mathrm{137}+\mathrm{3}\sqrt{\mathrm{2085}}\right)}+\mathrm{2} \\ $$$$=\frac{\mathrm{2359}−\mathrm{24}\sqrt{\mathrm{2085}}}{\mathrm{15}}={n}^{\mathrm{2}} \\ $$$${P}_{\mathrm{2}} =\frac{\mathrm{3}\left(\mathrm{137}−\mathrm{3}\sqrt{\mathrm{2085}}\right)}{\mathrm{10}}+\frac{\mathrm{10}}{\mathrm{3}\left(\mathrm{137}−\mathrm{3}\sqrt{\mathrm{2085}}\right)}+\mathrm{2} \\ $$$$=\frac{\mathrm{2359}+\mathrm{24}\sqrt{\mathrm{2085}}}{\mathrm{15}}={M}^{\mathrm{2}} \\ $$$$\mathrm{3}{M}−\mathrm{4}{n}=\mathrm{3}\sqrt{\frac{\mathrm{2359}+\mathrm{24}\sqrt{\mathrm{2085}}}{\mathrm{15}}}−\mathrm{4}\sqrt{\frac{\mathrm{2359}−\mathrm{24}\sqrt{\mathrm{2085}}}{\mathrm{15}}}\approx\mathrm{8}.\mathrm{962} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com