Question and Answers Forum

All Questions      Topic List

Operation Research Questions

Previous in All Question      Next in All Question      

Previous in Operation Research      Next in Operation Research      

Question Number 119150 by abdul88 last updated on 22/Oct/20

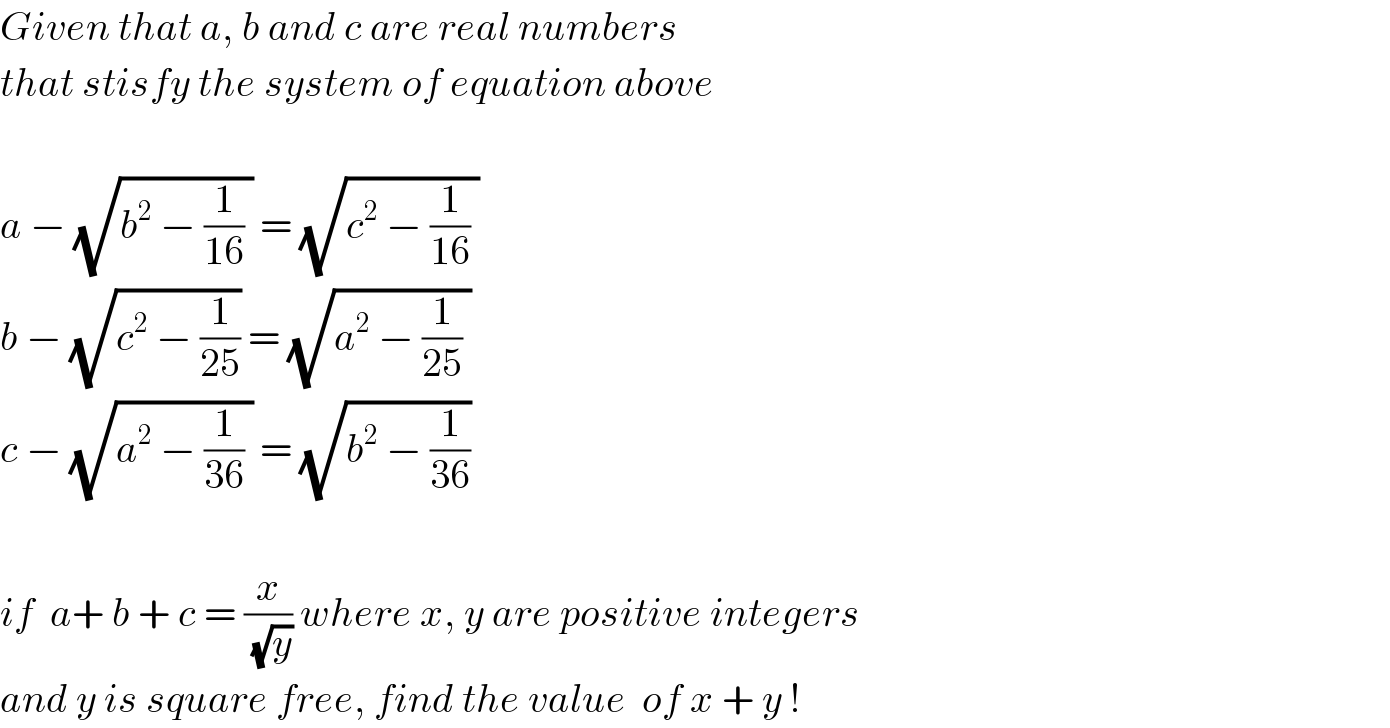
Given that a, b and c are real numbers that stisfy the system of equation above a − (√(b^2 − (1/(16)) )) = (√(c^2 − (1/(16)) )) b − (√(c^2 − (1/(25)))) = (√(a^2 − (1/(25)) )) c − (√(a^2 − (1/(36)) )) = (√(b^2 − (1/(36)))) if a+ b + c = (x/( (√y))) where x, y are positive integers and y is square free, find the value of x + y !

$${Given}\:{that}\:{a},\:{b}\:{and}\:{c}\:{are}\:{real}\:{numbers}\: \\ $$$${that}\:{stisfy}\:{the}\:{system}\:{of}\:{equation}\:{above} \\ $$$$ \\ $$$${a}\:−\:\sqrt{{b}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{16}}\:}\:=\:\sqrt{{c}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{16}}\:}\: \\ $$$${b}\:−\:\sqrt{{c}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{25}}}\:=\:\sqrt{{a}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{25}}\:} \\ $$$${c}\:−\:\sqrt{{a}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{36}}\:}\:=\:\sqrt{{b}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{36}}}\: \\ $$$$ \\ $$$${if}\:\:{a}+\:{b}\:+\:{c}\:=\:\frac{{x}}{\:\sqrt{{y}}}\:{where}\:{x},\:{y}\:{are}\:{positive}\:{integers} \\ $$$${and}\:{y}\:{is}\:{square}\:{free},\:{find}\:{the}\:{value}\:\:{of}\:{x}\:+\:{y}\:! \\ $$

Answered by MJS_new last updated on 22/Oct/20

the given equations have the shape o−(√(p^2 −r))=(√(q^2 −r)) o=(√(p^2 −r))+(√(q^2 −r)) o^2 =(p^2 −r)+(q^2 −r)+2(√(p^2 −r))(√(q^2 −r)) o^2 −p^2 −q^2 +2r=2(√(p^2 −r))(√(q^2 −r)) squaring again and transforming gives o^4 +p^4 +q^4 −2(o^2 p^2 +o^2 q^2 +p^2 q^2 )+4ro^2 =0 we see that o, p, q are interchangeable the only different term is 4ro^2 ⇒ substracting two equations we get (4/(16))a^2 −(4/(25))b^2 =0∧(4/(16))a^2 −(4/(36))c^2 =0 ⇒ b=(5/4)a∧c=(3/2)a inserting above we get a=((8(√7))/(105)) ⇒ b=((2(√7))/(21))∧c=((4(√7))/(35)) a+b+c=((2(√7))/7)=(2/( (√7))) ⇒ x=2∧y=7 ⇒ x+y=9

$$\mathrm{the}\:\mathrm{given}\:\mathrm{equations}\:\mathrm{have}\:\mathrm{the}\:\mathrm{shape} \\ $$$${o}−\sqrt{{p}^{\mathrm{2}} −{r}}=\sqrt{{q}^{\mathrm{2}} −{r}} \\ $$$${o}=\sqrt{{p}^{\mathrm{2}} −{r}}+\sqrt{{q}^{\mathrm{2}} −{r}} \\ $$$${o}^{\mathrm{2}} =\left({p}^{\mathrm{2}} −{r}\right)+\left({q}^{\mathrm{2}} −{r}\right)+\mathrm{2}\sqrt{{p}^{\mathrm{2}} −{r}}\sqrt{{q}^{\mathrm{2}} −{r}} \\ $$$${o}^{\mathrm{2}} −{p}^{\mathrm{2}} −{q}^{\mathrm{2}} +\mathrm{2}{r}=\mathrm{2}\sqrt{{p}^{\mathrm{2}} −{r}}\sqrt{{q}^{\mathrm{2}} −{r}} \\ $$$$\mathrm{squaring}\:\mathrm{again}\:\mathrm{and}\:\mathrm{transforming}\:\mathrm{gives} \\ $$$${o}^{\mathrm{4}} +{p}^{\mathrm{4}} +{q}^{\mathrm{4}} −\mathrm{2}\left({o}^{\mathrm{2}} {p}^{\mathrm{2}} +{o}^{\mathrm{2}} {q}^{\mathrm{2}} +{p}^{\mathrm{2}} {q}^{\mathrm{2}} \right)+\mathrm{4}{ro}^{\mathrm{2}} =\mathrm{0} \\ $$$$\mathrm{we}\:\mathrm{see}\:\mathrm{that}\:{o},\:{p},\:{q}\:\mathrm{are}\:\mathrm{interchangeable} \\ $$$$\mathrm{the}\:\mathrm{only}\:\mathrm{different}\:\mathrm{term}\:\mathrm{is}\:\mathrm{4}{ro}^{\mathrm{2}} \\ $$$$\Rightarrow\:\mathrm{substracting}\:\mathrm{two}\:\mathrm{equations}\:\mathrm{we}\:\mathrm{get} \\ $$$$\frac{\mathrm{4}}{\mathrm{16}}{a}^{\mathrm{2}} −\frac{\mathrm{4}}{\mathrm{25}}{b}^{\mathrm{2}} =\mathrm{0}\wedge\frac{\mathrm{4}}{\mathrm{16}}{a}^{\mathrm{2}} −\frac{\mathrm{4}}{\mathrm{36}}{c}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow\:{b}=\frac{\mathrm{5}}{\mathrm{4}}{a}\wedge{c}=\frac{\mathrm{3}}{\mathrm{2}}{a} \\ $$$$\mathrm{inserting}\:\mathrm{above}\:\mathrm{we}\:\mathrm{get} \\ $$$${a}=\frac{\mathrm{8}\sqrt{\mathrm{7}}}{\mathrm{105}}\:\Rightarrow\:{b}=\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{21}}\wedge{c}=\frac{\mathrm{4}\sqrt{\mathrm{7}}}{\mathrm{35}} \\ $$$${a}+{b}+{c}=\frac{\mathrm{2}\sqrt{\mathrm{7}}}{\mathrm{7}}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{7}}} \\ $$$$\Rightarrow\:{x}=\mathrm{2}\wedge{y}=\mathrm{7}\:\Rightarrow\:{x}+{y}=\mathrm{9} \\ $$

Commented by PRITHWISH SEN 2 last updated on 22/Oct/20

superb!^

$$\mathrm{superb}\overset{} {!} \\ $$

Commented by MJS_new last updated on 22/Oct/20

thank you

$$\mathrm{thank}\:\mathrm{you} \\ $$

Commented by abdul88 last updated on 23/Oct/20

thank you Sir....:)

$$ \\ $$$$\left.{thank}\:{you}\:{Sir}....:\right) \\ $$

Answered by 1549442205PVT last updated on 23/Oct/20

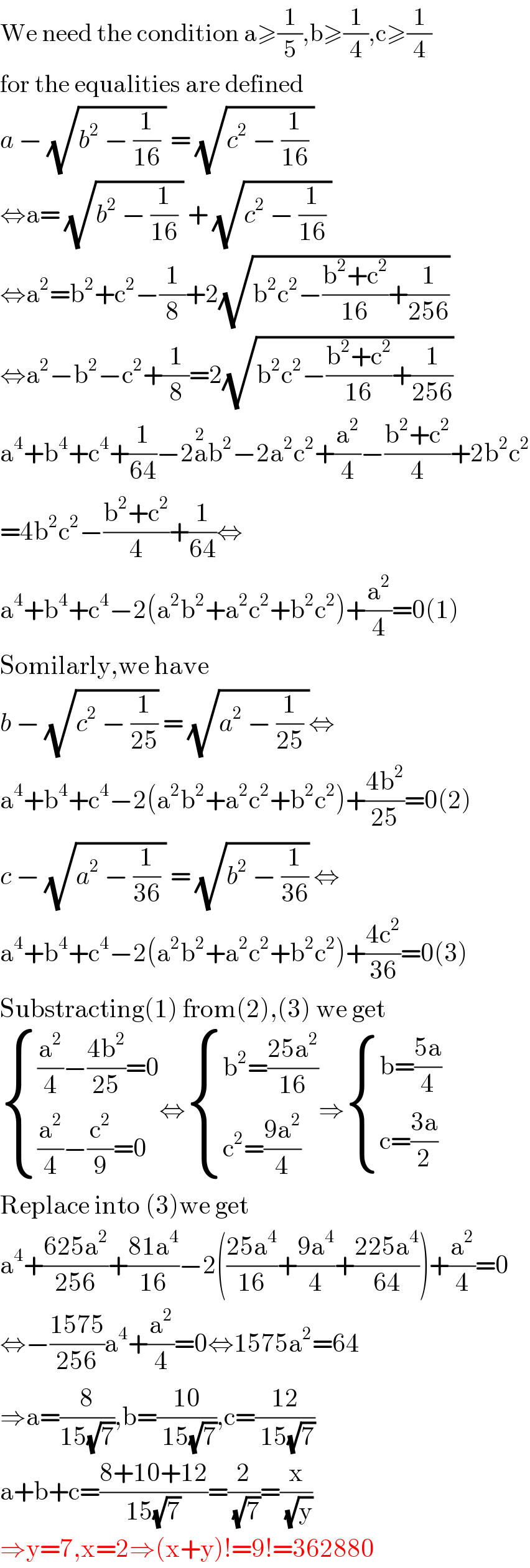
We need the condition a≥(1/5),b≥(1/4),c≥(1/4) for the equalities are defined a − (√(b^2 − (1/(16)) )) = (√(c^2 − (1/(16)) )) ⇔a= (√(b^2 − (1/(16)) )) + (√(c^2 − (1/(16)) )) ⇔a^2 =b^2 +c^2 −(1/8)+2(√(b^2 c^2 −((b^2 +c^2 )/(16))+(1/(256)))) ⇔a^2 −b^2 −c^2 +(1/8)=2(√(b^2 c^2 −((b^2 +c^2 )/(16))+(1/(256)))) a^4 +b^4 +c^4 +(1/(64))−2a^2 b^2 −2a^2 c^2 +(a^2 /4)−((b^2 +c^2 )/4)+2b^2 c^2 =4b^2 c^2 −((b^2 +c^2 )/4)+(1/(64))⇔ a^4 +b^4 +c^4 −2(a^2 b^2 +a^2 c^2 +b^2 c^2 )+(a^2 /4)=0(1) Somilarly,we have b − (√(c^2 − (1/(25)))) = (√(a^2 − (1/(25)) ))⇔ a^4 +b^4 +c^4 −2(a^2 b^2 +a^2 c^2 +b^2 c^2 )+((4b^2 )/(25))=0(2) c − (√(a^2 − (1/(36)) )) = (√(b^2 − (1/(36)))) ⇔ a^4 +b^4 +c^4 −2(a^2 b^2 +a^2 c^2 +b^2 c^2 )+((4c^2 )/(36))=0(3) Substracting(1) from(2),(3) we get { (((a^2 /4)−((4b^2 )/(25))=0)),(((a^2 /4)−(c^2 /9)=0)) :}⇔ { ((b^2 =((25a^2 )/(16)))),((c^2 =((9a^2 )/4))) :}⇒ { ((b=((5a)/4))),((c=((3a)/2))) :} Replace into (3)we get a^4 +((625a^2 )/(256))+((81a^4 )/(16))−2(((25a^4 )/(16))+((9a^4 )/4)+((225a^4 )/(64)))+(a^2 /4)=0 ⇔−((1575)/(256))a^4 +(a^2 /4)=0⇔1575a^2 =64 ⇒a=(8/(15(√7))),b=((10)/( 15(√7))),c=((12)/( 15(√7))) a+b+c=((8+10+12)/(15(√7)))=(2/( (√7)))=(x/( (√y))) ⇒y=7,x=2⇒(x+y)!=9!=362880

$$\mathrm{We}\:\mathrm{need}\:\mathrm{the}\:\mathrm{condition}\:\mathrm{a}\geqslant\frac{\mathrm{1}}{\mathrm{5}},\mathrm{b}\geqslant\frac{\mathrm{1}}{\mathrm{4}},\mathrm{c}\geqslant\frac{\mathrm{1}}{\mathrm{4}}\: \\ $$$$\mathrm{for}\:\mathrm{the}\:\mathrm{equalities}\:\mathrm{are}\:\mathrm{defined} \\ $$$${a}\:−\:\sqrt{{b}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{16}}\:}\:=\:\sqrt{{c}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{16}}\:}\: \\ $$$$\Leftrightarrow\mathrm{a}=\:\sqrt{{b}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{16}}\:}\:+\:\sqrt{{c}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{16}}\:}\: \\ $$$$\Leftrightarrow\mathrm{a}^{\mathrm{2}} =\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{8}}+\mathrm{2}\sqrt{\mathrm{b}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} −\frac{\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} }{\mathrm{16}}+\frac{\mathrm{1}}{\mathrm{256}}} \\ $$$$\Leftrightarrow\mathrm{a}^{\mathrm{2}} −\mathrm{b}^{\mathrm{2}} −\mathrm{c}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{8}}=\mathrm{2}\sqrt{\mathrm{b}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} −\frac{\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} }{\mathrm{16}}+\frac{\mathrm{1}}{\mathrm{256}}} \\ $$$$\mathrm{a}^{\mathrm{4}} +\mathrm{b}^{\mathrm{4}} +\mathrm{c}^{\mathrm{4}} +\frac{\mathrm{1}}{\mathrm{64}}−\mathrm{2}\overset{\mathrm{2}} {\mathrm{a}b}^{\mathrm{2}} −\mathrm{2a}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} +\frac{\mathrm{a}^{\mathrm{2}} }{\mathrm{4}}−\frac{\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} }{\mathrm{4}}+\mathrm{2b}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} \\ $$$$=\mathrm{4b}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} −\frac{\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} }{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{64}}\Leftrightarrow \\ $$$$\mathrm{a}^{\mathrm{4}} +\mathrm{b}^{\mathrm{4}} +\mathrm{c}^{\mathrm{4}} −\mathrm{2}\left(\mathrm{a}^{\mathrm{2}} \mathrm{b}^{\mathrm{2}} +\mathrm{a}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} \right)+\frac{\mathrm{a}^{\mathrm{2}} }{\mathrm{4}}=\mathrm{0}\left(\mathrm{1}\right) \\ $$$$\mathrm{Somilarly},\mathrm{we}\:\mathrm{have} \\ $$$${b}\:−\:\sqrt{{c}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{25}}}\:=\:\sqrt{{a}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{25}}\:}\Leftrightarrow \\ $$$$\mathrm{a}^{\mathrm{4}} +\mathrm{b}^{\mathrm{4}} +\mathrm{c}^{\mathrm{4}} −\mathrm{2}\left(\mathrm{a}^{\mathrm{2}} \mathrm{b}^{\mathrm{2}} +\mathrm{a}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} \right)+\frac{\mathrm{4b}^{\mathrm{2}} }{\mathrm{25}}=\mathrm{0}\left(\mathrm{2}\right) \\ $$$${c}\:−\:\sqrt{{a}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{36}}\:}\:=\:\sqrt{{b}^{\mathrm{2}} \:−\:\frac{\mathrm{1}}{\mathrm{36}}}\:\Leftrightarrow \\ $$$$\mathrm{a}^{\mathrm{4}} +\mathrm{b}^{\mathrm{4}} +\mathrm{c}^{\mathrm{4}} −\mathrm{2}\left(\mathrm{a}^{\mathrm{2}} \mathrm{b}^{\mathrm{2}} +\mathrm{a}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} \right)+\frac{\mathrm{4c}^{\mathrm{2}} }{\mathrm{36}}=\mathrm{0}\left(\mathrm{3}\right) \\ $$$$\mathrm{Substracting}\left(\mathrm{1}\right)\:\mathrm{from}\left(\mathrm{2}\right),\left(\mathrm{3}\right)\:\mathrm{we}\:\mathrm{get} \\ $$$$\begin{cases}{\frac{\mathrm{a}^{\mathrm{2}} }{\mathrm{4}}−\frac{\mathrm{4b}^{\mathrm{2}} }{\mathrm{25}}=\mathrm{0}}\\{\frac{\mathrm{a}^{\mathrm{2}} }{\mathrm{4}}−\frac{\mathrm{c}^{\mathrm{2}} }{\mathrm{9}}=\mathrm{0}}\end{cases}\Leftrightarrow\begin{cases}{\mathrm{b}^{\mathrm{2}} =\frac{\mathrm{25a}^{\mathrm{2}} }{\mathrm{16}}}\\{\mathrm{c}^{\mathrm{2}} =\frac{\mathrm{9a}^{\mathrm{2}} }{\mathrm{4}}}\end{cases}\Rightarrow\begin{cases}{\mathrm{b}=\frac{\mathrm{5a}}{\mathrm{4}}}\\{\mathrm{c}=\frac{\mathrm{3a}}{\mathrm{2}}}\end{cases} \\ $$$$\mathrm{Replace}\:\mathrm{into}\:\left(\mathrm{3}\right)\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{a}^{\mathrm{4}} +\frac{\mathrm{625a}^{\mathrm{2}} }{\mathrm{256}}+\frac{\mathrm{81a}^{\mathrm{4}} }{\mathrm{16}}−\mathrm{2}\left(\frac{\mathrm{25a}^{\mathrm{4}} }{\mathrm{16}}+\frac{\mathrm{9a}^{\mathrm{4}} }{\mathrm{4}}+\frac{\mathrm{225a}^{\mathrm{4}} }{\mathrm{64}}\right)+\frac{\mathrm{a}^{\mathrm{2}} }{\mathrm{4}}=\mathrm{0} \\ $$$$\Leftrightarrow−\frac{\mathrm{1575}}{\mathrm{256}}\mathrm{a}^{\mathrm{4}} +\frac{\mathrm{a}^{\mathrm{2}} }{\mathrm{4}}=\mathrm{0}\Leftrightarrow\mathrm{1575a}^{\mathrm{2}} =\mathrm{64} \\ $$$$\Rightarrow\mathrm{a}=\frac{\mathrm{8}}{\mathrm{15}\sqrt{\mathrm{7}}},\mathrm{b}=\frac{\mathrm{10}}{\:\mathrm{15}\sqrt{\mathrm{7}}},\mathrm{c}=\frac{\mathrm{12}}{\:\mathrm{15}\sqrt{\mathrm{7}}} \\ $$$$\mathrm{a}+\mathrm{b}+\mathrm{c}=\frac{\mathrm{8}+\mathrm{10}+\mathrm{12}}{\mathrm{15}\sqrt{\mathrm{7}}}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{7}}}=\frac{\mathrm{x}}{\:\sqrt{\mathrm{y}}} \\ $$$$\Rightarrow\mathrm{y}=\mathrm{7},\mathrm{x}=\mathrm{2}\Rightarrow\left(\mathrm{x}+\mathrm{y}\right)!=\mathrm{9}!=\mathrm{362880} \\ $$

Commented by abdul88 last updated on 23/Oct/20

Thank You Sir...:)

$$ \\ $$$$\left.{Thank}\:{You}\:{Sir}...:\right) \\ $$

Commented by 1549442205PVT last updated on 25/Oct/20

You are welcome

$$\mathrm{You}\:\mathrm{are}\:\mathrm{welcome} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com