Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 217931 by CrispyXYZ last updated on 23/Mar/25

Given a regular triangle ABC. AG=2GC. CK=2KB. AK∩BG=M. Prove that CM⊥AK.

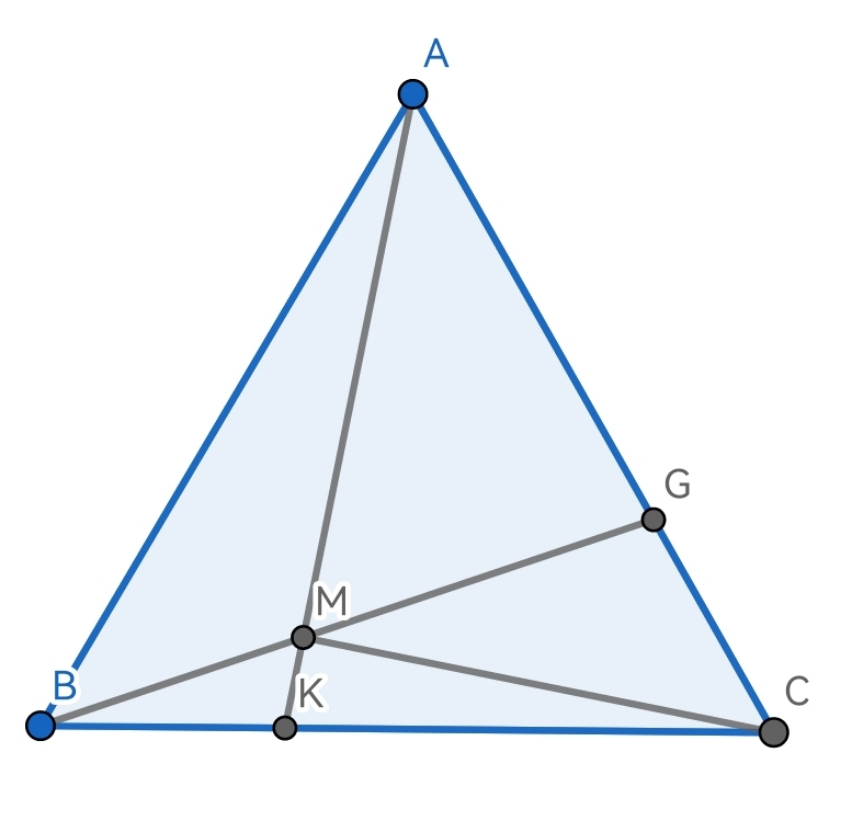
$$\mathrm{Given}\:\mathrm{a}\:\mathrm{regular}\:\mathrm{triangle}\:{ABC}. \\ $$$${AG}=\mathrm{2}{GC}.\:{CK}=\mathrm{2}{KB}.\:{AK}\cap{BG}={M}. \\ $$$$\mathrm{Prove}\:\mathrm{that}\:{CM}\bot{AK}. \\ $$

Commented by CrispyXYZ last updated on 23/Mar/25

Answered by mr W last updated on 23/Mar/25

Commented by mr W last updated on 23/Mar/25

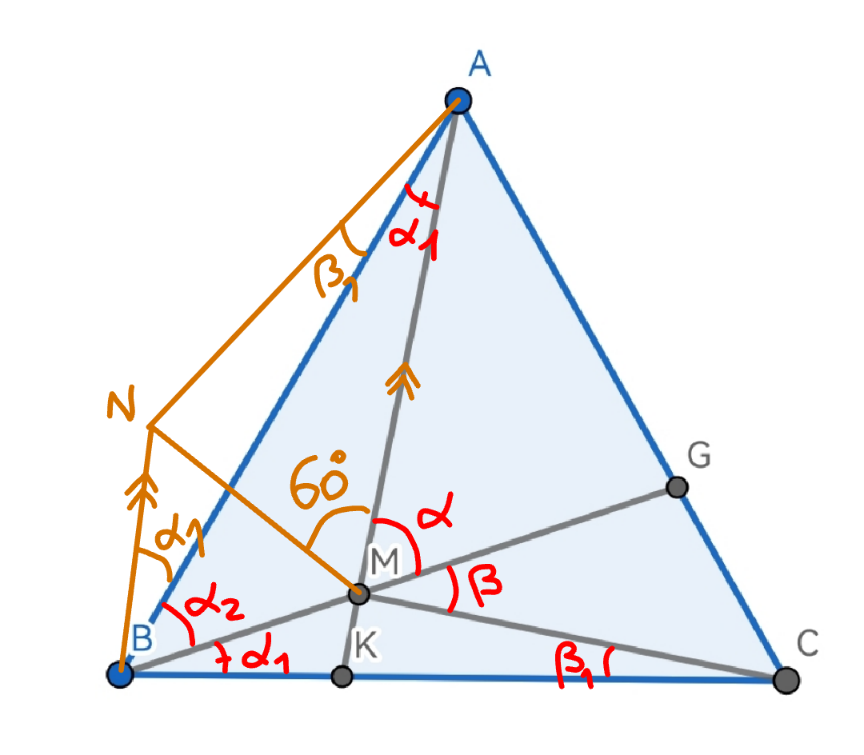
((CG)/(GA))×((AM)/(MK))×((KB)/(BC))=1 (1/2)×((AM)/(MK))×(1/3)=1 ⇒((AM)/(MK))=(6/1) ⇒AM=((6×AK)/7) ((CK)/(KB))×((BM)/(MG))×((GA)/(AC))=1 (2/1)×((BM)/(MG))×(2/3)=1 ⇒((BM)/(MG))=(3/4) ⇒BM=((3×BG)/7) since BG=AK, ⇒BM=((AM)/2) α=α_1 +α_2 β=α_1 +β_1 make ΔABN≡ΔBCM ∠MBN=α_1 +α_2 =60° ΔBMN is equilateral. BN//MA ∠AMN=60° MN=BM=((AM)/2) ⇒∠MAN=α_1 +β_1 =30° ∠AMC=α+β=α_1 +α_2 +α_1 +β_1 =60°+α_1 +β_1 =60°+30°=90° ⇒CM⊥AK

$$\frac{{CG}}{{GA}}×\frac{{AM}}{{MK}}×\frac{{KB}}{{BC}}=\mathrm{1} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}×\frac{{AM}}{{MK}}×\frac{\mathrm{1}}{\mathrm{3}}=\mathrm{1} \\ $$$$\Rightarrow\frac{{AM}}{{MK}}=\frac{\mathrm{6}}{\mathrm{1}} \\ $$$$\Rightarrow{AM}=\frac{\mathrm{6}×{AK}}{\mathrm{7}} \\ $$$$\frac{{CK}}{{KB}}×\frac{{BM}}{{MG}}×\frac{{GA}}{{AC}}=\mathrm{1} \\ $$$$\frac{\mathrm{2}}{\mathrm{1}}×\frac{{BM}}{{MG}}×\frac{\mathrm{2}}{\mathrm{3}}=\mathrm{1} \\ $$$$\Rightarrow\frac{{BM}}{{MG}}=\frac{\mathrm{3}}{\mathrm{4}} \\ $$$$\Rightarrow{BM}=\frac{\mathrm{3}×{BG}}{\mathrm{7}} \\ $$$${since}\:{BG}={AK}, \\ $$$$\Rightarrow{BM}=\frac{{AM}}{\mathrm{2}} \\ $$$$ \\ $$$$\alpha=\alpha_{\mathrm{1}} +\alpha_{\mathrm{2}} \\ $$$$\beta=\alpha_{\mathrm{1}} +\beta_{\mathrm{1}} \\ $$$${make}\:\Delta{ABN}\equiv\Delta{BCM} \\ $$$$\angle{MBN}=\alpha_{\mathrm{1}} +\alpha_{\mathrm{2}} =\mathrm{60}° \\ $$$$\Delta{BMN}\:{is}\:{equilateral}. \\ $$$${BN}//{MA} \\ $$$$\angle{AMN}=\mathrm{60}° \\ $$$${MN}={BM}=\frac{{AM}}{\mathrm{2}} \\ $$$$\Rightarrow\angle{MAN}=\alpha_{\mathrm{1}} +\beta_{\mathrm{1}} =\mathrm{30}° \\ $$$$\angle{AMC}=\alpha+\beta=\alpha_{\mathrm{1}} +\alpha_{\mathrm{2}} +\alpha_{\mathrm{1}} +\beta_{\mathrm{1}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{60}°+\alpha_{\mathrm{1}} +\beta_{\mathrm{1}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{60}°+\mathrm{30}°=\mathrm{90}° \\ $$$$\Rightarrow{CM}\bot{AK} \\ $$

Answered by A5T last updated on 23/Mar/25

Commented by A5T last updated on 23/Mar/25

WLOG, let AB=BC=CA=3⇒BK=1 ((AD)/(DB))×((BK)/(KC))×((CG)/(GA))=1⇒((AD)/(DB))=4⇒AD=((12)/5) AK=(√(BK^2 +AB^2 −2BK×ABcos60))=(√7) ((AM)/(MK))=((AD)/(DB))+((AG)/(GC))=4+2=6⇒AM=((6(√7))/( 7)) CD=(√(AD^2 +AC^2 −2AD×ACcos60))=(√((189)/(25))) ((CM)/(MD))=((CG)/(GA))+((CK)/(CB))=(5/2)⇒DM=((2(√(189)))/( 7×5)) AM^2 +DM^2 =((4×27)/(7×5^2 ))+((36)/7)=((144)/(25))=AD^2 ⇒∠AMD=90°⇒∠AMC=90°⇒CM⊥AK

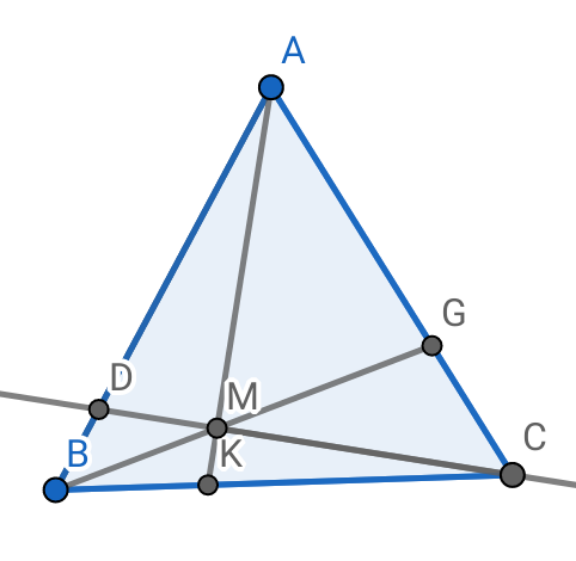
$$\mathrm{WLOG},\:\mathrm{let}\:\mathrm{AB}=\mathrm{BC}=\mathrm{CA}=\mathrm{3}\Rightarrow\mathrm{BK}=\mathrm{1} \\ $$$$\frac{\mathrm{AD}}{\mathrm{DB}}×\frac{\mathrm{BK}}{\mathrm{KC}}×\frac{\mathrm{CG}}{\mathrm{GA}}=\mathrm{1}\Rightarrow\frac{\mathrm{AD}}{\mathrm{DB}}=\mathrm{4}\Rightarrow\mathrm{AD}=\frac{\mathrm{12}}{\mathrm{5}} \\ $$$$\mathrm{AK}=\sqrt{\mathrm{BK}^{\mathrm{2}} +\mathrm{AB}^{\mathrm{2}} −\mathrm{2BK}×\mathrm{ABcos60}}=\sqrt{\mathrm{7}} \\ $$$$\frac{\mathrm{AM}}{\mathrm{MK}}=\frac{\mathrm{AD}}{\mathrm{DB}}+\frac{\mathrm{AG}}{\mathrm{GC}}=\mathrm{4}+\mathrm{2}=\mathrm{6}\Rightarrow\mathrm{AM}=\frac{\mathrm{6}\sqrt{\mathrm{7}}}{\:\mathrm{7}} \\ $$$$\mathrm{CD}=\sqrt{\mathrm{AD}^{\mathrm{2}} +\mathrm{AC}^{\mathrm{2}} −\mathrm{2AD}×\mathrm{ACcos60}}=\sqrt{\frac{\mathrm{189}}{\mathrm{25}}} \\ $$$$\frac{\mathrm{CM}}{\mathrm{MD}}=\frac{\mathrm{CG}}{\mathrm{GA}}+\frac{\mathrm{CK}}{\mathrm{CB}}=\frac{\mathrm{5}}{\mathrm{2}}\Rightarrow\mathrm{DM}=\frac{\mathrm{2}\sqrt{\mathrm{189}}}{\:\mathrm{7}×\mathrm{5}} \\ $$$$\mathrm{AM}^{\mathrm{2}} +\mathrm{DM}^{\mathrm{2}} =\frac{\mathrm{4}×\mathrm{27}}{\mathrm{7}×\mathrm{5}^{\mathrm{2}} }+\frac{\mathrm{36}}{\mathrm{7}}=\frac{\mathrm{144}}{\mathrm{25}}=\mathrm{AD}^{\mathrm{2}} \\ $$$$\Rightarrow\angle\mathrm{AMD}=\mathrm{90}°\Rightarrow\angle\mathrm{AMC}=\mathrm{90}°\Rightarrow\mathrm{CM}\bot\mathrm{AK} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com