GeometryQuestion and Answers: Page 94
Question Number 50460 Answers: 2 Comments: 2
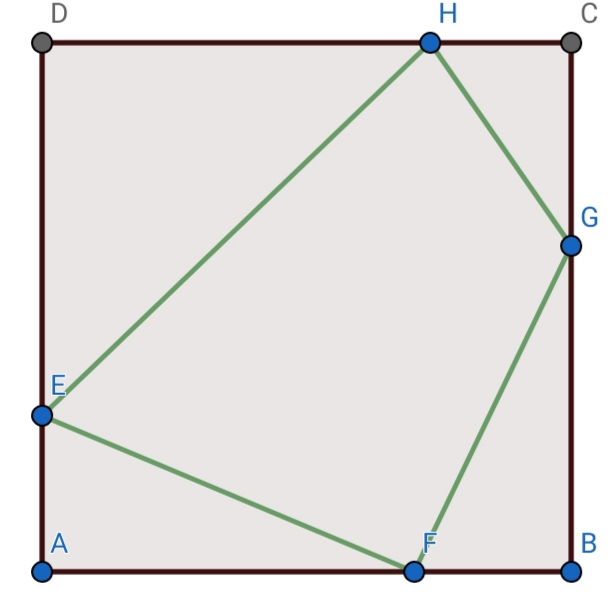
Question Number 50352 Answers: 1 Comments: 5
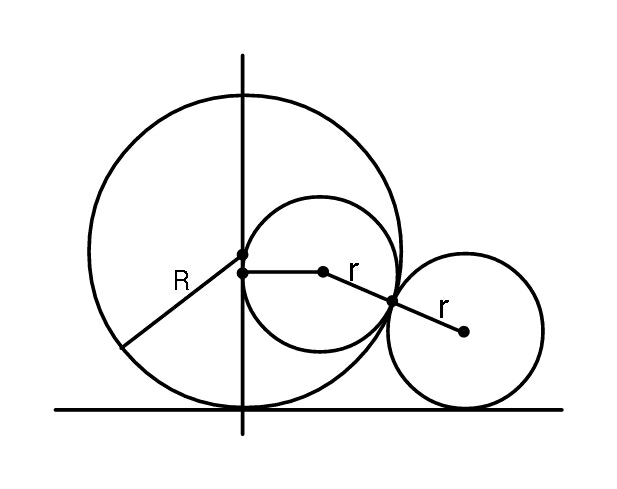
Question Number 49987 Answers: 1 Comments: 4
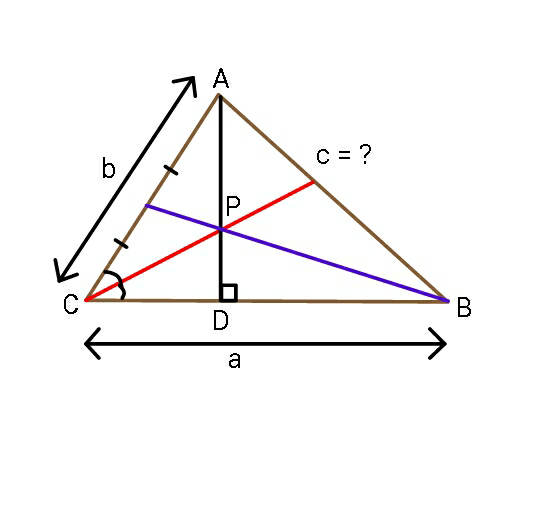
Question Number 49984 Answers: 1 Comments: 1

Question Number 49970 Answers: 2 Comments: 1
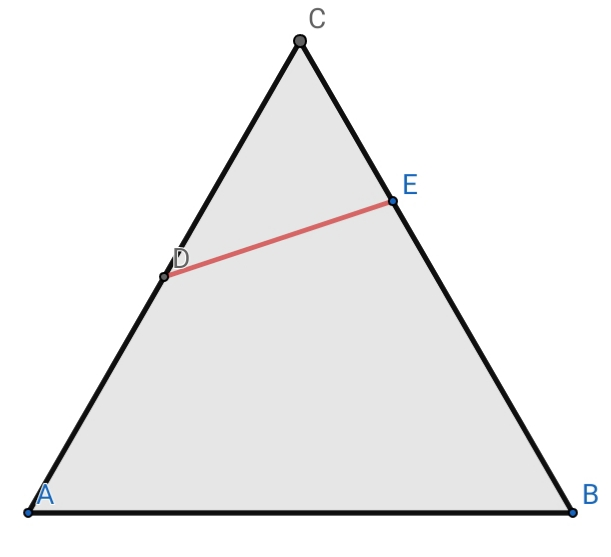
Question Number 49830 Answers: 3 Comments: 1
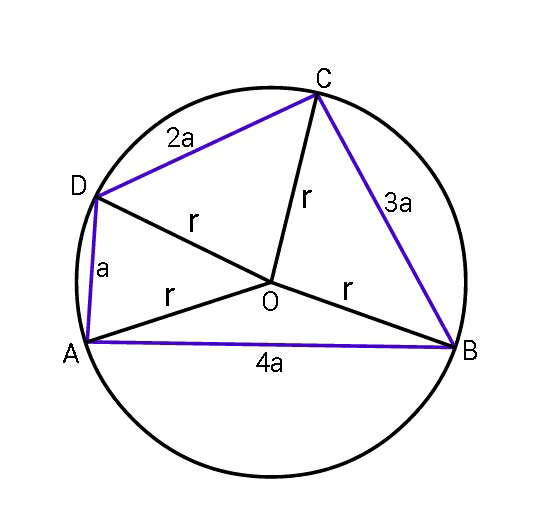
Question Number 49736 Answers: 1 Comments: 1
Question Number 49731 Answers: 1 Comments: 0
Question Number 49730 Answers: 0 Comments: 1
Question Number 49725 Answers: 2 Comments: 1

Question Number 49696 Answers: 1 Comments: 1

Question Number 49748 Answers: 1 Comments: 0
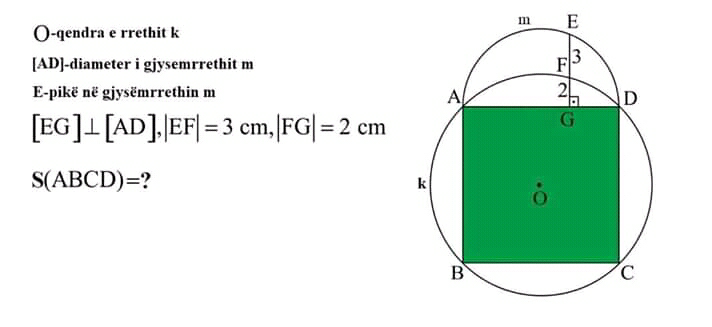
Question Number 51422 Answers: 2 Comments: 1

Question Number 49536 Answers: 3 Comments: 2
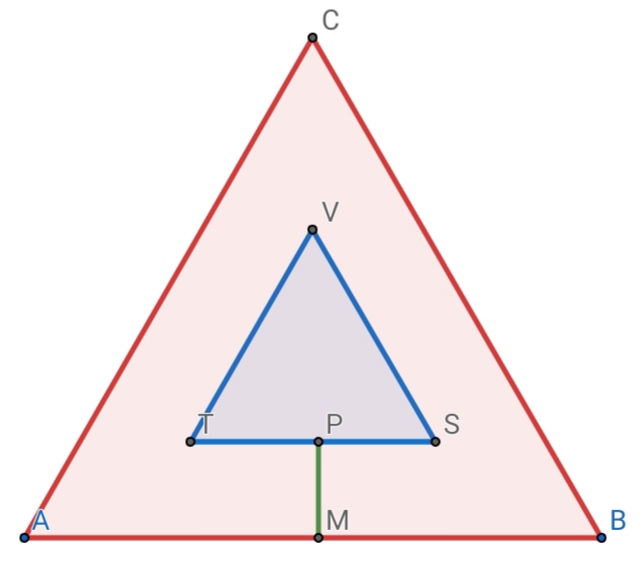
Question Number 49530 Answers: 3 Comments: 3

Question Number 49433 Answers: 3 Comments: 6

Question Number 49430 Answers: 0 Comments: 1

Question Number 49384 Answers: 1 Comments: 1

Question Number 49365 Answers: 2 Comments: 1

Question Number 49362 Answers: 1 Comments: 7

Question Number 49360 Answers: 0 Comments: 4

Question Number 49280 Answers: 1 Comments: 3

Question Number 49183 Answers: 1 Comments: 3

Question Number 49148 Answers: 1 Comments: 2
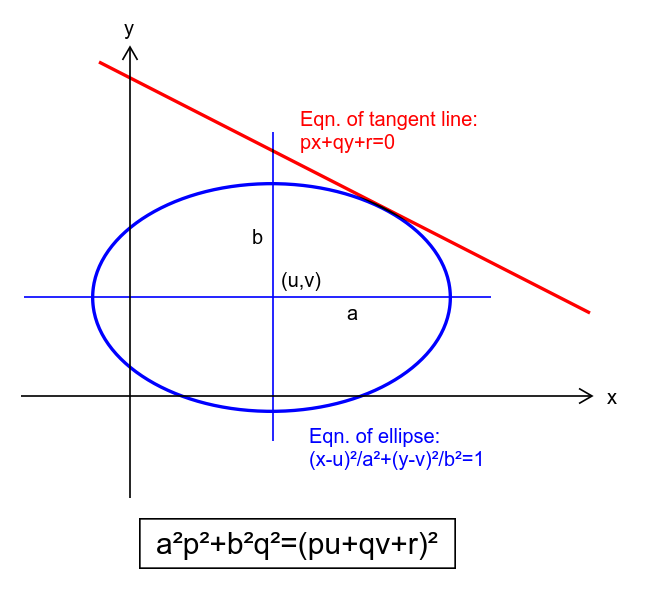
Question Number 49060 Answers: 2 Comments: 0
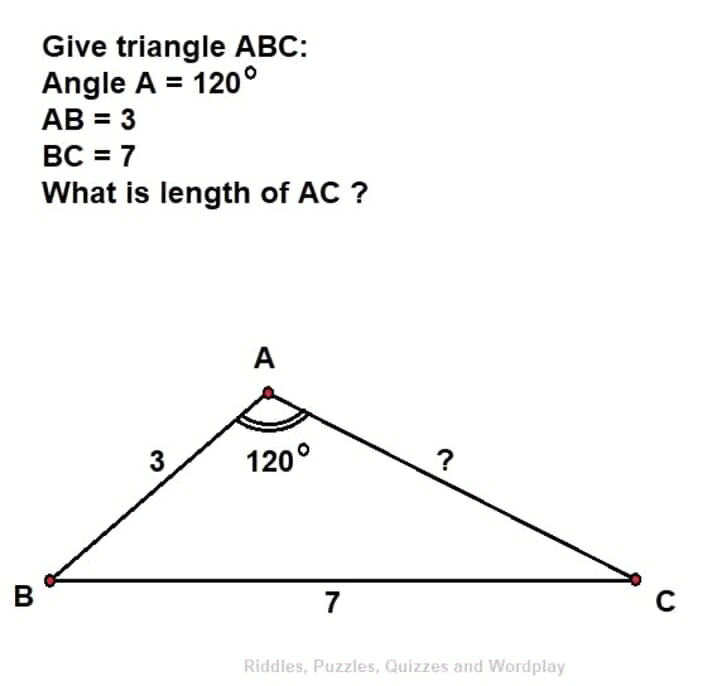
Question Number 48879 Answers: 0 Comments: 7