GeometryQuestion and Answers: Page 92
Question Number 53168 Answers: 2 Comments: 6

Question Number 53071 Answers: 0 Comments: 6

Question Number 53066 Answers: 1 Comments: 9

Question Number 53031 Answers: 1 Comments: 1
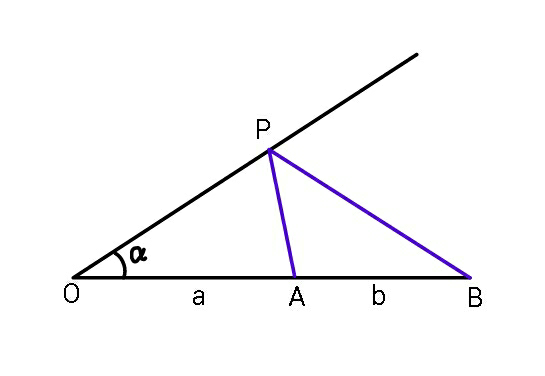
Question Number 52936 Answers: 0 Comments: 1
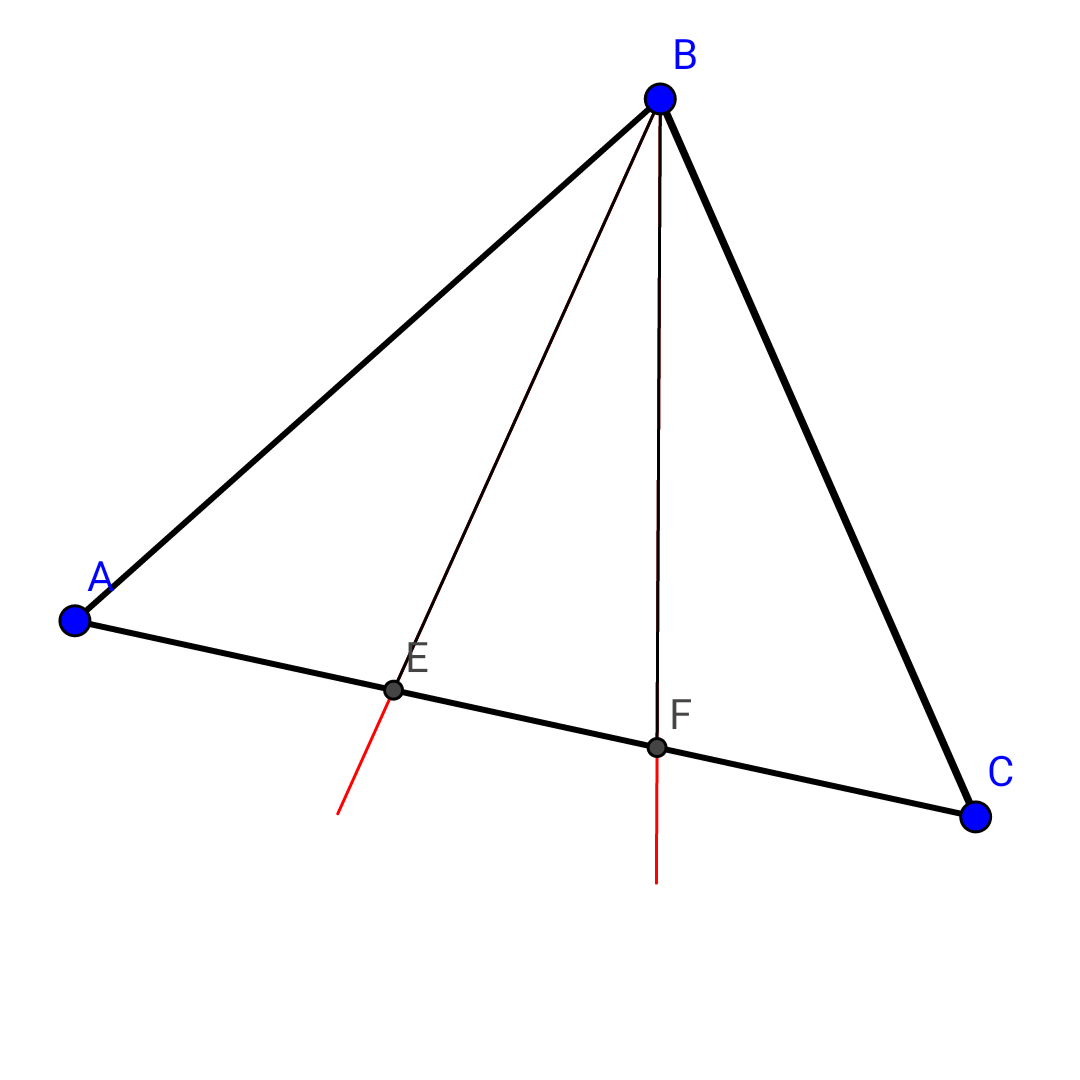
Question Number 52911 Answers: 2 Comments: 2

Question Number 52881 Answers: 1 Comments: 9

Question Number 52806 Answers: 1 Comments: 2

Question Number 52780 Answers: 1 Comments: 1

Question Number 52663 Answers: 2 Comments: 1

Question Number 52539 Answers: 2 Comments: 0

Question Number 52533 Answers: 0 Comments: 5

Question Number 52523 Answers: 0 Comments: 12

Question Number 52468 Answers: 2 Comments: 4
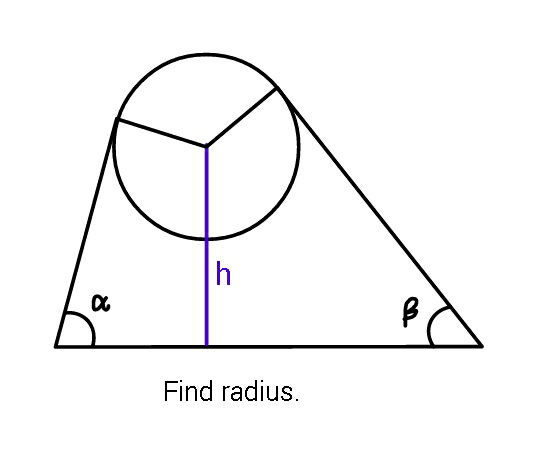
Question Number 52433 Answers: 0 Comments: 4

Question Number 52428 Answers: 0 Comments: 7
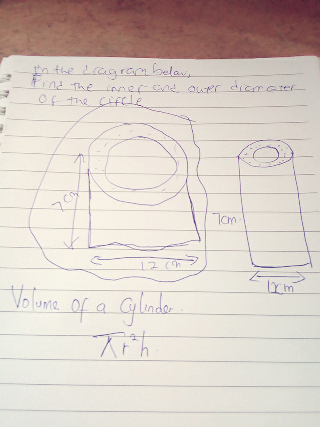
Question Number 52426 Answers: 2 Comments: 1

Question Number 52409 Answers: 1 Comments: 1

Question Number 52360 Answers: 1 Comments: 1

Question Number 52321 Answers: 0 Comments: 0
Question Number 52261 Answers: 0 Comments: 9
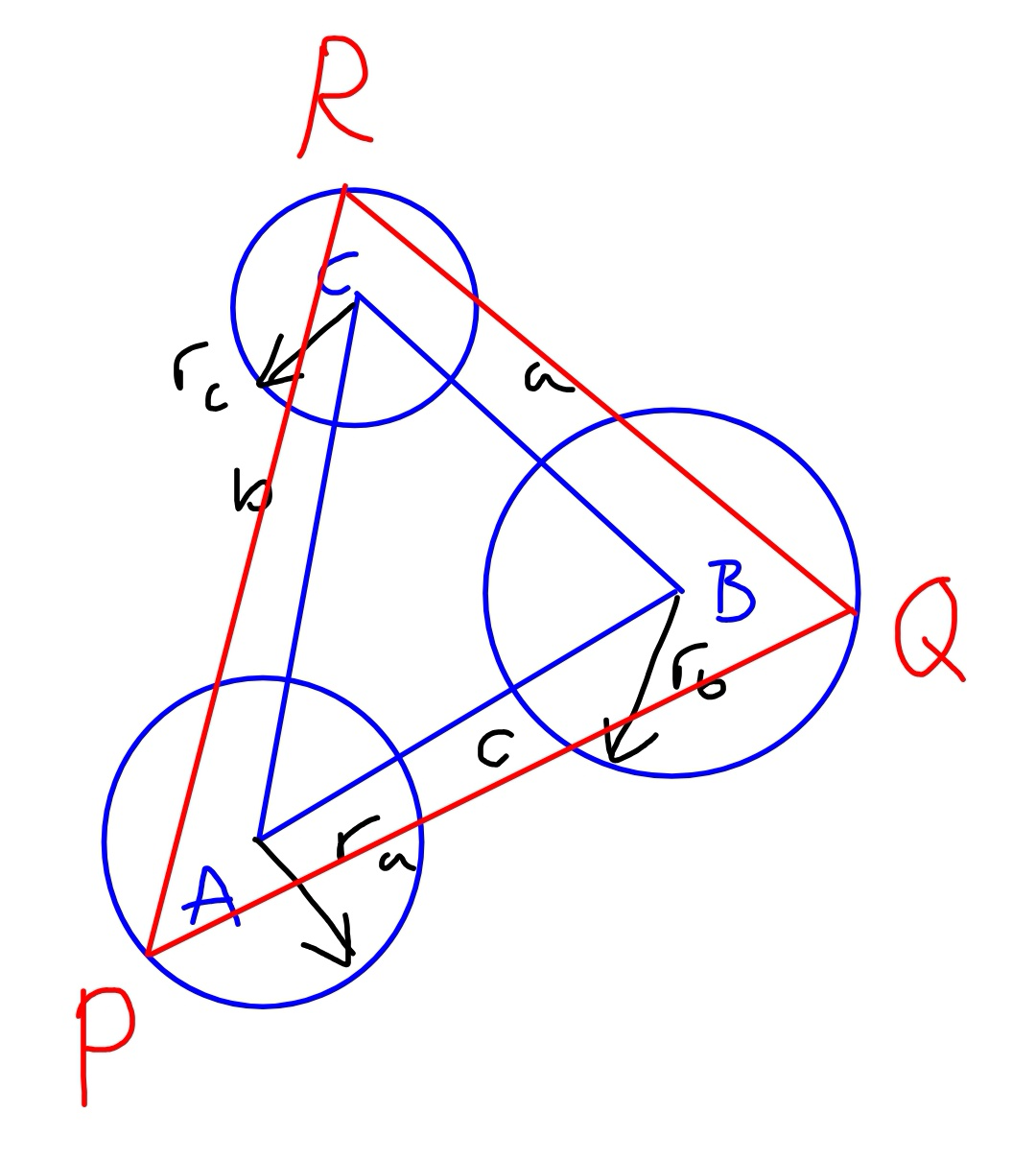
Question Number 52198 Answers: 2 Comments: 0

Question Number 52182 Answers: 0 Comments: 1
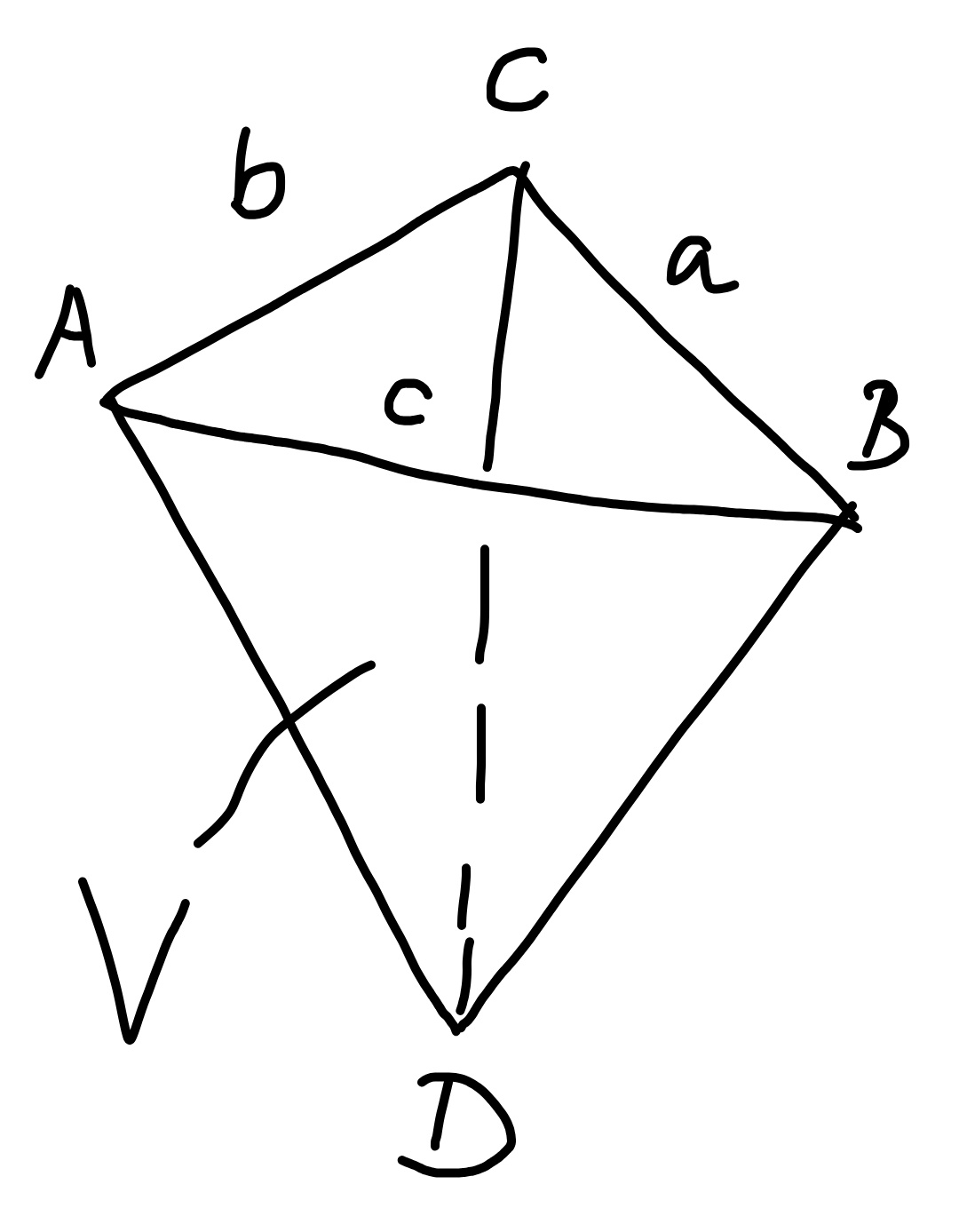
Question Number 52190 Answers: 2 Comments: 3

Question Number 52129 Answers: 1 Comments: 5

Question Number 52061 Answers: 2 Comments: 3