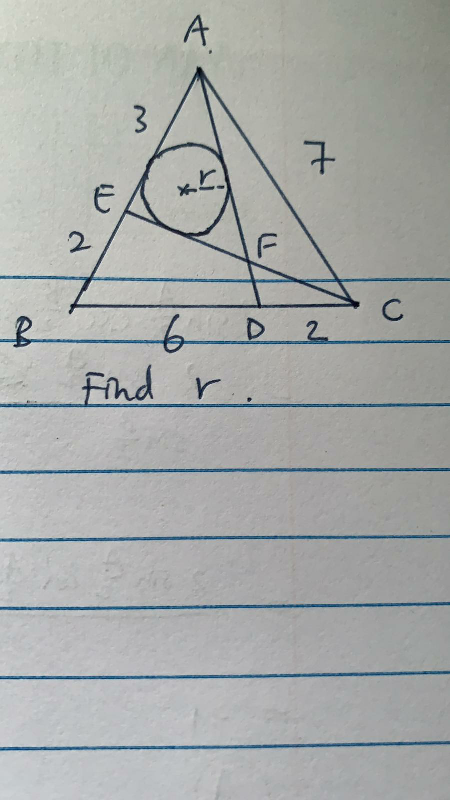GeometryQuestion and Answers: Page 88
Question Number 62380 Answers: 1 Comments: 0
Question Number 62363 Answers: 2 Comments: 0

Question Number 62124 Answers: 0 Comments: 0
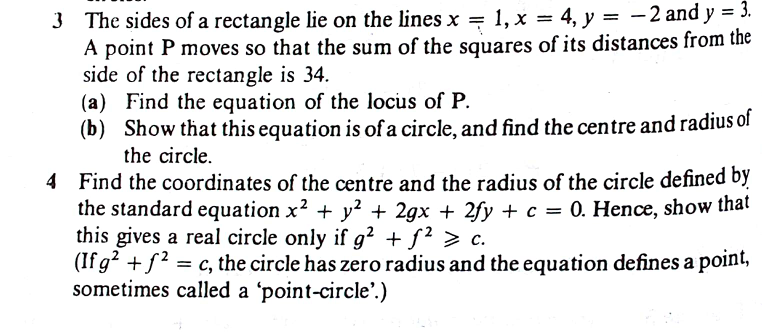
Question Number 62121 Answers: 2 Comments: 0
Question Number 62096 Answers: 0 Comments: 0

Question Number 62094 Answers: 0 Comments: 2

Question Number 61994 Answers: 1 Comments: 1

Question Number 61954 Answers: 0 Comments: 1

Question Number 61952 Answers: 1 Comments: 1

Question Number 61912 Answers: 0 Comments: 1
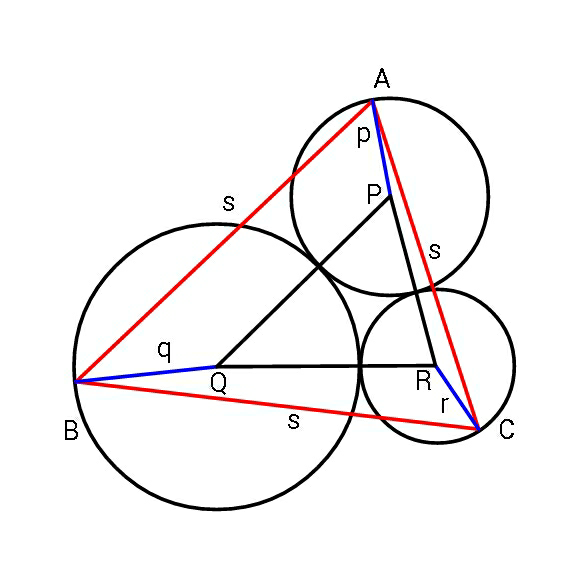
Question Number 61864 Answers: 1 Comments: 3

Question Number 61861 Answers: 3 Comments: 5

Question Number 61825 Answers: 1 Comments: 0

Question Number 61775 Answers: 0 Comments: 1

Question Number 61762 Answers: 2 Comments: 1

Question Number 61733 Answers: 0 Comments: 1
Question Number 61569 Answers: 1 Comments: 3

Question Number 61559 Answers: 2 Comments: 1

Question Number 61554 Answers: 1 Comments: 1
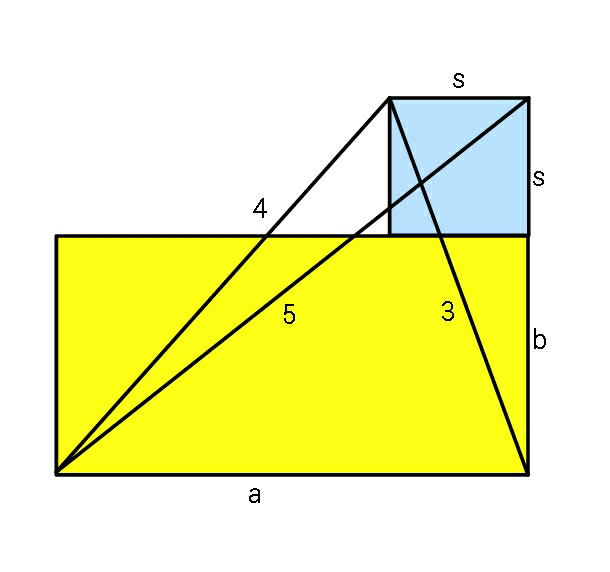
Question Number 61521 Answers: 1 Comments: 0

Question Number 61520 Answers: 0 Comments: 0

Question Number 61451 Answers: 0 Comments: 0

Question Number 61449 Answers: 1 Comments: 1

Question Number 61424 Answers: 1 Comments: 0

Question Number 61347 Answers: 2 Comments: 1
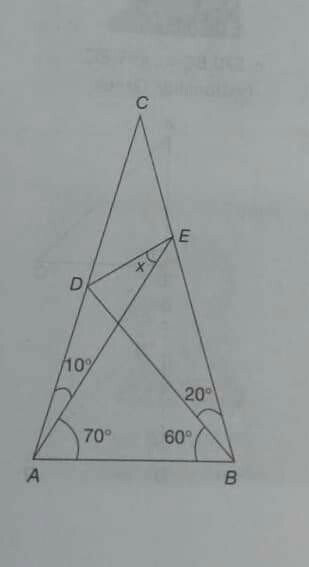
Question Number 61335 Answers: 1 Comments: 0