GeometryQuestion and Answers: Page 81
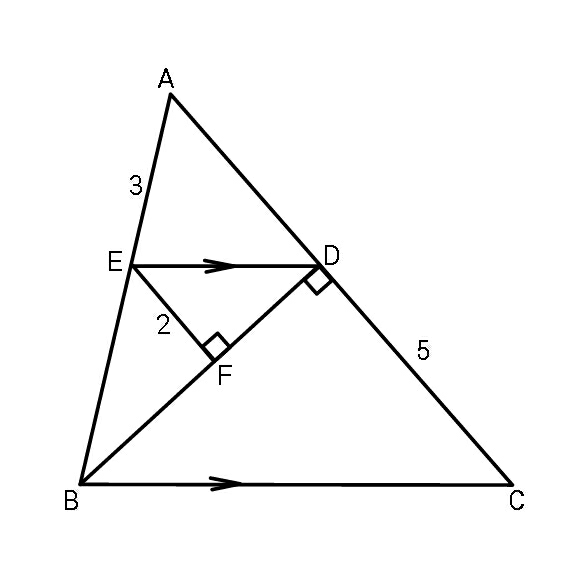
Question Number 72606 Answers: 2 Comments: 0
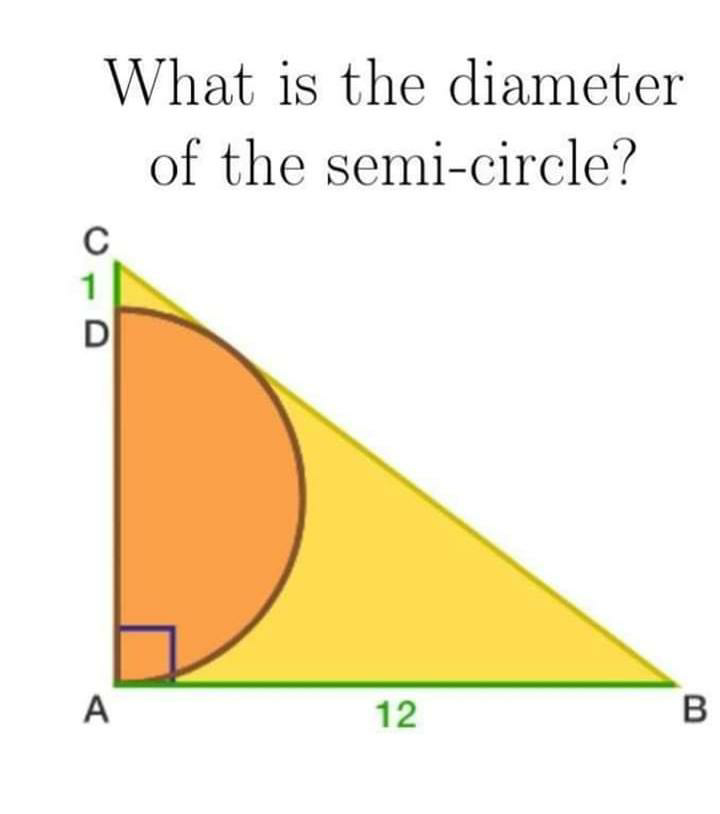
Question Number 72408 Answers: 1 Comments: 1

Question Number 72294 Answers: 1 Comments: 4

Question Number 72247 Answers: 1 Comments: 0

Question Number 72248 Answers: 1 Comments: 1

Question Number 72194 Answers: 2 Comments: 1
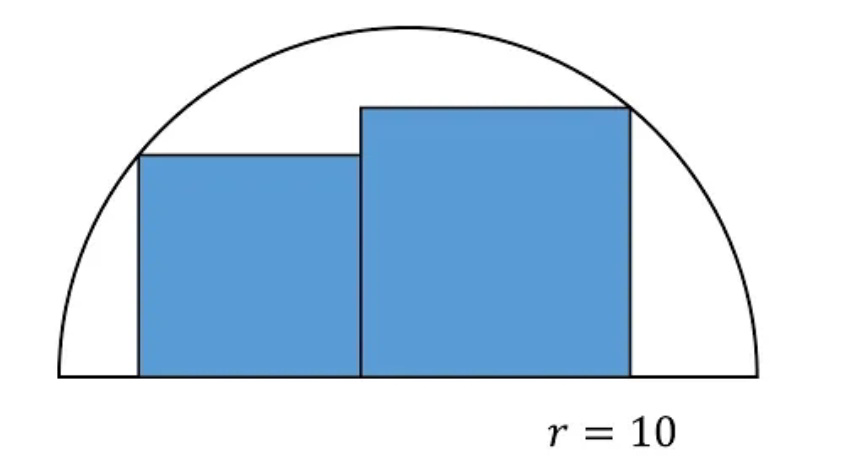
Question Number 71993 Answers: 1 Comments: 0

Question Number 71964 Answers: 5 Comments: 1
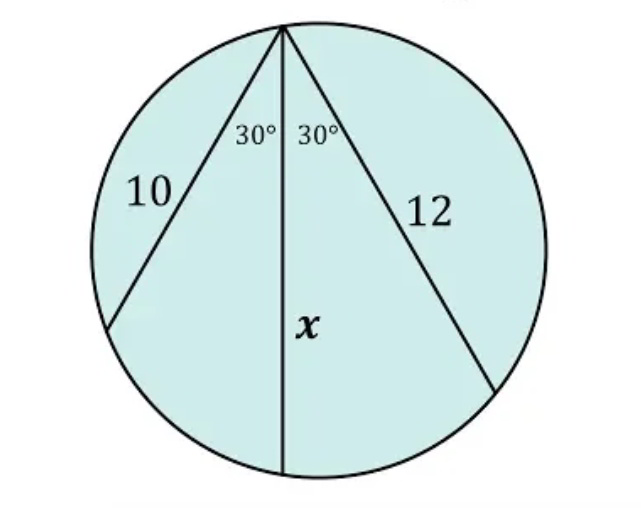
Question Number 71724 Answers: 0 Comments: 0

Question Number 71698 Answers: 1 Comments: 2

Question Number 71693 Answers: 1 Comments: 0

Question Number 71680 Answers: 1 Comments: 0

Question Number 71645 Answers: 1 Comments: 1

Question Number 71593 Answers: 1 Comments: 0
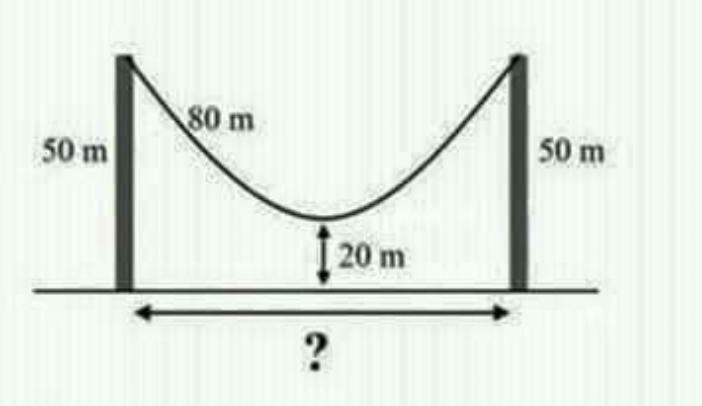
Question Number 71572 Answers: 2 Comments: 1

Question Number 71538 Answers: 2 Comments: 1

Question Number 71517 Answers: 1 Comments: 0
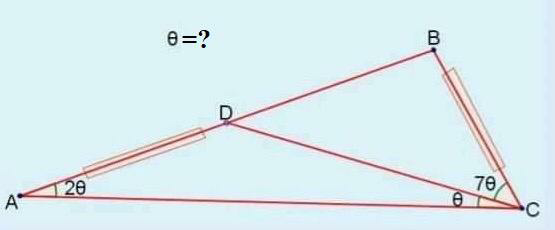
Question Number 71673 Answers: 1 Comments: 1

Question Number 71423 Answers: 0 Comments: 7

Question Number 71410 Answers: 1 Comments: 0
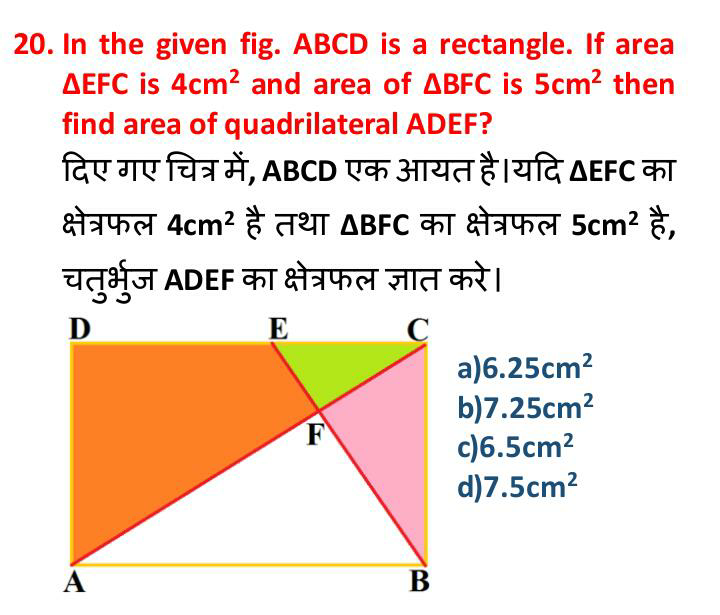
Question Number 71428 Answers: 1 Comments: 0

Question Number 71265 Answers: 0 Comments: 1

Question Number 71255 Answers: 1 Comments: 2
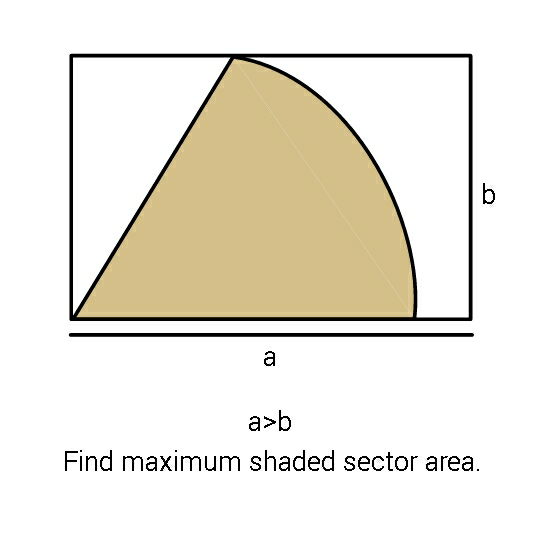
Question Number 71184 Answers: 1 Comments: 4

Question Number 71034 Answers: 0 Comments: 6

Question Number 70672 Answers: 1 Comments: 1