
GeometryQuestion and Answers: Page 78
Question Number 78609 Answers: 0 Comments: 0

Question Number 78542 Answers: 0 Comments: 0

Question Number 78511 Answers: 1 Comments: 2
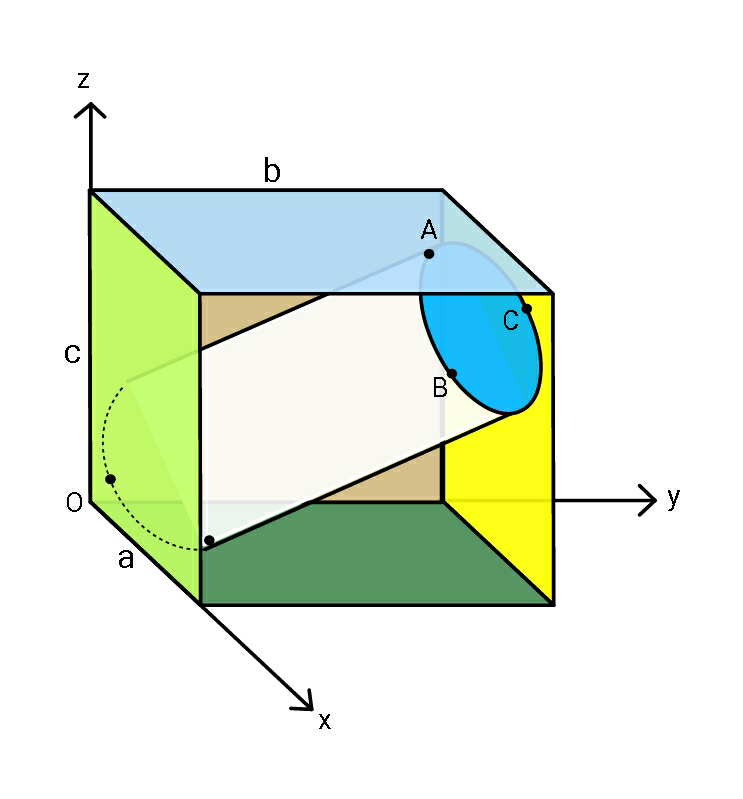
Question Number 78289 Answers: 1 Comments: 1
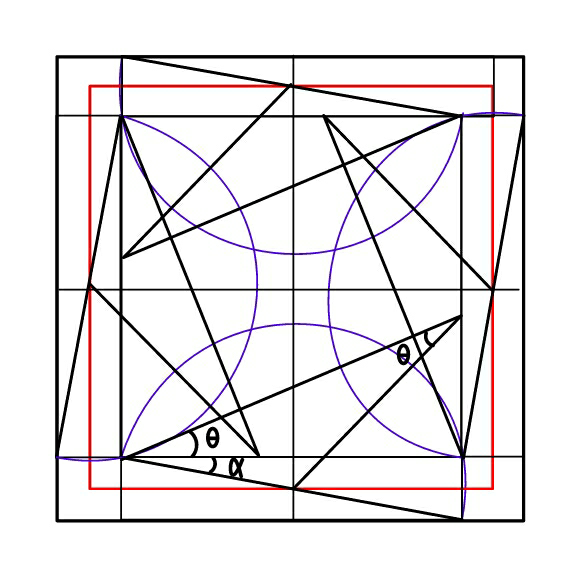
Question Number 78246 Answers: 0 Comments: 0
Question Number 78216 Answers: 1 Comments: 5
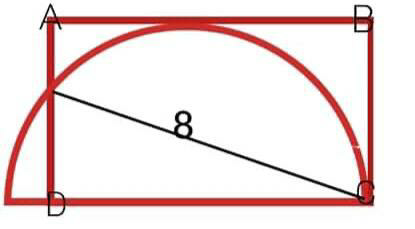
Question Number 78153 Answers: 1 Comments: 1

Question Number 78056 Answers: 1 Comments: 0

Question Number 78042 Answers: 1 Comments: 0

Question Number 77988 Answers: 1 Comments: 1

Question Number 77966 Answers: 1 Comments: 0

Question Number 77739 Answers: 1 Comments: 2

Question Number 77681 Answers: 1 Comments: 9
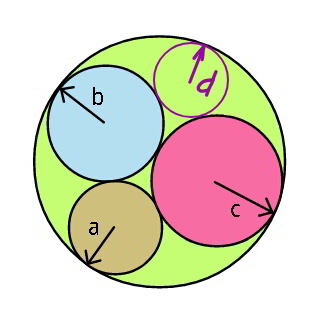
Question Number 77655 Answers: 2 Comments: 1
Question Number 77651 Answers: 1 Comments: 4

Question Number 77614 Answers: 1 Comments: 6

Question Number 77554 Answers: 0 Comments: 1

Question Number 77539 Answers: 0 Comments: 11

Question Number 77491 Answers: 0 Comments: 0

Question Number 77481 Answers: 1 Comments: 0

Question Number 77425 Answers: 1 Comments: 2

Question Number 77420 Answers: 0 Comments: 10

Question Number 77280 Answers: 0 Comments: 2

Question Number 77279 Answers: 0 Comments: 0

Question Number 77213 Answers: 0 Comments: 3

Question Number 77143 Answers: 1 Comments: 2

