
GeometryQuestion and Answers: Page 48
Question Number 173825 Answers: 0 Comments: 2

Question Number 173826 Answers: 0 Comments: 0
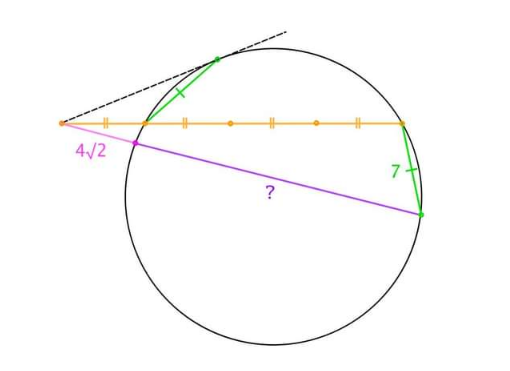
Question Number 173668 Answers: 3 Comments: 1
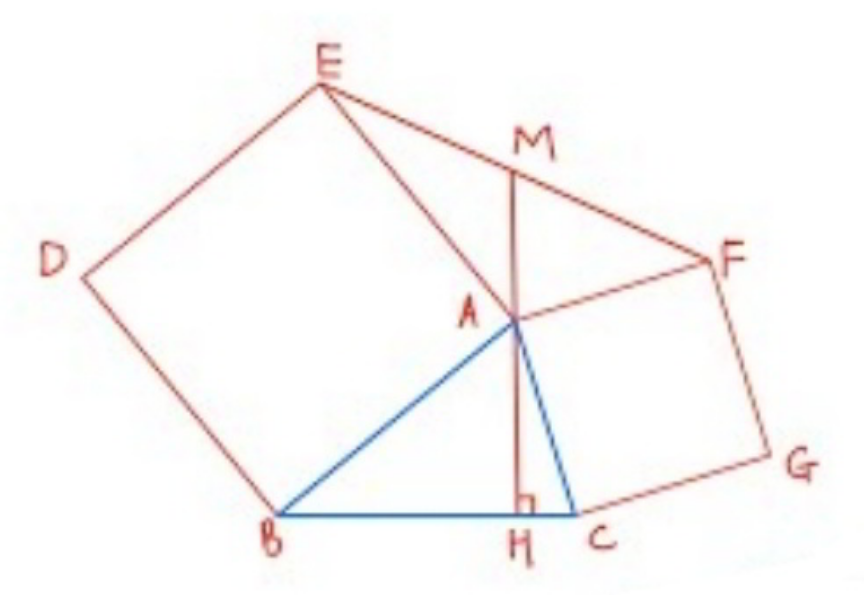
Question Number 173644 Answers: 1 Comments: 0

Question Number 173643 Answers: 0 Comments: 0
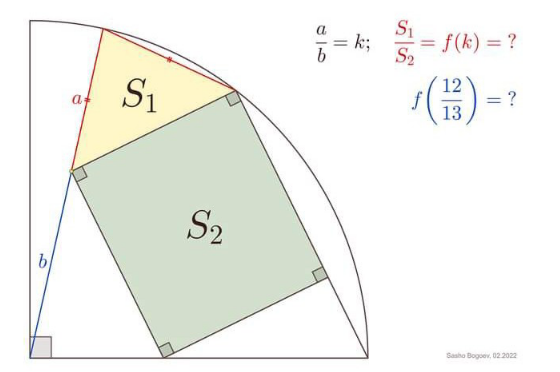
Question Number 181581 Answers: 1 Comments: 0

Question Number 173408 Answers: 1 Comments: 1
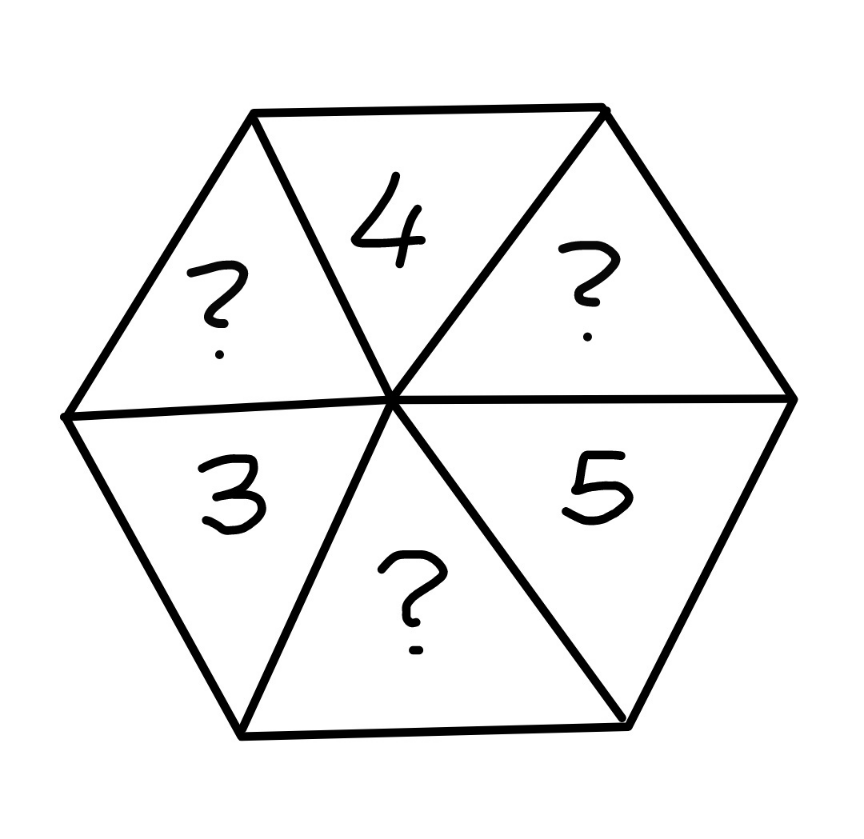
Question Number 173275 Answers: 2 Comments: 1

Question Number 173273 Answers: 1 Comments: 1

Question Number 173032 Answers: 1 Comments: 0

Question Number 173026 Answers: 1 Comments: 0

Question Number 173025 Answers: 1 Comments: 0

Question Number 172918 Answers: 0 Comments: 9

Question Number 172763 Answers: 2 Comments: 0
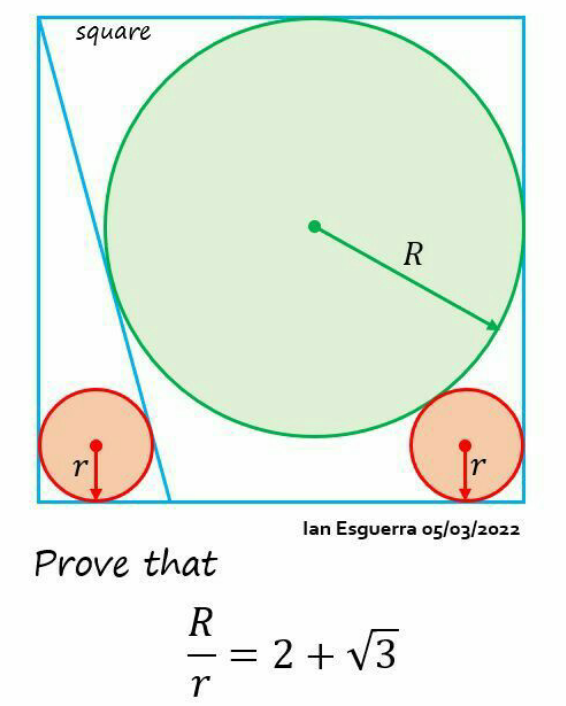
Question Number 172762 Answers: 1 Comments: 0

Question Number 172730 Answers: 1 Comments: 0

Question Number 172722 Answers: 0 Comments: 0

Question Number 172723 Answers: 1 Comments: 0

Question Number 172628 Answers: 1 Comments: 2

Question Number 172558 Answers: 1 Comments: 0

Question Number 181329 Answers: 1 Comments: 0

Question Number 172504 Answers: 2 Comments: 0

Question Number 172483 Answers: 1 Comments: 0

Question Number 172484 Answers: 1 Comments: 0

Question Number 172453 Answers: 1 Comments: 6
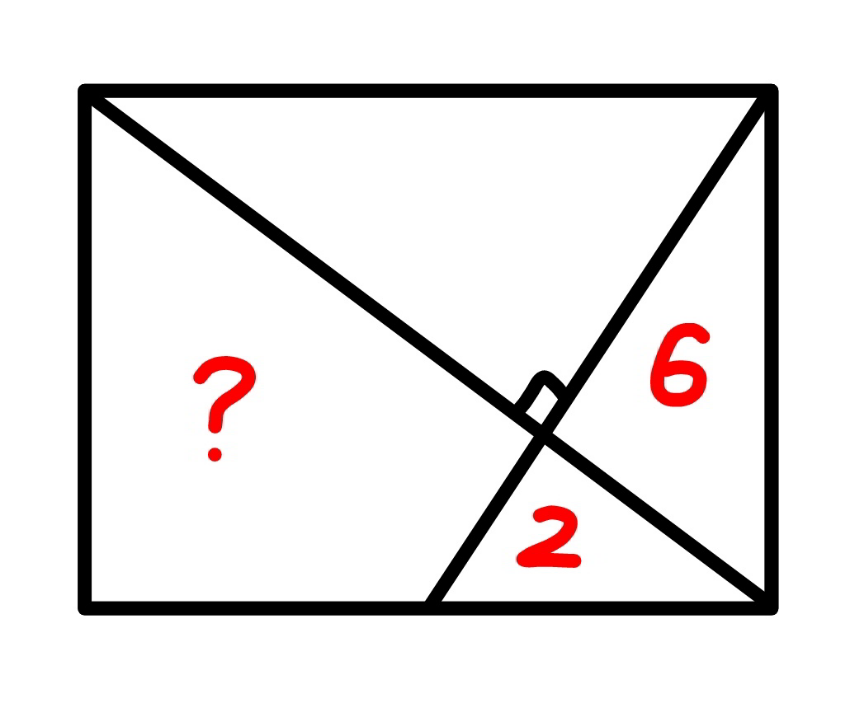
Question Number 172437 Answers: 1 Comments: 0

