
GeometryQuestion and Answers: Page 115
Question Number 11594 Answers: 1 Comments: 0
Question Number 11403 Answers: 0 Comments: 1
Question Number 11344 Answers: 0 Comments: 1
$${n}_{{c}_{{n}} } =\frac{{n}!}{\left({n}−{n}\right)!{n}!} \\ $$
Question Number 11343 Answers: 0 Comments: 1
Question Number 11221 Answers: 0 Comments: 4

Question Number 11148 Answers: 1 Comments: 0
$$\int\frac{{x}\:{dx}}{\sqrt[{\mathrm{3}}]{{x}+\mathrm{1}}}=....??? \\ $$
Question Number 11078 Answers: 1 Comments: 0
Question Number 10815 Answers: 0 Comments: 0
$${Evalute}\:\:\int\left({x}^{\mathrm{2}\:} \:\:+\:\mathrm{9}\right)^{\mathrm{9}} \:{dx}\:. \\ $$
Question Number 10829 Answers: 2 Comments: 0
Question Number 10760 Answers: 1 Comments: 1
Question Number 10667 Answers: 1 Comments: 0
Question Number 10639 Answers: 1 Comments: 3
Question Number 10620 Answers: 1 Comments: 1

Question Number 11183 Answers: 1 Comments: 0

Question Number 10513 Answers: 0 Comments: 1
$${e}^{\left(−\mathrm{2}×\mathrm{10}^{−\mathrm{2}} /\mathrm{2}\right)} \\ $$
Question Number 10480 Answers: 2 Comments: 0
$${d}/{dx}\left(\mathrm{cos}\:^{\mathrm{2}} {xdy}/{dx}\right)=\mathrm{0} \\ $$$$ \\ $$
Question Number 10477 Answers: 0 Comments: 0
Question Number 10435 Answers: 1 Comments: 1

Question Number 10426 Answers: 0 Comments: 0
Question Number 10415 Answers: 1 Comments: 0
Question Number 10303 Answers: 0 Comments: 1

Question Number 10222 Answers: 2 Comments: 1
Question Number 10200 Answers: 0 Comments: 0
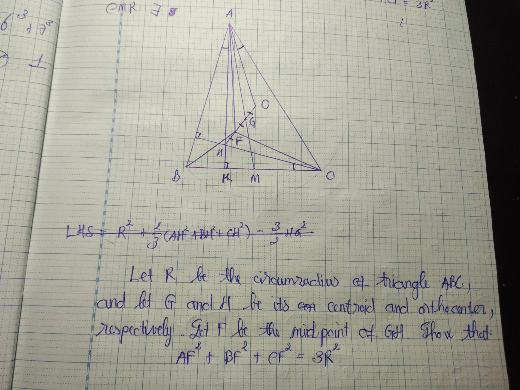
Question Number 10195 Answers: 1 Comments: 1
Question Number 10181 Answers: 0 Comments: 0
Question Number 10166 Answers: 1 Comments: 1

Pg 110 Pg 111 Pg 112 Pg 113 Pg 114 Pg 115 Pg 116 Pg 117 Pg 118 Pg 119
