Question and Answers Forum

GeometryQuestion and Answers: Page 114

Question Number 12534 Answers: 1 Comments: 0

please help me .How can resolve this system? {_((1+(√2))x+y=1) ^(x^2 +y^2 =1)

$${please}\:{help}\:{me}\:.{How}\:{can}\:{resolve}\:{this}\:{system}? \\ $$$$\left\{_{\left(\mathrm{1}+\sqrt{\mathrm{2}}\right){x}+{y}=\mathrm{1}} ^{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} =\mathrm{1}} \right. \\ $$

Question Number 12506 Answers: 2 Comments: 0

A wooden stick was broken randomly into three pieces. What is the probability that a triangle can be built from those three parts?

$$\mathrm{A}\:\mathrm{wooden}\:\mathrm{stick}\:\mathrm{was}\:\mathrm{broken}\:\mathrm{randomly}\:\mathrm{into} \\ $$$$\mathrm{three}\:\mathrm{pieces}.\:\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{probability}\:\mathrm{that}\:\mathrm{a}\:\mathrm{triangle} \\ $$$$\mathrm{can}\:\mathrm{be}\:\mathrm{built}\:\mathrm{from}\:\mathrm{those}\:\mathrm{three}\:\mathrm{parts}? \\ $$

Question Number 12490 Answers: 0 Comments: 1

328976/256

$$\mathrm{328976}/\mathrm{256} \\ $$

Question Number 12499 Answers: 1 Comments: 0

Water flows out of a tank through a hole of diameter 2cm above the hole (1) Determine the velocity of outflow (2) The rate of outflow when the level of the water in the tank is 2cm above the hole.

$$\mathrm{Water}\:\mathrm{flows}\:\mathrm{out}\:\mathrm{of}\:\mathrm{a}\:\mathrm{tank}\:\mathrm{through}\:\mathrm{a}\:\mathrm{hole}\:\mathrm{of}\:\mathrm{diameter}\:\mathrm{2cm}\:\mathrm{above}\:\mathrm{the}\:\mathrm{hole} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{Determine}\:\mathrm{the}\:\mathrm{velocity}\:\mathrm{of}\:\mathrm{outflow} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{The}\:\mathrm{rate}\:\mathrm{of}\:\mathrm{outflow}\:\mathrm{when}\:\mathrm{the}\:\mathrm{level}\:\mathrm{of}\:\mathrm{the}\:\mathrm{water}\:\mathrm{in}\:\mathrm{the}\:\mathrm{tank}\:\mathrm{is}\:\mathrm{2cm}\:\mathrm{above} \\ $$$$\mathrm{the}\:\mathrm{hole}.\: \\ $$

Question Number 12371 Answers: 0 Comments: 0

tank you

$${tank}\:{you} \\ $$$$ \\ $$

Question Number 12332 Answers: 2 Comments: 7

prove ; ((0/0))=2

$$\mathrm{prove}\:;\:\left(\frac{\mathrm{0}}{\mathrm{0}}\right)=\mathrm{2} \\ $$

Question Number 12267 Answers: 2 Comments: 0

Find the nth term of the sequence 1) (1/3) , (1/(15)) , (1/(35)) , (1/(63)) , (1/(99)) 2) (1/2), (1/6), (1/(12)), (1/(20)), (1/(30))

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{nth}\:\mathrm{term}\:\mathrm{of}\:\mathrm{the}\:\mathrm{sequence} \\ $$$$\left.\mathrm{1}\right)\:\:\:\frac{\mathrm{1}}{\mathrm{3}}\:,\:\frac{\mathrm{1}}{\mathrm{15}}\:,\:\frac{\mathrm{1}}{\mathrm{35}}\:,\:\frac{\mathrm{1}}{\mathrm{63}}\:,\:\frac{\mathrm{1}}{\mathrm{99}} \\ $$$$\left.\mathrm{2}\right)\:\:\:\:\frac{\mathrm{1}}{\mathrm{2}},\:\frac{\mathrm{1}}{\mathrm{6}},\:\frac{\mathrm{1}}{\mathrm{12}},\:\frac{\mathrm{1}}{\mathrm{20}},\:\frac{\mathrm{1}}{\mathrm{30}} \\ $$

Question Number 12265 Answers: 0 Comments: 2

Determinant method can be used to solve the system below?, if yes solve by determinant method and if no solve by another method x+y−z=8 2x+y−2z=3 (give clear reason for your answer)

$${Determinant}\:{method}\:{can}\:{be}\:{used}\:{to}\:{solve} \\ $$$${the}\:{system}\:{below}?,\:\mathrm{if}\:\mathrm{yes}\:\mathrm{solve}\:\mathrm{by}\:\mathrm{determinant}\:\mathrm{method}\:\mathrm{and} \\ $$$$\:\mathrm{if}\:\mathrm{no}\:\mathrm{solve}\:\mathrm{by}\:\mathrm{another}\:\mathrm{method} \\ $$$$\:\:\:\:\:\:\:\:\: \\ $$$${x}+{y}−{z}=\mathrm{8} \\ $$$$\mathrm{2}{x}+{y}−\mathrm{2}{z}=\mathrm{3} \\ $$$$\left({give}\:{clear}\:{reason}\:{for}\:{your}\:{answer}\right) \\ $$

Question Number 12148 Answers: 0 Comments: 13

Question Number 12131 Answers: 0 Comments: 0

a cube has a rib ABCD.EFGH, the midle point P on BF so that BP = PF, and the midle point Q on FG so that FQ = QG how long projection point C to APQH field ?

$${a}\:{cube}\:{has}\:{a}\:{rib}\:{ABCD}.{EFGH},\:{the}\:{midle}\:{point}\:{P}\:\:{on}\:{BF}\:{so}\:{that}\:{BP}\:=\:{PF}, \\ $$$${and}\:{the}\:{midle}\:{point}\:{Q}\:{on}\:{FG}\:{so}\:{that}\:{FQ}\:=\:{QG} \\ $$$${how}\:{long}\:{projection}\:{point}\:{C}\:{to}\:{APQH}\:{field}\:? \\ $$

Question Number 12045 Answers: 1 Comments: 0

how much matrices of integers number A= [(a,b),(c,d) ]if A^2 =I and b=c

$${how}\:{much}\:{matrices}\:{of}\:{integers}\:{number} \\ $$$${A}=\begin{bmatrix}{{a}}&{{b}}\\{{c}}&{{d}}\end{bmatrix}{if}\:{A}^{\mathrm{2}} ={I}\:{and}\:{b}={c} \\ $$

Question Number 12019 Answers: 1 Comments: 0

Question Number 12013 Answers: 1 Comments: 0

The slope of a curve is, 7x + 3 and it passes through the point (2, 4), Find the equation of the point

$$\mathrm{The}\:\mathrm{slope}\:\mathrm{of}\:\mathrm{a}\:\mathrm{curve}\:\mathrm{is},\:\mathrm{7x}\:+\:\mathrm{3}\:\:\mathrm{and}\:\mathrm{it}\:\mathrm{passes}\:\mathrm{through}\:\mathrm{the}\:\mathrm{point}\:\left(\mathrm{2},\:\mathrm{4}\right), \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{the}\:\mathrm{point} \\ $$

Question Number 12008 Answers: 1 Comments: 1

Question Number 11991 Answers: 0 Comments: 0

show that: ((2n)/(2n+1))=1−(1/(2n+1)) via power series, and other methods

$$\mathrm{show}\:\mathrm{that}: \\ $$$$\frac{\mathrm{2}{n}}{\mathrm{2}{n}+\mathrm{1}}=\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}{n}+\mathrm{1}} \\ $$$$\mathrm{via}\:\mathrm{power}\:\mathrm{series},\:\mathrm{and}\:\mathrm{other}\:\mathrm{methods} \\ $$

Question Number 11969 Answers: 2 Comments: 0

A gas occupies 30 dm^3 at s t p, what volume will it occupy at 91°C and 380 mmHg

$$\mathrm{A}\:\mathrm{gas}\:\mathrm{occupies}\:\mathrm{30}\:\mathrm{dm}^{\mathrm{3}} \:\mathrm{at}\:\mathrm{s}\:\mathrm{t}\:\mathrm{p},\:\:\mathrm{what}\:\mathrm{volume}\:\mathrm{will}\:\mathrm{it}\:\mathrm{occupy}\:\mathrm{at}\:\mathrm{91}°\mathrm{C}\: \\ $$$$\mathrm{and}\:\mathrm{380}\:\mathrm{mmHg} \\ $$

Question Number 11935 Answers: 3 Comments: 0

((7!)/(6!))+((8!)/(7!))+((9!)/(8!))+...((n!)/((n−1)!))=84 ⇒n=?

$$\frac{\mathrm{7}!}{\mathrm{6}!}+\frac{\mathrm{8}!}{\mathrm{7}!}+\frac{\mathrm{9}!}{\mathrm{8}!}+...\frac{\mathrm{n}!}{\left(\mathrm{n}−\mathrm{1}\right)!}=\mathrm{84}\:\Rightarrow\mathrm{n}=? \\ $$

Question Number 11915 Answers: 2 Comments: 2

Question Number 11880 Answers: 0 Comments: 0

S_(ABCD) =3+2(√2) ∠BAO=∠MAO=22,5° ∠BCM=∠DCM S_(AOB) =?

$$\boldsymbol{\mathrm{S}}_{\boldsymbol{\mathrm{ABCD}}} =\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}} \\ $$$$\angle\boldsymbol{\mathrm{BAO}}=\angle\boldsymbol{\mathrm{MAO}}=\mathrm{22},\mathrm{5}° \\ $$$$\angle\boldsymbol{\mathrm{BCM}}=\angle\boldsymbol{\mathrm{DCM}} \\ $$$$\boldsymbol{\mathrm{S}}_{\boldsymbol{\mathrm{AOB}}} =? \\ $$

Question Number 11867 Answers: 0 Comments: 1

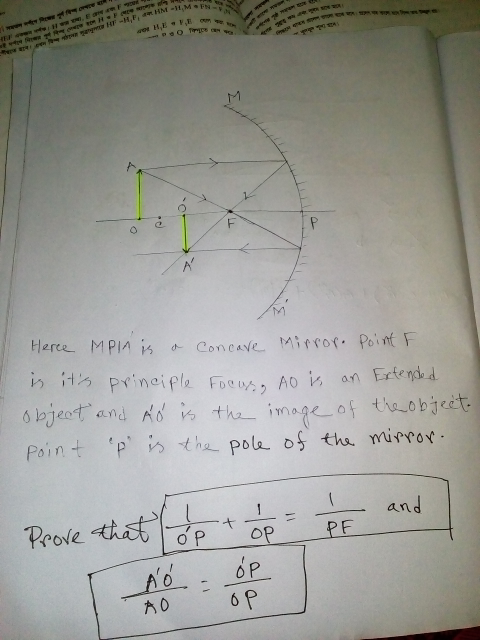
Question Number 11864 Answers: 2 Comments: 0

∫_7 ^(10) x^2 dx/x^2 −3x+2=

$$\underset{\mathrm{7}} {\overset{\mathrm{10}} {\int}}{x}^{\mathrm{2}} {dx}/{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}= \\ $$

Question Number 11827 Answers: 1 Comments: 0

∫x^2 dcosx=?

$$\int\mathrm{x}^{\mathrm{2}} \mathrm{dcosx}=? \\ $$

Question Number 11759 Answers: 1 Comments: 0

A quadrilateral with consecutive sides of lenght 7, 15, 15, and d is inscribed in a circle with its diameter d. Find the radius of circle

$$\mathrm{A}\:\mathrm{quadrilateral}\:\mathrm{with}\:\mathrm{consecutive}\:\mathrm{sides}\:\mathrm{of}\:\mathrm{lenght}\:\mathrm{7},\:\mathrm{15},\:\mathrm{15},\:\mathrm{and}\:{d} \\ $$$$\mathrm{is}\:\mathrm{inscribed}\:\mathrm{in}\:\mathrm{a}\:\mathrm{circle}\:\mathrm{with}\:\mathrm{its}\:\mathrm{diameter}\:{d}. \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{radius}\:\mathrm{of}\:\mathrm{circle} \\ $$

Question Number 11740 Answers: 0 Comments: 0

Metal pellets of mass 0.1 kg are fired into an iron plate of large mass m and specific heat capacity c at a temperature of 15°C . 25% of the kinetic energy of the pellets is converted thermal energy and the plate temperature rises to 16°C. The average speed of the pellets before hitting the plate was ?

$$\mathrm{Metal}\:\mathrm{pellets}\:\mathrm{of}\:\mathrm{mass}\:\mathrm{0}.\mathrm{1}\:\mathrm{kg}\:\mathrm{are}\:\mathrm{fired}\:\mathrm{into}\:\mathrm{an}\:\mathrm{iron}\:\mathrm{plate}\:\mathrm{of}\:\mathrm{large}\:\mathrm{mass}\:\mathrm{m} \\ $$$$\mathrm{and}\:\mathrm{specific}\:\mathrm{heat}\:\mathrm{capacity}\:\mathrm{c}\:\mathrm{at}\:\mathrm{a}\:\mathrm{temperature}\:\mathrm{of}\:\mathrm{15}°\mathrm{C}\:.\:\mathrm{25\%}\:\mathrm{of}\:\mathrm{the}\:\mathrm{kinetic} \\ $$$$\mathrm{energy}\:\mathrm{of}\:\mathrm{the}\:\mathrm{pellets}\:\mathrm{is}\:\mathrm{converted}\:\mathrm{thermal}\:\mathrm{energy}\:\mathrm{and}\:\mathrm{the}\:\mathrm{plate}\:\mathrm{temperature} \\ $$$$\mathrm{rises}\:\mathrm{to}\:\mathrm{16}°\mathrm{C}.\:\mathrm{The}\:\mathrm{average}\:\mathrm{speed}\:\mathrm{of}\:\mathrm{the}\:\mathrm{pellets}\:\mathrm{before}\:\mathrm{hitting}\:\mathrm{the}\:\mathrm{plate}\:\mathrm{was}\:? \\ $$

Question Number 11781 Answers: 3 Comments: 0

sin(2θ) + 7cos(2θ) = 6 find θ

$$\mathrm{sin}\left(\mathrm{2}\theta\right)\:+\:\mathrm{7cos}\left(\mathrm{2}\theta\right)\:=\:\mathrm{6} \\ $$$$\mathrm{find}\:\theta \\ $$

Question Number 11632 Answers: 1 Comments: 0

Pg 109 Pg 110 Pg 111 Pg 112 Pg 113 Pg 114 Pg 115 Pg 116 Pg 117 Pg 118

Contact: info@tinkutara.com