
GeometryQuestion and Answers: Page 111
Question Number 16070 Answers: 0 Comments: 0
Question Number 16069 Answers: 0 Comments: 0
Question Number 16068 Answers: 2 Comments: 0
Question Number 16067 Answers: 1 Comments: 8
Question Number 16066 Answers: 2 Comments: 0
Question Number 16065 Answers: 0 Comments: 0
Question Number 16064 Answers: 0 Comments: 8
Question Number 16053 Answers: 0 Comments: 1
Question Number 16014 Answers: 1 Comments: 0
Question Number 15987 Answers: 1 Comments: 1
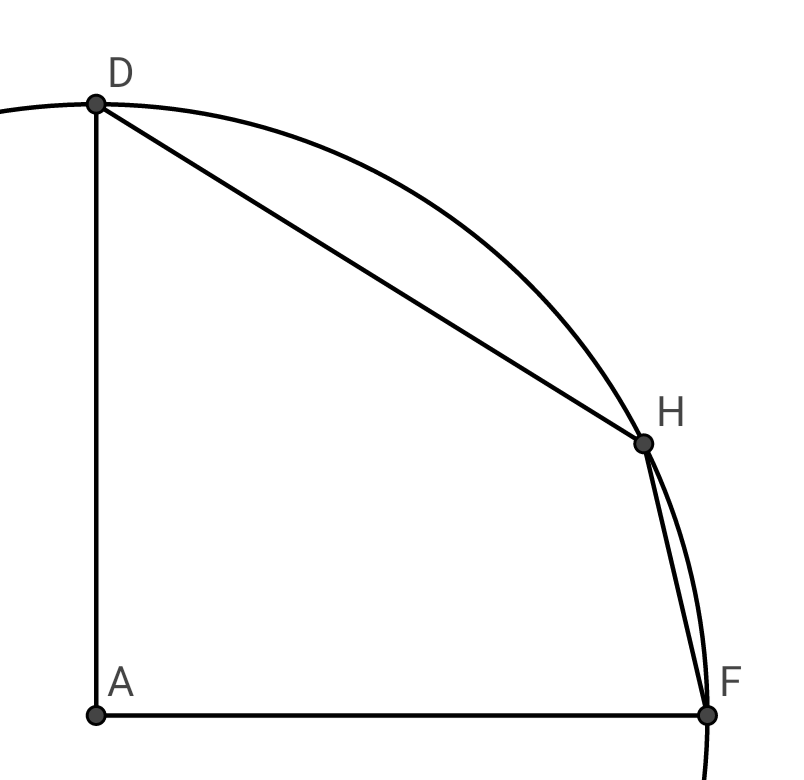
Question Number 15982 Answers: 0 Comments: 3
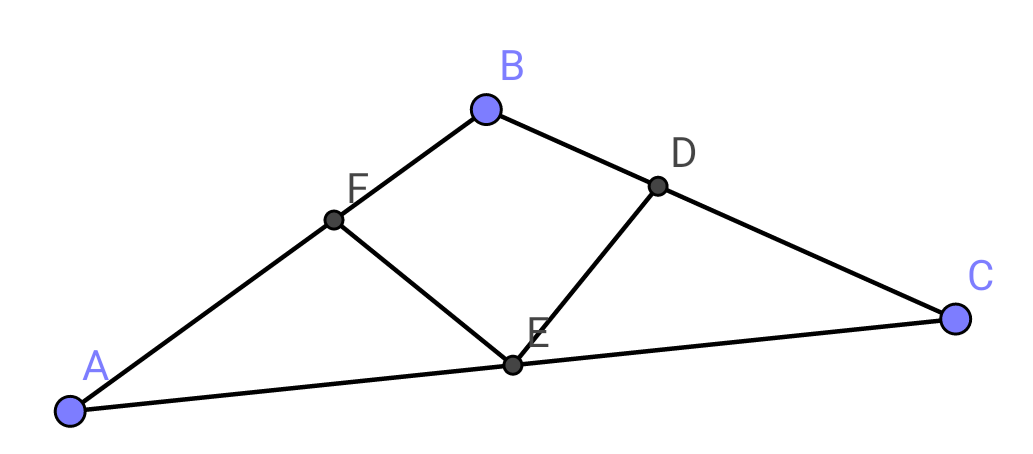
Question Number 15969 Answers: 0 Comments: 17

Question Number 15919 Answers: 0 Comments: 1
$${multiply}\:\mathrm{3}{x}+\mathrm{4}{y}+\mathrm{5}{x}−\mathrm{8}{y} \\ $$
Question Number 15917 Answers: 1 Comments: 1

Question Number 15908 Answers: 1 Comments: 7

Question Number 15904 Answers: 2 Comments: 1

Question Number 15784 Answers: 1 Comments: 1

Question Number 15782 Answers: 1 Comments: 1
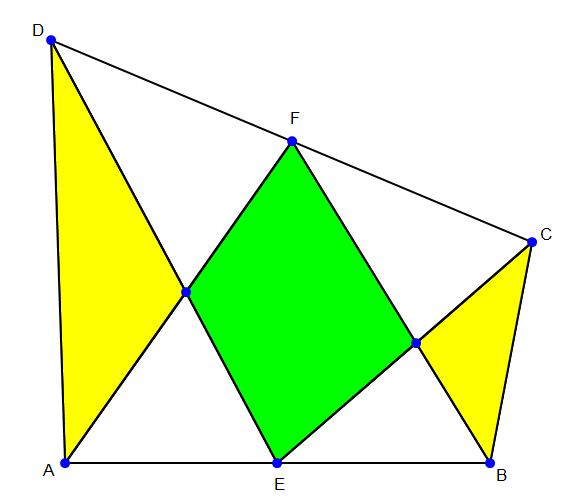
Question Number 15700 Answers: 1 Comments: 1

Question Number 15699 Answers: 1 Comments: 0
Question Number 15672 Answers: 1 Comments: 0
Question Number 15642 Answers: 1 Comments: 0

Question Number 15572 Answers: 2 Comments: 1

Question Number 15567 Answers: 2 Comments: 3
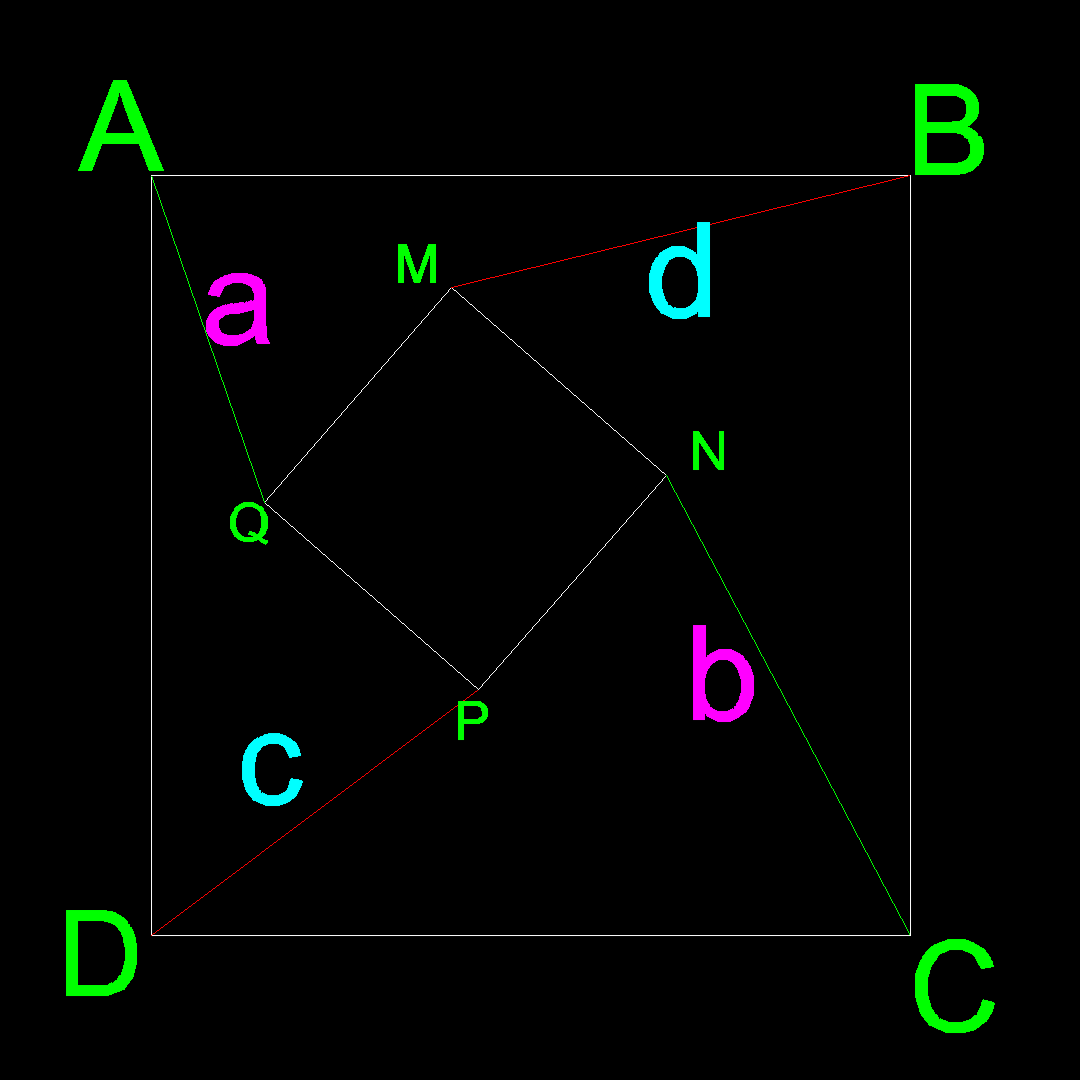
Question Number 16613 Answers: 1 Comments: 2

Question Number 15440 Answers: 3 Comments: 9

Pg 106 Pg 107 Pg 108 Pg 109 Pg 110 Pg 111 Pg 112 Pg 113 Pg 114 Pg 115
