Question and Answers Forum

GeometryQuestion and Answers: Page 110

Question Number 16738 Answers: 0 Comments: 0

Prove that the segments joining the midpoints of the opposite sides of an equiangular hexagon are concurrent.

$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{the}\:\mathrm{segments}\:\mathrm{joining}\:\mathrm{the} \\ $$$$\mathrm{midpoints}\:\mathrm{of}\:\mathrm{the}\:\mathrm{opposite}\:\mathrm{sides}\:\mathrm{of}\:\mathrm{an} \\ $$$$\mathrm{equiangular}\:\mathrm{hexagon}\:\mathrm{are}\:\mathrm{concurrent}. \\ $$

Question Number 16737 Answers: 0 Comments: 0

A convex hexagon is given in which any two opposite sides have the following property: the distance between their midpoints is ((√3)/2) times the sum of their lengths. Prove that the hexagon is equiangular.

$$\mathrm{A}\:\mathrm{convex}\:\mathrm{hexagon}\:\mathrm{is}\:\mathrm{given}\:\mathrm{in}\:\mathrm{which} \\ $$$$\mathrm{any}\:\mathrm{two}\:\mathrm{opposite}\:\mathrm{sides}\:\mathrm{have}\:\mathrm{the} \\ $$$$\mathrm{following}\:\mathrm{property}:\:\mathrm{the}\:\mathrm{distance} \\ $$$$\mathrm{between}\:\mathrm{their}\:\mathrm{midpoints}\:\mathrm{is}\:\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:\mathrm{times}\:\mathrm{the} \\ $$$$\mathrm{sum}\:\mathrm{of}\:\mathrm{their}\:\mathrm{lengths}.\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{the} \\ $$$$\mathrm{hexagon}\:\mathrm{is}\:\mathrm{equiangular}. \\ $$

Question Number 16736 Answers: 0 Comments: 0

The side lengths of an equiangular octagon are rational numbers. Prove that the octagon has a symmetry center.

$$\mathrm{The}\:\mathrm{side}\:\mathrm{lengths}\:\mathrm{of}\:\mathrm{an}\:\mathrm{equiangular} \\ $$$$\mathrm{octagon}\:\mathrm{are}\:\mathrm{rational}\:\mathrm{numbers}.\:\mathrm{Prove} \\ $$$$\mathrm{that}\:\mathrm{the}\:\mathrm{octagon}\:\mathrm{has}\:\mathrm{a}\:\mathrm{symmetry} \\ $$$$\mathrm{center}. \\ $$

Question Number 16735 Answers: 0 Comments: 0

Let a_1 , a_2 , ..., a_n be the side lengths of an equiangular polygon. Prove that if a_1 ≥ a_2 ≥ ... ≥ a_n , then the polygon is regular.

$$\mathrm{Let}\:{a}_{\mathrm{1}} ,\:{a}_{\mathrm{2}} ,\:...,\:{a}_{{n}} \:\mathrm{be}\:\mathrm{the}\:\mathrm{side}\:\mathrm{lengths}\:\mathrm{of}\: \\ $$$$\mathrm{an}\:\mathrm{equiangular}\:\mathrm{polygon}.\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{if} \\ $$$${a}_{\mathrm{1}} \:\geqslant\:{a}_{\mathrm{2}} \:\geqslant\:...\:\geqslant\:{a}_{{n}} ,\:\mathrm{then}\:\mathrm{the}\:\mathrm{polygon}\:\mathrm{is} \\ $$$$\mathrm{regular}. \\ $$

Question Number 16734 Answers: 0 Comments: 0

An equiangular polygon with an odd number of sides is inscribed in a circle. Prove that the polygon is regular.

$$\mathrm{An}\:\mathrm{equiangular}\:\mathrm{polygon}\:\mathrm{with}\:\mathrm{an}\:\mathrm{odd} \\ $$$$\mathrm{number}\:\mathrm{of}\:\mathrm{sides}\:\mathrm{is}\:\mathrm{inscribed}\:\mathrm{in}\:\mathrm{a}\:\mathrm{circle}. \\ $$$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{the}\:\mathrm{polygon}\:\mathrm{is}\:\mathrm{regular}. \\ $$

Question Number 16754 Answers: 2 Comments: 1

Question Number 16665 Answers: 0 Comments: 1

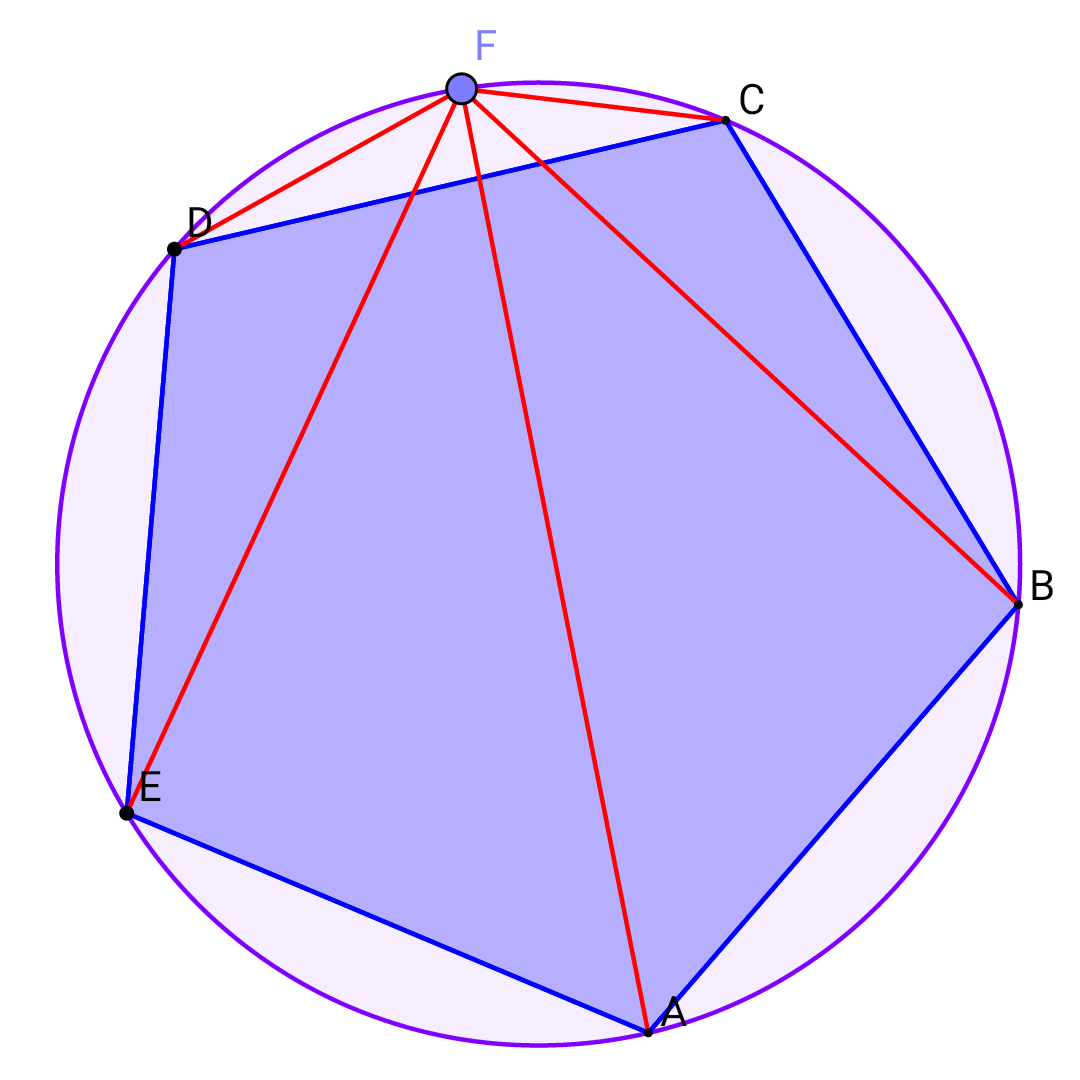
Question Number 16641 Answers: 0 Comments: 0

Prove that p is a prime number if and only if every equiangular polygon with p sides of rational lengths is regular.

$$\mathrm{Prove}\:\mathrm{that}\:{p}\:\mathrm{is}\:\mathrm{a}\:\mathrm{prime}\:\mathrm{number}\:\mathrm{if}\:\mathrm{and} \\ $$$$\mathrm{only}\:\mathrm{if}\:\mathrm{every}\:\mathrm{equiangular}\:\mathrm{polygon}\:\mathrm{with} \\ $$$${p}\:\mathrm{sides}\:\mathrm{of}\:\mathrm{rational}\:\mathrm{lengths}\:\mathrm{is}\:\mathrm{regular}. \\ $$

Question Number 16595 Answers: 1 Comments: 4

Question Number 16592 Answers: 1 Comments: 1

please what does the question mean by the overlapping portion of A and B.

$$\mathrm{please}\:\mathrm{what}\:\mathrm{does}\:\mathrm{the}\:\mathrm{question}\:\mathrm{mean} \\ $$$$\mathrm{by}\:\mathrm{the}\:\mathrm{overlapping}\:\mathrm{portion}\:\mathrm{of}\:\mathrm{A}\: \\ $$$$\mathrm{and}\:\mathrm{B}. \\ $$

Question Number 16579 Answers: 0 Comments: 3

Question Number 16570 Answers: 0 Comments: 1

A,B and E are three circles all with radius 1 unit.A and E touch at P whole B and E touch at Q. ∠POQ=x° where O is the centre of E.Find the area of the overlapping portion of A and B if 0≤x≤60°

$$\mathrm{A},\mathrm{B}\:\mathrm{and}\:\mathrm{E}\:\mathrm{are}\:\mathrm{three}\:\mathrm{circles}\:\mathrm{all}\: \\ $$$$\mathrm{with}\:\mathrm{radius}\:\mathrm{1}\:\mathrm{unit}.\mathrm{A}\:\mathrm{and}\:\mathrm{E}\:\mathrm{touch} \\ $$$$\mathrm{at}\:\mathrm{P}\:\mathrm{whole}\:\mathrm{B}\:\mathrm{and}\:\mathrm{E}\:\mathrm{touch}\:\mathrm{at}\:\mathrm{Q}. \\ $$$$\angle\mathrm{POQ}=\mathrm{x}°\:\mathrm{where}\:\mathrm{O}\:\mathrm{is}\:\mathrm{the}\:\mathrm{centre}\: \\ $$$$\mathrm{of}\:\mathrm{E}.\mathrm{Find}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\: \\ $$$$\mathrm{overlapping}\:\mathrm{portion}\:\mathrm{of}\:\mathrm{A}\:\mathrm{and}\:\mathrm{B}\:\mathrm{if} \\ $$$$\mathrm{0}\leqslant\mathrm{x}\leqslant\mathrm{60}° \\ $$

Question Number 16483 Answers: 0 Comments: 12

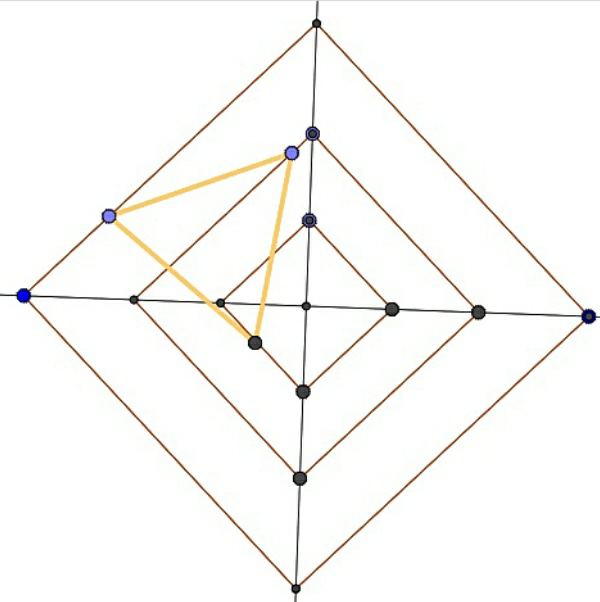
Question Number 16464 Answers: 0 Comments: 2

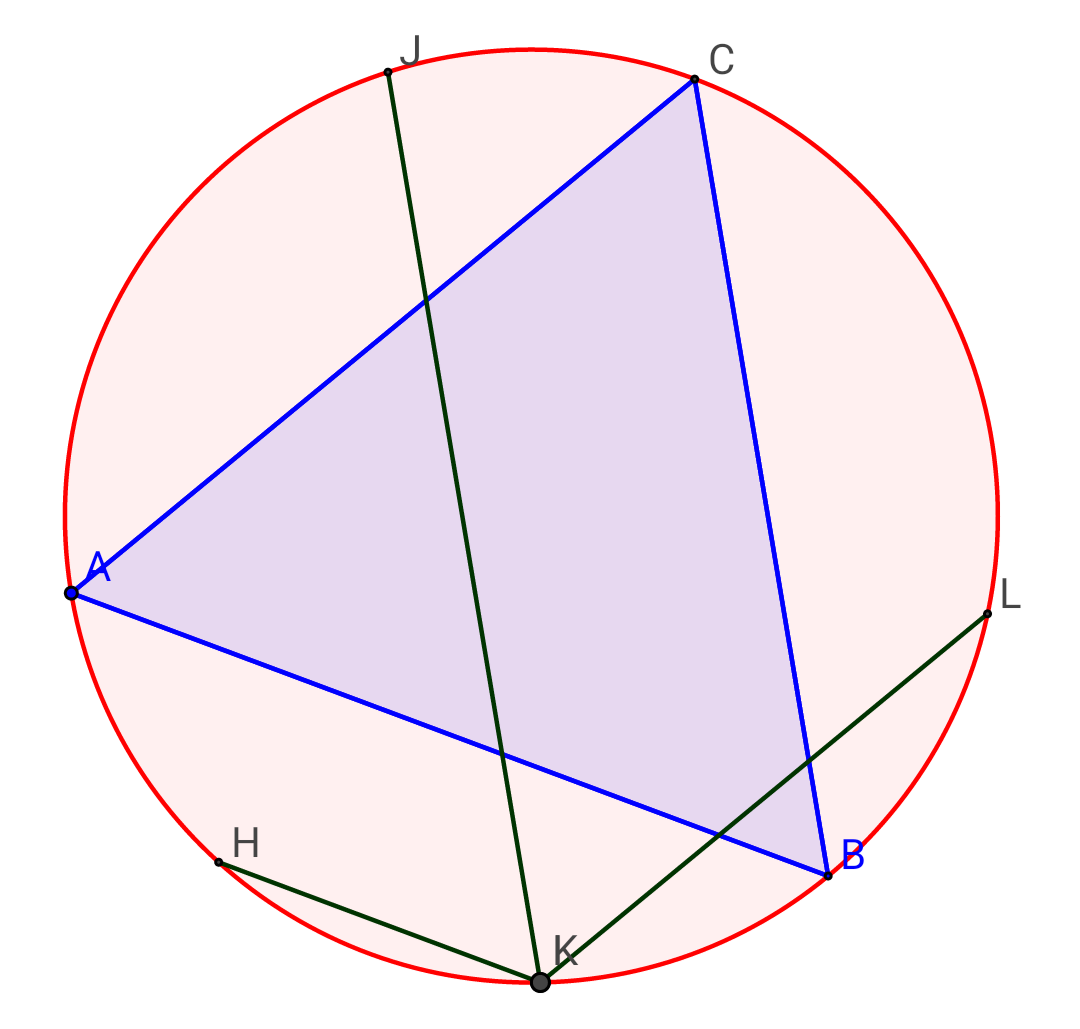
Question Number 16409 Answers: 3 Comments: 9

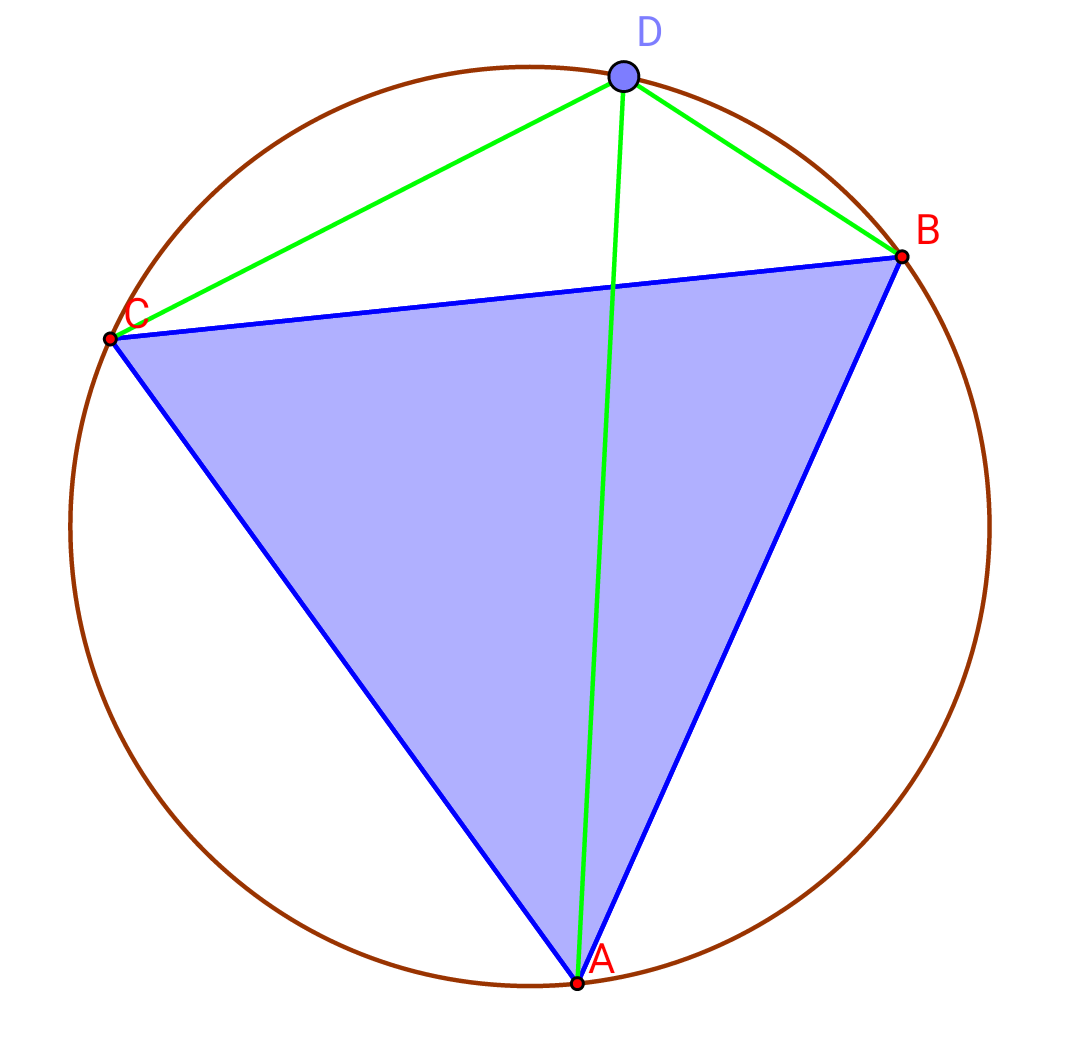
Question Number 16364 Answers: 1 Comments: 0

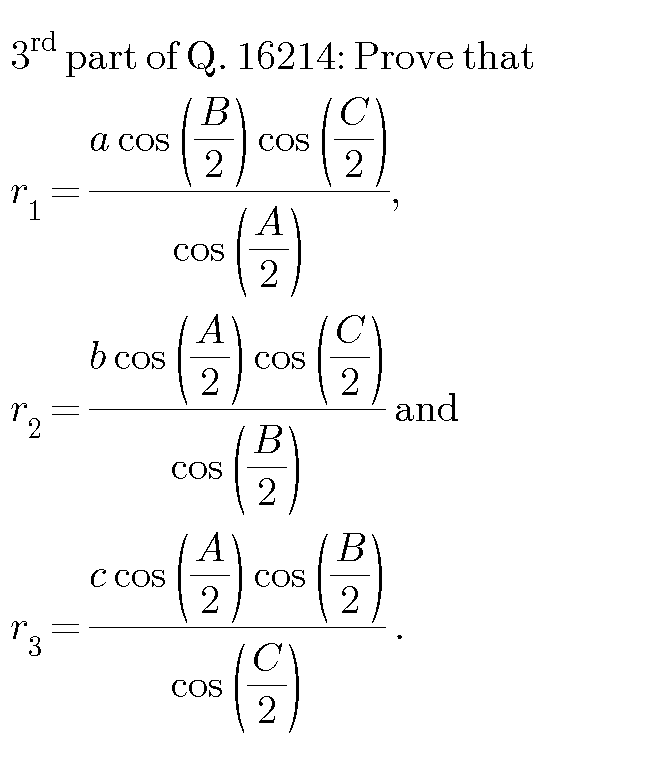
Question Number 16441 Answers: 1 Comments: 2

Question Number 16302 Answers: 1 Comments: 5

Related to Q16140 What if the three lines d_1 ,d_2 ,d_3 are not parallel, but concurrent?

Question Number 16277 Answers: 3 Comments: 1

Question Number 16226 Answers: 0 Comments: 1

Question Number 16214 Answers: 2 Comments: 4

In ΔABC, r_1 , r_2 and r_3 are the exradii as shown. Prove that r_1 = (Δ/(s − a)) , r_2 = (Δ/(s − b)) and r_3 = (Δ/(s − c)) . Here s = ((a + b + c)/2) .

$$\mathrm{In}\:\Delta{ABC},\:{r}_{\mathrm{1}} ,\:{r}_{\mathrm{2}} \:\mathrm{and}\:{r}_{\mathrm{3}} \:\mathrm{are}\:\mathrm{the}\:\mathrm{exradii} \\ $$$$\mathrm{as}\:\mathrm{shown}.\:\mathrm{Prove}\:\mathrm{that}\:{r}_{\mathrm{1}} \:=\:\frac{\Delta}{{s}\:−\:{a}}\:, \\ $$$${r}_{\mathrm{2}} \:=\:\frac{\Delta}{{s}\:−\:{b}}\:\mathrm{and}\:{r}_{\mathrm{3}} \:=\:\frac{\Delta}{{s}\:−\:{c}}\:.\:\mathrm{Here} \\ $$$${s}\:=\:\frac{{a}\:+\:{b}\:+\:{c}}{\mathrm{2}}\:. \\ $$

Question Number 16194 Answers: 0 Comments: 21

Question Number 16140 Answers: 2 Comments: 0

Question Number 16110 Answers: 0 Comments: 1

let a_1 >a_2 >0 and a_(n+1) =(√(a_n a_(n−1 ) )) where n is greater than equal to 2 Then The sequence {a_(2n) } is (1) monotonic increasing (2)monotonic decreasing (3)non monotonic (4)unbounded

$$\mathrm{let}\:\mathrm{a}_{\mathrm{1}} >\mathrm{a}_{\mathrm{2}} >\mathrm{0}\:\mathrm{and}\:\mathrm{a}_{\mathrm{n}+\mathrm{1}} =\sqrt{\mathrm{a}_{\mathrm{n}} \mathrm{a}_{\mathrm{n}−\mathrm{1}\:\:\:} } \\ $$$$\mathrm{where}\:\mathrm{n}\:\mathrm{is}\:\mathrm{greater}\:\mathrm{than}\:\mathrm{equal}\:\mathrm{to}\:\mathrm{2}\: \\ $$$$\mathrm{Then} \\ $$$$\mathrm{The}\:\mathrm{sequence}\:\left\{\mathrm{a}_{\mathrm{2n}} \right\}\:\mathrm{is}\: \\ $$$$\left(\mathrm{1}\right)\:\mathrm{monotonic}\:\mathrm{increasing} \\ $$$$\left(\mathrm{2}\right)\mathrm{monotonic}\:\mathrm{decreasing} \\ $$$$\left(\mathrm{3}\right)\mathrm{non}\:\mathrm{monotonic} \\ $$$$\left(\mathrm{4}\right)\mathrm{unbounded} \\ $$$$ \\ $$

Question Number 16108 Answers: 1 Comments: 1

Question Number 16077 Answers: 0 Comments: 0

Let ABCDE be an equiangular pentagon whose side lengths are rational numbers. Prove that the pentagon is regular.

$$\mathrm{Let}\:{ABCDE}\:\mathrm{be}\:\mathrm{an}\:\mathrm{equiangular} \\ $$$$\mathrm{pentagon}\:\mathrm{whose}\:\mathrm{side}\:\mathrm{lengths}\:\mathrm{are} \\ $$$$\mathrm{rational}\:\mathrm{numbers}.\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{the} \\ $$$$\mathrm{pentagon}\:\mathrm{is}\:\mathrm{regular}. \\ $$

Pg 105 Pg 106 Pg 107 Pg 108 Pg 109 Pg 110 Pg 111 Pg 112 Pg 113 Pg 114

Contact: info@tinkutara.com