Question and Answers Forum

GeometryQuestion and Answers: Page 108

Question Number 18461 Answers: 1 Comments: 0

Question Number 18394 Answers: 0 Comments: 0

Question Number 18323 Answers: 0 Comments: 0

Σ((cos 2rθ)/(sin^2 2rθ−sin^2 θ))

$$\Sigma\frac{\mathrm{cos}\:\mathrm{2}{r}\theta}{\mathrm{sin}\:^{\mathrm{2}} \mathrm{2}{r}\theta−\mathrm{sin}\:^{\mathrm{2}} \theta} \\ $$

Question Number 18236 Answers: 0 Comments: 0

∫ (dx/(1 − sin x + cos x))

$$\int\:\frac{{dx}}{\mathrm{1}\:−\:\mathrm{sin}\:{x}\:+\:\mathrm{cos}\:{x}} \\ $$

Question Number 20977 Answers: 0 Comments: 1

Imtegrate ∫e^(−ax^2 +bx+c) dx for a>0. It′s just for fun. If you have questions leave a comment. I′ll do my best to answer them.

$${Imtegrate}\:\int{e}^{−{ax}^{\mathrm{2}} +{bx}+{c}} {dx}\:{for}\:{a}>\mathrm{0}. \\ $$$${It}'{s}\:{just}\:{for}\:{fun}.\:{If}\:{you}\:{have}\:{questions} \\ $$$${leave}\:{a}\:{comment}.\:{I}'{ll}\:{do}\:{my}\:{best}\:{to}\:{answer}\:{them}. \\ $$

Question Number 17939 Answers: 1 Comments: 1

∫secxdx

$$\int{secxdx}\: \\ $$

Question Number 17901 Answers: 1 Comments: 2

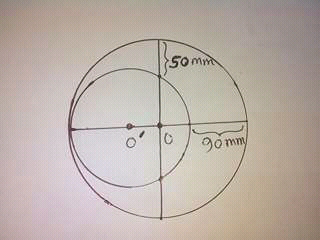
Question Number 17948 Answers: 1 Comments: 3

Question Number 17886 Answers: 0 Comments: 7

Question Number 17884 Answers: 1 Comments: 1

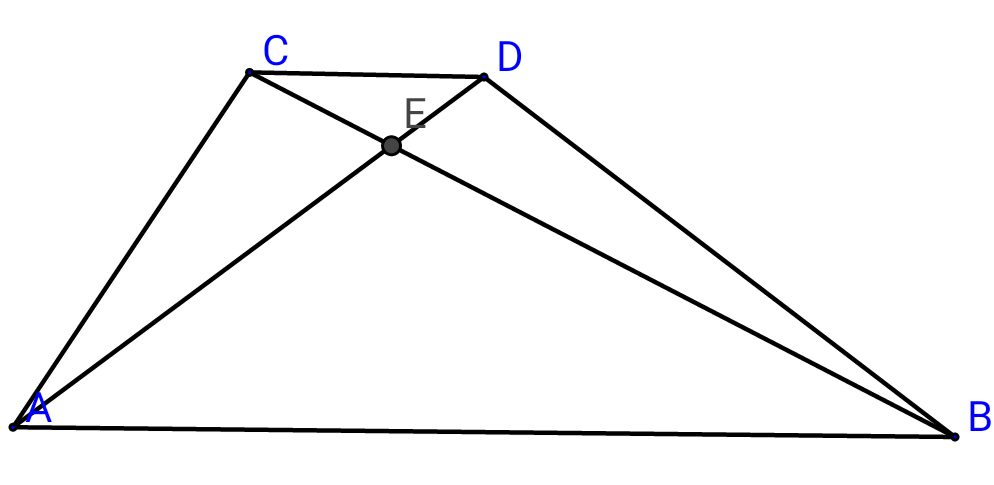
Question Number 17935 Answers: 0 Comments: 0

Ball A is dropped from the top of a building. At the same instant ball B is thrown vertically upwards from the ground. When the ball collide, they are moving in opposite directions and the speed of A(u) is twice the speed of B. The relative velocity of the ball just before collision and relative acceleration between them is (only their magnitudes) (A) 0 and 0 (B) ((3u)/2) and 0 (C) ((3u)/2) and 2g (D) ((3u)/2) and g

$$\mathrm{Ball}\:{A}\:\mathrm{is}\:\mathrm{dropped}\:\mathrm{from}\:\mathrm{the}\:\mathrm{top}\:\mathrm{of}\:\mathrm{a}\:\mathrm{building}. \\ $$$$\mathrm{At}\:\mathrm{the}\:\mathrm{same}\:\mathrm{instant}\:\mathrm{ball}\:{B}\:\mathrm{is}\:\mathrm{thrown} \\ $$$$\mathrm{vertically}\:\mathrm{upwards}\:\mathrm{from}\:\mathrm{the}\:\mathrm{ground}. \\ $$$$\mathrm{When}\:\mathrm{the}\:\mathrm{ball}\:\mathrm{collide},\:\mathrm{they}\:\mathrm{are}\:\mathrm{moving}\:\:\mathrm{in} \\ $$$$\mathrm{opposite}\:\mathrm{directions}\:\mathrm{and}\:\mathrm{the}\:\mathrm{speed}\:\mathrm{of}\:{A}\left({u}\right) \\ $$$$\mathrm{is}\:\mathrm{twice}\:\mathrm{the}\:\mathrm{speed}\:\mathrm{of}\:{B}.\:\mathrm{The}\:\mathrm{relative}\: \\ $$$$\mathrm{velocity}\:\mathrm{of}\:\mathrm{the}\:\mathrm{ball}\:\mathrm{just}\:\mathrm{before}\:\mathrm{collision} \\ $$$$\mathrm{and}\:\mathrm{relative}\:\mathrm{acceleration}\:\mathrm{between}\:\mathrm{them} \\ $$$$\mathrm{is}\:\left(\mathrm{only}\:\mathrm{their}\:\mathrm{magnitudes}\right) \\ $$$$\left(\mathrm{A}\right)\:\mathrm{0}\:\mathrm{and}\:\mathrm{0}\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\frac{\mathrm{3}{u}}{\mathrm{2}}\:\mathrm{and}\:\mathrm{0} \\ $$$$\left(\mathrm{C}\right)\:\frac{\mathrm{3}{u}}{\mathrm{2}}\:\mathrm{and}\:\mathrm{2}{g}\:\:\:\left(\mathrm{D}\right)\:\frac{\mathrm{3}{u}}{\mathrm{2}}\:\mathrm{and}\:{g} \\ $$

Question Number 17771 Answers: 1 Comments: 1

Question Number 17743 Answers: 2 Comments: 0

Question Number 17692 Answers: 1 Comments: 0

Question Number 17653 Answers: 0 Comments: 6

A line segment moves in the plane with its end points on the coordinate axes so that the sum of the length of its intersect on the coordinate axes is a constant C . Find the locus of the mid points of this segment . Ans. is 8(∣x∣^3 +∣y∣^3 )=C . Λ means power . pls. solve it.

$$\mathrm{A}\:\mathrm{line}\:\mathrm{segment}\:\mathrm{moves}\:\mathrm{in}\:\mathrm{the}\:\mathrm{plane} \\ $$$$\mathrm{with}\:\mathrm{its}\:\mathrm{end}\:\mathrm{points}\:\mathrm{on}\:\mathrm{the}\:\mathrm{coordinate} \\ $$$$\mathrm{axes}\:\mathrm{so}\:\mathrm{that}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{length} \\ $$$$\mathrm{of}\:\mathrm{its}\:\mathrm{intersect}\:\mathrm{on}\:\mathrm{the}\:\mathrm{coordinate}\: \\ $$$$\mathrm{axes}\:\mathrm{is}\:\mathrm{a}\:\mathrm{constant}\:\mathrm{C}\:. \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{locus}\:\mathrm{of}\:\mathrm{the}\:\mathrm{mid}\:\mathrm{points}\:\mathrm{of} \\ $$$$\mathrm{this}\:\mathrm{segment}\:. \\ $$$$\mathrm{Ans}.\:\mathrm{is}\:\:\:\mathrm{8}\left(\mid\mathrm{x}\mid^{\mathrm{3}} +\mid\mathrm{y}\mid^{\mathrm{3}} \right)=\mathrm{C}\:. \\ $$$$\Lambda\:\:\mathrm{means}\:\mathrm{power}\:.\:\mathrm{pls}.\:\mathrm{solve}\:\mathrm{it}. \\ $$

Question Number 17614 Answers: 0 Comments: 3

The triangle ABC has CA = CB. P is a point on the circumcircle between A and B (and on the opposite side of the line AB to C). D is the foot of the perpendicular from C to PB. Show that PA + PB = 2∙PD.

$$\mathrm{The}\:\mathrm{triangle}\:\mathrm{ABC}\:\mathrm{has}\:\mathrm{CA}\:=\:\mathrm{CB}.\:\mathrm{P}\:\mathrm{is}\:\mathrm{a} \\ $$$$\mathrm{point}\:\mathrm{on}\:\mathrm{the}\:\mathrm{circumcircle}\:\mathrm{between}\:\mathrm{A} \\ $$$$\mathrm{and}\:\mathrm{B}\:\left(\mathrm{and}\:\mathrm{on}\:\mathrm{the}\:\mathrm{opposite}\:\mathrm{side}\:\mathrm{of}\:\mathrm{the}\right. \\ $$$$\left.\mathrm{line}\:\mathrm{AB}\:\mathrm{to}\:\mathrm{C}\right).\:\mathrm{D}\:\mathrm{is}\:\mathrm{the}\:\mathrm{foot}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{perpendicular}\:\mathrm{from}\:\mathrm{C}\:\mathrm{to}\:\mathrm{PB}.\:\mathrm{Show}\:\mathrm{that} \\ $$$$\mathrm{PA}\:+\:\mathrm{PB}\:=\:\mathrm{2}\centerdot\mathrm{PD}. \\ $$

Question Number 17580 Answers: 0 Comments: 8

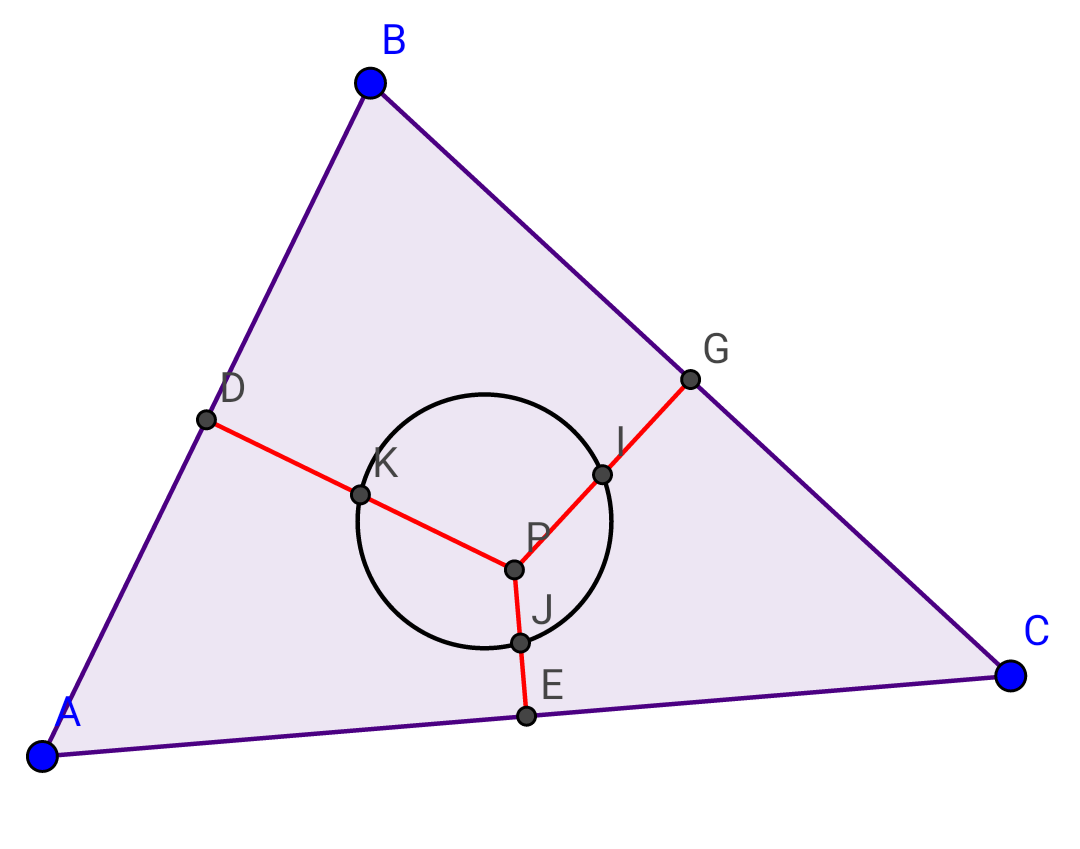
Question Number 17524 Answers: 1 Comments: 0

The circle ω touches the circle Ω internally at P. The centre O of Ω is outside ω. Let XY be a diameter of Ω which is also tangent to ω. Assume PY > PX. Let PY intersect ω at Z. If YZ = 2PZ, what is the magnitude of ∠PYX in degrees?

$$\mathrm{The}\:\mathrm{circle}\:\omega\:\mathrm{touches}\:\mathrm{the}\:\mathrm{circle}\:\Omega \\ $$$$\mathrm{internally}\:\mathrm{at}\:{P}.\:\mathrm{The}\:\mathrm{centre}\:{O}\:\mathrm{of}\:\Omega\:\mathrm{is} \\ $$$$\mathrm{outside}\:\omega.\:\mathrm{Let}\:{XY}\:\mathrm{be}\:\mathrm{a}\:\mathrm{diameter}\:\mathrm{of}\:\Omega \\ $$$$\mathrm{which}\:\mathrm{is}\:\mathrm{also}\:\mathrm{tangent}\:\mathrm{to}\:\omega.\:\mathrm{Assume} \\ $$$${PY}\:>\:{PX}.\:\mathrm{Let}\:{PY}\:\mathrm{intersect}\:\omega\:\mathrm{at}\:{Z}.\:\mathrm{If} \\ $$$${YZ}\:=\:\mathrm{2}{PZ},\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{magnitude}\:\mathrm{of} \\ $$$$\angle{PYX}\:\mathrm{in}\:\mathrm{degrees}? \\ $$

Question Number 17520 Answers: 1 Comments: 1

Find the coordinate of the point in RΛ3 which is the reflection the point (1,2,3) with respect to plane X+Y+Z=1 .

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{coordinate}\:\mathrm{of}\:\mathrm{the}\:\mathrm{point}\:\mathrm{in} \\ $$$$\mathrm{R}\Lambda\mathrm{3}\:\mathrm{which}\:\mathrm{is}\:\mathrm{the}\:\mathrm{reflection}\:\mathrm{the}\:\mathrm{point} \\ $$$$\left(\mathrm{1},\mathrm{2},\mathrm{3}\right)\:\mathrm{with}\:\mathrm{respect}\:\mathrm{to}\:\mathrm{plane}\: \\ $$$$\mathrm{X}+\mathrm{Y}+\mathrm{Z}=\mathrm{1}\:. \\ $$

Question Number 17449 Answers: 1 Comments: 4

Between 2:00 and 2:15, what time is it exactly when the hour, minute, and second′s hand of a clock occupy the same angular position.

$$\mathrm{Between}\:\mathrm{2}:\mathrm{00}\:\mathrm{and}\:\mathrm{2}:\mathrm{15},\:\mathrm{what}\:\mathrm{time} \\ $$$$\mathrm{is}\:\mathrm{it}\:\mathrm{exactly}\:\mathrm{when}\:\mathrm{the}\:\mathrm{hour}, \\ $$$$\mathrm{minute},\:\mathrm{and}\:\mathrm{second}'\mathrm{s}\:\mathrm{hand}\:\mathrm{of}\:\mathrm{a} \\ $$$$\mathrm{clock}\:\mathrm{occupy}\:\mathrm{the}\:\mathrm{same}\:\mathrm{angular} \\ $$$$\mathrm{position}. \\ $$$$ \\ $$

Question Number 17440 Answers: 1 Comments: 0

3x−4y=0,4y−5z=0,5z−3x=0 then x,y,z is AP,GP,HP,AGP??????

$$\mathrm{3}{x}−\mathrm{4}{y}=\mathrm{0},\mathrm{4}{y}−\mathrm{5}{z}=\mathrm{0},\mathrm{5}{z}−\mathrm{3}{x}=\mathrm{0} \\ $$$${then}\:{x},{y},{z}\:{is}\:{AP},{GP},{HP},{AGP}?????? \\ $$

Question Number 17645 Answers: 2 Comments: 1

Suppose that the point M lying in the interior of the parallelogram ABCD, two parallels to AB and AD are drawn, intersecting the sides of ABCD at the points P, Q, R, S (See Figure). Prove that M lies on the diagonal AC if and only if [MRDS] = [MPBQ].

$$\mathrm{Suppose}\:\mathrm{that}\:\mathrm{the}\:\mathrm{point}\:{M}\:\mathrm{lying}\:\mathrm{in}\:\mathrm{the} \\ $$$$\mathrm{interior}\:\mathrm{of}\:\mathrm{the}\:\mathrm{parallelogram}\:{ABCD}, \\ $$$$\mathrm{two}\:\mathrm{parallels}\:\mathrm{to}\:{AB}\:\mathrm{and}\:{AD}\:\mathrm{are}\:\mathrm{drawn}, \\ $$$$\mathrm{intersecting}\:\mathrm{the}\:\mathrm{sides}\:\mathrm{of}\:{ABCD}\:\mathrm{at}\:\mathrm{the} \\ $$$$\mathrm{points}\:{P},\:{Q},\:{R},\:{S}\:\left(\mathrm{See}\:\mathrm{Figure}\right).\:\mathrm{Prove} \\ $$$$\mathrm{that}\:{M}\:\mathrm{lies}\:\mathrm{on}\:\mathrm{the}\:\mathrm{diagonal}\:{AC}\:\mathrm{if}\:\mathrm{and} \\ $$$$\mathrm{only}\:\mathrm{if}\:\left[{MRDS}\right]\:=\:\left[{MPBQ}\right]. \\ $$

Question Number 17373 Answers: 2 Comments: 0

Find the point in interior of a convex quadrilateral such that the sum of its distances to the 4 vertices is minimal. Find the point in interior of a convex quadrilateral such that the sum of its distances to the 4 sides is minimal.

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{point}\:\mathrm{in}\:\mathrm{interior}\:\mathrm{of}\:\mathrm{a}\:\mathrm{convex} \\ $$$$\mathrm{quadrilateral}\:\mathrm{such}\:\mathrm{that}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{its} \\ $$$$\mathrm{distances}\:\mathrm{to}\:\mathrm{the}\:\mathrm{4}\:\mathrm{vertices}\:\mathrm{is}\:\mathrm{minimal}. \\ $$$$ \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{point}\:\mathrm{in}\:\mathrm{interior}\:\mathrm{of}\:\mathrm{a}\:\mathrm{convex} \\ $$$$\mathrm{quadrilateral}\:\mathrm{such}\:\mathrm{that}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{its} \\ $$$$\mathrm{distances}\:\mathrm{to}\:\mathrm{the}\:\mathrm{4}\:\mathrm{sides}\:\mathrm{is}\:\mathrm{minimal}. \\ $$

Question Number 17273 Answers: 1 Comments: 2

The intersection of the ABC triangle median is at G point. The corner of the BGC is 90°. If the AG cut length is 12 cm, locate the BC side.

Question Number 17177 Answers: 1 Comments: 0

Question Number 17158 Answers: 0 Comments: 4

Please solve Q. 16069. Ask from me the solution if needed and please explain it.

$$\mathrm{Please}\:\mathrm{solve}\:\mathrm{Q}.\:\mathrm{16069}.\:\mathrm{Ask}\:\mathrm{from}\:\mathrm{me}\:\mathrm{the} \\ $$$$\mathrm{solution}\:\mathrm{if}\:\mathrm{needed}\:\mathrm{and}\:\mathrm{please}\:\mathrm{explain}\:\mathrm{it}. \\ $$

Pg 103 Pg 104 Pg 105 Pg 106 Pg 107 Pg 108 Pg 109 Pg 110 Pg 111 Pg 112

Contact: info@tinkutara.com