Question and Answers Forum

GeometryQuestion and Answers: Page 107

Question Number 20156 Answers: 1 Comments: 0

Solve: inverse laplace. L^(−1) ((s/(s^(2 ) + 6s + 25)))

$$\mathrm{Solve}:\:\mathrm{inverse}\:\mathrm{laplace}.\:\:\:\:\mathrm{L}^{−\mathrm{1}} \left(\frac{\mathrm{s}}{\mathrm{s}^{\mathrm{2}\:} +\:\mathrm{6s}\:+\:\mathrm{25}}\right) \\ $$

Question Number 20131 Answers: 1 Comments: 4

In rectangle ABCD,AB=8, BC=20.P is a point on AD so that ∠BPC=90°.If r_1 ,r_2 ,r_3 are the radii of the incircles of APB, BPC, and CPD. find r_1 +r_2 +r_3

$${In}\:{rectangle}\:{ABCD},{AB}=\mathrm{8}, \\ $$$${BC}=\mathrm{20}.{P}\:{is}\:{a}\:{point}\:{on}\:{AD}\:{so} \\ $$$${that}\:\angle{BPC}=\mathrm{90}°.{If}\:{r}_{\mathrm{1}} ,{r}_{\mathrm{2}} ,{r}_{\mathrm{3}} \:{are}\:{the} \\ $$$${radii}\:{of}\:{the}\:{incircles}\:{of}\:{APB}, \\ $$$${BPC},\:{and}\:{CPD}.\:{find}\:{r}_{\mathrm{1}} +{r}_{\mathrm{2}} +{r}_{\mathrm{3}} \\ $$

Question Number 19939 Answers: 0 Comments: 0

f(x)=lnx

$${f}\left({x}\right)={lnx} \\ $$

Question Number 19794 Answers: 0 Comments: 0

In a triangle ABC with ∠BCA = 90°, the perpendicular bisector of AB intersects segments AB and AC at X and Y, respectively. If the ratio of the area of quadrilateral BXYC to the area of triangle ABC is 13 : 18 and BC = 12 then what is the length of AC?

$$\mathrm{In}\:\mathrm{a}\:\mathrm{triangle}\:{ABC}\:\mathrm{with}\:\angle{BCA}\:=\:\mathrm{90}°, \\ $$$$\mathrm{the}\:\mathrm{perpendicular}\:\mathrm{bisector}\:\mathrm{of}\:{AB} \\ $$$$\mathrm{intersects}\:\mathrm{segments}\:{AB}\:\mathrm{and}\:{AC}\:\mathrm{at}\:{X} \\ $$$$\mathrm{and}\:{Y},\:\mathrm{respectively}.\:\mathrm{If}\:\mathrm{the}\:\mathrm{ratio}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{area}\:\mathrm{of}\:\mathrm{quadrilateral}\:{BXYC}\:\mathrm{to}\:\mathrm{the} \\ $$$$\mathrm{area}\:\mathrm{of}\:\mathrm{triangle}\:{ABC}\:\mathrm{is}\:\mathrm{13}\::\:\mathrm{18}\:\mathrm{and} \\ $$$${BC}\:=\:\mathrm{12}\:\mathrm{then}\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{length}\:\mathrm{of}\:{AC}? \\ $$

Question Number 19792 Answers: 2 Comments: 0

Let ABCD be a convex quadrilateral with ∠DAB = ∠BDC = 90°. Let the incircles of triangles ABD and BCD touch BD at P and Q, respectively, with P lying in between B and Q. If AD = 999 and PQ = 200 then what is the sum of the radii of the incircles of triangles ABD and BDC?

$$\mathrm{Let}\:{ABCD}\:\mathrm{be}\:\mathrm{a}\:\mathrm{convex}\:\mathrm{quadrilateral} \\ $$$$\mathrm{with}\:\angle{DAB}\:=\:\angle{BDC}\:=\:\mathrm{90}°.\:\mathrm{Let}\:\mathrm{the} \\ $$$$\mathrm{incircles}\:\mathrm{of}\:\mathrm{triangles}\:{ABD}\:\mathrm{and}\:{BCD} \\ $$$$\mathrm{touch}\:{BD}\:\mathrm{at}\:{P}\:\mathrm{and}\:{Q},\:\mathrm{respectively}, \\ $$$$\mathrm{with}\:{P}\:\mathrm{lying}\:\mathrm{in}\:\mathrm{between}\:{B}\:\mathrm{and}\:{Q}.\:\mathrm{If} \\ $$$${AD}\:=\:\mathrm{999}\:\mathrm{and}\:{PQ}\:=\:\mathrm{200}\:\mathrm{then}\:\mathrm{what}\:\mathrm{is} \\ $$$$\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{radii}\:\mathrm{of}\:\mathrm{the}\:\mathrm{incircles}\:\mathrm{of} \\ $$$$\mathrm{triangles}\:{ABD}\:\mathrm{and}\:{BDC}? \\ $$

Question Number 19709 Answers: 1 Comments: 0

In the cyclic quadrilateral ABCD AB=7,BC=8,CD=8,DA=15. Calculate the angle ADC and the length ofAC.

$${In}\:{the}\:{cyclic}\:{quadrilateral}\:{ABCD} \\ $$$${AB}=\mathrm{7},{BC}=\mathrm{8},{CD}=\mathrm{8},{DA}=\mathrm{15}. \\ $$$${Calculate}\:{the}\:{angle}\:{ADC}\:{and} \\ $$$${the}\:{length}\:{ofAC}. \\ $$

Question Number 19783 Answers: 1 Comments: 3

The sides of a triangle are of lengths (√((m^2 −n^2 ))) ,m^2 +n^2 , 2mn. Show that it is a right angle Δ.

$${The}\:{sides}\:{of}\:{a}\:{triangle}\:{are}\:{of} \\ $$$${lengths}\:\sqrt{\left({m}^{\mathrm{2}} −{n}^{\mathrm{2}} \right)}\:,{m}^{\mathrm{2}} +{n}^{\mathrm{2}} ,\:\mathrm{2}{mn}. \\ $$$${Show}\:{that}\:{it}\:{is}\:{a}\:{right}\:{angle}\:\Delta. \\ $$

Question Number 19699 Answers: 0 Comments: 0

Let S be a circle with centre O. A chord AB, not a diameter, divides S into two regions R_1 and R_2 such that O belongs to R_2 . Let S_1 be a circle with centre in R_1 , touching AB at X and S internally. Let S_2 be a circle with centre in R_2 , touching AB at Y, the circle S internally and passing through the centre of S. The point X lies on the diameter passing through the centre of S_2 and ∠YXO = 30°. If the radius of S_2 is 100 then what is the radius of S_1 ?

$$\mathrm{Let}\:{S}\:\mathrm{be}\:\mathrm{a}\:\mathrm{circle}\:\mathrm{with}\:\mathrm{centre}\:{O}.\:\mathrm{A}\:\mathrm{chord} \\ $$$${AB},\:\mathrm{not}\:\mathrm{a}\:\mathrm{diameter},\:\mathrm{divides}\:{S}\:\mathrm{into}\:\mathrm{two} \\ $$$$\mathrm{regions}\:{R}_{\mathrm{1}} \:\mathrm{and}\:{R}_{\mathrm{2}} \:\mathrm{such}\:\mathrm{that}\:{O}\:\mathrm{belongs} \\ $$$$\mathrm{to}\:{R}_{\mathrm{2}} .\:\mathrm{Let}\:{S}_{\mathrm{1}} \:\mathrm{be}\:\mathrm{a}\:\mathrm{circle}\:\mathrm{with}\:\mathrm{centre}\:\mathrm{in} \\ $$$${R}_{\mathrm{1}} ,\:\mathrm{touching}\:{AB}\:\mathrm{at}\:{X}\:\mathrm{and}\:{S}\:\mathrm{internally}. \\ $$$$\mathrm{Let}\:{S}_{\mathrm{2}} \:\mathrm{be}\:\mathrm{a}\:\mathrm{circle}\:\mathrm{with}\:\mathrm{centre}\:\mathrm{in}\:{R}_{\mathrm{2}} , \\ $$$$\mathrm{touching}\:{AB}\:\mathrm{at}\:{Y},\:\mathrm{the}\:\mathrm{circle}\:{S}\:\mathrm{internally} \\ $$$$\mathrm{and}\:\mathrm{passing}\:\mathrm{through}\:\mathrm{the}\:\mathrm{centre}\:\mathrm{of}\:{S}. \\ $$$$\mathrm{The}\:\mathrm{point}\:{X}\:\mathrm{lies}\:\mathrm{on}\:\mathrm{the}\:\mathrm{diameter} \\ $$$$\mathrm{passing}\:\mathrm{through}\:\mathrm{the}\:\mathrm{centre}\:\mathrm{of}\:{S}_{\mathrm{2}} \:\mathrm{and} \\ $$$$\angle{YXO}\:=\:\mathrm{30}°.\:\mathrm{If}\:\mathrm{the}\:\mathrm{radius}\:\mathrm{of}\:{S}_{\mathrm{2}} \:\mathrm{is}\:\mathrm{100} \\ $$$$\mathrm{then}\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{radius}\:\mathrm{of}\:{S}_{\mathrm{1}} ? \\ $$

Question Number 19668 Answers: 0 Comments: 2

Question Number 19659 Answers: 1 Comments: 0

((1+secθ)/(secθ))=((sin^(2 ) θ)/(1−cosθ))

$$\frac{\mathrm{1}+{sec}\theta}{{sec}\theta}=\frac{{sin}^{\mathrm{2}\:} \theta}{\mathrm{1}−{cos}\theta} \\ $$$$ \\ $$

Question Number 19415 Answers: 1 Comments: 0

PS is a line segment of length 4 and O is the midpoint of PS. A semicircular arc is drawn with PS as diameter. Let X be the midpoint of this arc. Q and R are points on the arc PXS such that QR is parallel to PS and the semicircular arc drawn with QR as diameter is tangent to PS. What is the area of the region QXROQ bounded by the two semicircular arcs?

$${PS}\:\mathrm{is}\:\mathrm{a}\:\mathrm{line}\:\mathrm{segment}\:\mathrm{of}\:\mathrm{length}\:\mathrm{4}\:\mathrm{and}\:{O} \\ $$$$\mathrm{is}\:\mathrm{the}\:\mathrm{midpoint}\:\mathrm{of}\:{PS}.\:\mathrm{A}\:\mathrm{semicircular} \\ $$$$\mathrm{arc}\:\mathrm{is}\:\mathrm{drawn}\:\mathrm{with}\:{PS}\:\mathrm{as}\:\mathrm{diameter}.\:\mathrm{Let} \\ $$$${X}\:\mathrm{be}\:\mathrm{the}\:\mathrm{midpoint}\:\mathrm{of}\:\mathrm{this}\:\mathrm{arc}.\:{Q}\:\mathrm{and}\:{R} \\ $$$$\mathrm{are}\:\mathrm{points}\:\mathrm{on}\:\mathrm{the}\:\mathrm{arc}\:{PXS}\:\mathrm{such}\:\mathrm{that}\:{QR} \\ $$$$\mathrm{is}\:\mathrm{parallel}\:\mathrm{to}\:{PS}\:\mathrm{and}\:\mathrm{the}\:\mathrm{semicircular} \\ $$$$\mathrm{arc}\:\mathrm{drawn}\:\mathrm{with}\:{QR}\:\mathrm{as}\:\mathrm{diameter}\:\mathrm{is} \\ $$$$\mathrm{tangent}\:\mathrm{to}\:{PS}.\:\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{region}\:{QXROQ}\:\mathrm{bounded}\:\mathrm{by}\:\mathrm{the}\:\mathrm{two} \\ $$$$\mathrm{semicircular}\:\mathrm{arcs}? \\ $$

Question Number 19394 Answers: 1 Comments: 0

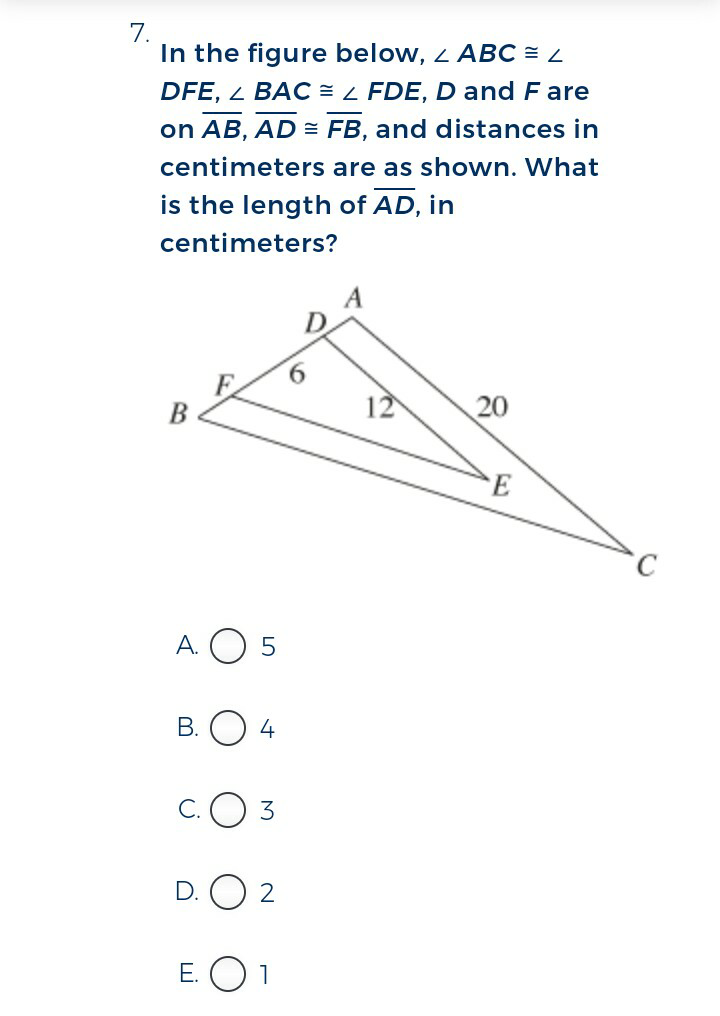
Question Number 19388 Answers: 0 Comments: 1

related to Q.19333 the side lengthes of a triangle are integer. if the perimeter of the triangle is 100, how many different triangles exist? what is the maximum area of them?

Question Number 19301 Answers: 1 Comments: 0

e^(iπ) +1=0

$${e}^{{i}\pi} +\mathrm{1}=\mathrm{0} \\ $$

Question Number 19293 Answers: 1 Comments: 3

Let AC be a line segment in the plane and B a point between A and C. Construct isosceles triangles PAB and QBC on one side of the segment AC such that ∠APB = ∠BQC = 120° and an isosceles triangle RAC on the other side of AC such that ∠ARC = 120°. Show that PQR is an equilateral triangle.

$$\mathrm{Let}\:{AC}\:\mathrm{be}\:\mathrm{a}\:\mathrm{line}\:\mathrm{segment}\:\mathrm{in}\:\mathrm{the}\:\mathrm{plane} \\ $$$$\mathrm{and}\:{B}\:\mathrm{a}\:\mathrm{point}\:\mathrm{between}\:{A}\:\mathrm{and}\:{C}. \\ $$$$\mathrm{Construct}\:\mathrm{isosceles}\:\mathrm{triangles}\:{PAB}\:\mathrm{and} \\ $$$${QBC}\:\mathrm{on}\:\mathrm{one}\:\mathrm{side}\:\mathrm{of}\:\mathrm{the}\:\mathrm{segment}\:{AC} \\ $$$$\mathrm{such}\:\mathrm{that}\:\angle{APB}\:=\:\angle{BQC}\:=\:\mathrm{120}°\:\mathrm{and} \\ $$$$\mathrm{an}\:\mathrm{isosceles}\:\mathrm{triangle}\:{RAC}\:\mathrm{on}\:\mathrm{the}\:\mathrm{other} \\ $$$$\mathrm{side}\:\mathrm{of}\:{AC}\:\mathrm{such}\:\mathrm{that}\:\angle{ARC}\:=\:\mathrm{120}°. \\ $$$$\mathrm{Show}\:\mathrm{that}\:{PQR}\:\mathrm{is}\:\mathrm{an}\:\mathrm{equilateral} \\ $$$$\mathrm{triangle}. \\ $$

Question Number 19279 Answers: 0 Comments: 0

lim_(x→π) (((2x)/(cot(1/x))))

$$\underset{{x}\rightarrow\pi} {\mathrm{lim}}\left(\frac{\mathrm{2}{x}}{{cot}\frac{\mathrm{1}}{{x}}}\right) \\ $$

Question Number 19150 Answers: 1 Comments: 4

A semicircle is tangent to both legs of a right triangle and has its centre on the hypotenuse. The hypotenuse is partitioned into 4 segments, with lengths 3, 12, 12, and x, as shown in the figure. Determine the value of ′x′.

$$\mathrm{A}\:\mathrm{semicircle}\:\mathrm{is}\:\mathrm{tangent}\:\mathrm{to}\:\mathrm{both}\:\mathrm{legs}\:\mathrm{of}\:\mathrm{a} \\ $$$$\mathrm{right}\:\mathrm{triangle}\:\mathrm{and}\:\mathrm{has}\:\mathrm{its}\:\mathrm{centre}\:\mathrm{on}\:\mathrm{the} \\ $$$$\mathrm{hypotenuse}.\:\mathrm{The}\:\mathrm{hypotenuse}\:\mathrm{is} \\ $$$$\mathrm{partitioned}\:\mathrm{into}\:\mathrm{4}\:\mathrm{segments},\:\mathrm{with}\:\mathrm{lengths} \\ $$$$\mathrm{3},\:\mathrm{12},\:\mathrm{12},\:\mathrm{and}\:{x},\:\mathrm{as}\:\mathrm{shown}\:\mathrm{in}\:\mathrm{the}\:\mathrm{figure}. \\ $$$$\mathrm{Determine}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:'{x}'. \\ $$

Question Number 19104 Answers: 1 Comments: 1

Let ABC be an acute-angled triangle with AC ≠ BC and let O be the circumcenter and F be the foot of altitude through C. Further, let X and Y be the feet of perpendiculars dropped from A and B respectively to (the extension of) CO. The line FO intersects the circumcircle of ΔFXY, second time at P. Prove that OP < OF.

$$\mathrm{Let}\:\mathrm{ABC}\:\mathrm{be}\:\mathrm{an}\:\mathrm{acute}-\mathrm{angled}\:\mathrm{triangle} \\ $$$$\mathrm{with}\:\mathrm{AC}\:\neq\:\mathrm{BC}\:\mathrm{and}\:\mathrm{let}\:\mathrm{O}\:\mathrm{be}\:\mathrm{the} \\ $$$$\mathrm{circumcenter}\:\mathrm{and}\:\mathrm{F}\:\mathrm{be}\:\mathrm{the}\:\mathrm{foot}\:\mathrm{of} \\ $$$$\mathrm{altitude}\:\mathrm{through}\:\mathrm{C}.\:\mathrm{Further},\:\mathrm{let}\:\mathrm{X}\:\mathrm{and}\:\mathrm{Y} \\ $$$$\mathrm{be}\:\mathrm{the}\:\mathrm{feet}\:\mathrm{of}\:\mathrm{perpendiculars}\:\mathrm{dropped} \\ $$$$\mathrm{from}\:\mathrm{A}\:\mathrm{and}\:\mathrm{B}\:\mathrm{respectively}\:\mathrm{to}\:\left(\mathrm{the}\right. \\ $$$$\left.\mathrm{extension}\:\mathrm{of}\right)\:\mathrm{CO}.\:\mathrm{The}\:\mathrm{line}\:\mathrm{FO}\:\mathrm{intersects} \\ $$$$\mathrm{the}\:\mathrm{circumcircle}\:\mathrm{of}\:\Delta\mathrm{FXY},\:\mathrm{second}\:\mathrm{time} \\ $$$$\mathrm{at}\:\mathrm{P}.\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{OP}\:<\:\mathrm{OF}. \\ $$

Question Number 18801 Answers: 0 Comments: 1

Question Number 18799 Answers: 1 Comments: 0

Question Number 18783 Answers: 1 Comments: 0

∫sin x

$$ \\ $$$$\int\mathrm{sin}\:{x} \\ $$

Question Number 18967 Answers: 0 Comments: 3

Let PQRS be a rectangle such that PQ = a and QR = b. Suppose r_1 is the radius of the circle passing through P and Q and touching RS and r_2 is the radius of the circle passing through Q and R and touching PS. Show that : 5(a + b) ≤ 8(r_1 + r_2 )

$$\mathrm{Let}\:\mathrm{PQRS}\:\mathrm{be}\:\mathrm{a}\:\mathrm{rectangle}\:\mathrm{such}\:\mathrm{that} \\ $$$$\mathrm{PQ}\:=\:{a}\:\mathrm{and}\:\mathrm{QR}\:=\:{b}.\:\mathrm{Suppose}\:\mathrm{r}_{\mathrm{1}} \:\mathrm{is}\:\mathrm{the} \\ $$$$\mathrm{radius}\:\mathrm{of}\:\mathrm{the}\:\mathrm{circle}\:\mathrm{passing}\:\mathrm{through}\:\mathrm{P} \\ $$$$\mathrm{and}\:\mathrm{Q}\:\mathrm{and}\:\mathrm{touching}\:\mathrm{RS}\:\mathrm{and}\:\mathrm{r}_{\mathrm{2}} \:\mathrm{is}\:\mathrm{the} \\ $$$$\mathrm{radius}\:\mathrm{of}\:\mathrm{the}\:\mathrm{circle}\:\mathrm{passing}\:\mathrm{through}\:\mathrm{Q} \\ $$$$\mathrm{and}\:\mathrm{R}\:\mathrm{and}\:\mathrm{touching}\:\mathrm{PS}.\:\mathrm{Show}\:\mathrm{that}\:: \\ $$$$\mathrm{5}\left({a}\:+\:{b}\right)\:\leqslant\:\mathrm{8}\left(\mathrm{r}_{\mathrm{1}} \:+\:\mathrm{r}_{\mathrm{2}} \right) \\ $$

Question Number 18681 Answers: 1 Comments: 0

y = ∣sin x∣ + 2 y = ∣x∣ + 2 −π −π ≤ x ≤ π Find the area that have created from the equations above

$${y}\:=\:\mid\mathrm{sin}\:{x}\mid\:+\:\mathrm{2} \\ $$$${y}\:=\:\mid{x}\mid\:+\:\mathrm{2}\:−\pi \\ $$$$−\pi\:\leqslant\:{x}\:\leqslant\:\pi \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{area}\:\mathrm{that}\:\mathrm{have}\:\mathrm{created} \\ $$$$\mathrm{from}\:\mathrm{the}\:\mathrm{equations}\:\mathrm{above} \\ $$

Question Number 18968 Answers: 1 Comments: 1

Find the side lengths of a triangle if side lengths are consecutive integers,and one of whose angles is twice as large as another.

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{side}\:\mathrm{lengths}\:\mathrm{of}\:\mathrm{a}\:\mathrm{triangle} \\ $$$$\mathrm{if}\:\mathrm{side}\:\mathrm{lengths}\:\mathrm{are}\:\mathrm{consecutive}\: \\ $$$$\mathrm{integers},\mathrm{and}\:\mathrm{one}\:\mathrm{of}\:\mathrm{whose}\:\mathrm{angles} \\ $$$$\mathrm{is}\:\mathrm{twice}\:\mathrm{as}\:\mathrm{large}\:\mathrm{as}\:\mathrm{another}. \\ $$

Question Number 18625 Answers: 1 Comments: 1

x−5×+3=7

$$\mathrm{x}−\mathrm{5}×+\mathrm{3}=\mathrm{7} \\ $$

Question Number 19238 Answers: 0 Comments: 4

Let ABCD be a parallelogram. Two points E and F are chosen on the sides BC and CD, respectively, such that ((EB)/(EC)) = m, and ((FC)/(FD)) = n. Lines AE and BF intersect at G. Prove that the ratio ((AG)/(GE)) = (((m + 1)(n + 1))/(mn)).

$$\mathrm{Let}\:{ABCD}\:\mathrm{be}\:\mathrm{a}\:\mathrm{parallelogram}.\:\mathrm{Two} \\ $$$$\mathrm{points}\:{E}\:\mathrm{and}\:{F}\:\mathrm{are}\:\mathrm{chosen}\:\mathrm{on}\:\mathrm{the}\:\mathrm{sides} \\ $$$${BC}\:\mathrm{and}\:{CD},\:\mathrm{respectively},\:\mathrm{such}\:\mathrm{that} \\ $$$$\frac{{EB}}{{EC}}\:=\:{m},\:\mathrm{and}\:\frac{{FC}}{{FD}}\:=\:{n}.\:\mathrm{Lines}\:{AE}\:\mathrm{and}\:{BF} \\ $$$$\mathrm{intersect}\:\mathrm{at}\:{G}.\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{the}\:\mathrm{ratio} \\ $$$$\frac{{AG}}{{GE}}\:=\:\frac{\left({m}\:+\:\mathrm{1}\right)\left({n}\:+\:\mathrm{1}\right)}{{mn}}. \\ $$

Pg 102 Pg 103 Pg 104 Pg 105 Pg 106 Pg 107 Pg 108 Pg 109 Pg 110 Pg 111

Contact: info@tinkutara.com