Question and Answers Forum

GeometryQuestion and Answers: Page 106

Question Number 22055 Answers: 0 Comments: 1

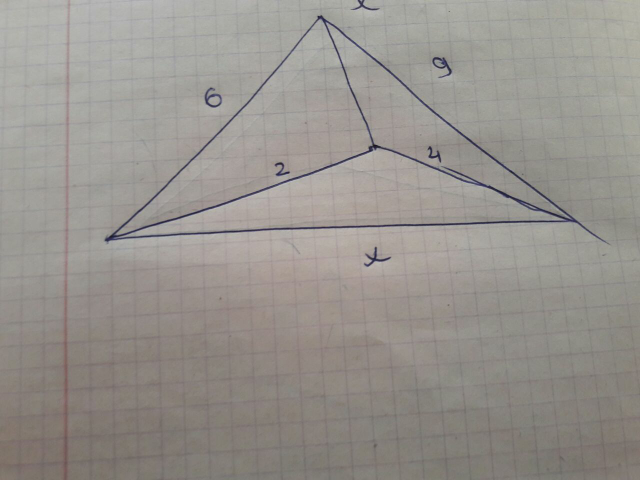
Question Number 22001 Answers: 1 Comments: 0

if determinant (((z−(z/4))),())=2 then value of determinant ((z),())

$${if}\:\begin{vmatrix}{{z}−\frac{{z}}{\mathrm{4}}}\\{}\end{vmatrix}=\mathrm{2}\:{then}\:{value}\:{of}\:\begin{vmatrix}{{z}}\\{}\end{vmatrix} \\ $$

Question Number 21973 Answers: 1 Comments: 1

ΔABC with sides a, b, c ∈ Z and ∠A = 3∠B If its circumference is minimum, then find a, b, c

$$\Delta{ABC}\:\mathrm{with}\:\mathrm{sides}\:{a},\:{b},\:{c}\:\in\:\mathbb{Z}\:\mathrm{and}\:\angle{A}\:=\:\mathrm{3}\angle{B} \\ $$$$\mathrm{If}\:\mathrm{its}\:\mathrm{circumference}\:\mathrm{is}\:\mathrm{minimum},\:\mathrm{then} \\ $$$$\mathrm{find}\:{a},\:{b},\:{c} \\ $$

Question Number 21903 Answers: 0 Comments: 0

ΔABC with sides a, b, c ∈ Z and ∠A = 3∠B If its circumference is minimum, then find a, b, c

Question Number 21840 Answers: 1 Comments: 0

A cone is placed inside a sphere. If volume of the cone is maximum, find the ratio of radius from the cone and sphere

$$\mathrm{A}\:\mathrm{cone}\:\mathrm{is}\:\mathrm{placed}\:\mathrm{inside}\:\mathrm{a}\:\mathrm{sphere}. \\ $$$$\mathrm{If}\:\mathrm{volume}\:\mathrm{of}\:\mathrm{the}\:\mathrm{cone}\:\mathrm{is}\:\mathrm{maximum}, \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{ratio}\:\mathrm{of}\:\mathrm{radius}\:\mathrm{from}\:\mathrm{the}\:\mathrm{cone}\:\mathrm{and}\:\mathrm{sphere} \\ $$

Question Number 21825 Answers: 0 Comments: 2

Find the simplest form of Σ_(k = 1) ^n 2^k [sin^2 (((2kπ)/3)) + (1/4)]

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{simplest}\:\mathrm{form}\:\mathrm{of} \\ $$$$\underset{{k}\:=\:\mathrm{1}} {\overset{{n}} {\sum}}\mathrm{2}^{{k}} \left[\mathrm{sin}^{\mathrm{2}} \:\left(\frac{\mathrm{2}{k}\pi}{\mathrm{3}}\right)\:+\:\frac{\mathrm{1}}{\mathrm{4}}\right] \\ $$

Question Number 21754 Answers: 1 Comments: 0

Q. In 2014, country X had 783 miles of paved roads. Starting in 2015, the country has been building 8 miles of new paved roads each year. At this rate, how many miles of paved roads will country X have in 2030?

$$\mathrm{Q}.\:\mathrm{In}\:\mathrm{2014},\:\mathrm{country}\:\mathrm{X}\:\mathrm{had}\:\mathrm{783}\:\mathrm{miles}\:\mathrm{of}\:\mathrm{paved}\:\mathrm{roads}.\:\mathrm{Starting}\:\mathrm{in}\: \\ $$$$\:\:\:\:\:\:\:\mathrm{2015},\:\mathrm{the}\:\mathrm{country}\:\mathrm{has}\:\:\mathrm{been}\:\mathrm{building}\:\:\mathrm{8}\:\:\mathrm{miles}\:\mathrm{of}\:\mathrm{new}\:\mathrm{paved} \\ $$$$\:\:\:\:\:\:\mathrm{roads}\:\mathrm{each}\:\mathrm{year}.\:\mathrm{At}\:\mathrm{this}\:\mathrm{rate},\:\:\mathrm{how}\:\:\mathrm{many}\:\mathrm{miles}\:\mathrm{of}\:\mathrm{paved}\:\mathrm{roads} \\ $$$$\:\:\:\:\:\:\mathrm{will}\:\mathrm{country}\:\mathrm{X}\:\mathrm{have}\:\mathrm{in}\:\mathrm{2030}? \\ $$

Question Number 21782 Answers: 0 Comments: 0

a_1 =1, a_(n+1) =(a_n /(√(a_n +n+1))) Σ_(n=1) ^∞ a_n =?

$${a}_{\mathrm{1}} =\mathrm{1},\:{a}_{{n}+\mathrm{1}} =\frac{{a}_{{n}} }{\sqrt{{a}_{{n}} +{n}+\mathrm{1}}} \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{a}_{{n}} =? \\ $$

Question Number 21656 Answers: 0 Comments: 4

Let A(x) is a cubic polynomial and B(x) = (x −1)(x − 2)(x − 3) Find how many C(x) so that B(C(x)) = B(x) . A(x)

$$\mathrm{Let}\:{A}\left({x}\right)\:\mathrm{is}\:\mathrm{a}\:\mathrm{cubic}\:\mathrm{polynomial}\:\mathrm{and}\:{B}\left({x}\right)\:=\:\left({x}\:−\mathrm{1}\right)\left({x}\:−\:\mathrm{2}\right)\left({x}\:−\:\mathrm{3}\right) \\ $$$$\mathrm{Find}\:\mathrm{how}\:\mathrm{many}\:{C}\left({x}\right)\:\mathrm{so}\:\mathrm{that} \\ $$$${B}\left({C}\left({x}\right)\right)\:=\:{B}\left({x}\right)\:.\:{A}\left({x}\right) \\ $$

Question Number 21655 Answers: 1 Comments: 0

(((2017)),(( 0)) ) + (((2017)),(( 2)) ) + (((2017)),(( 4)) ) + (((2017)),(( 6)) ) + ... + (((2017)),((2016)) ) is equal to ...

$$\begin{pmatrix}{\mathrm{2017}}\\{\:\:\:\:\mathrm{0}}\end{pmatrix}\:+\:\begin{pmatrix}{\mathrm{2017}}\\{\:\:\:\:\mathrm{2}}\end{pmatrix}\:+\:\begin{pmatrix}{\mathrm{2017}}\\{\:\:\:\:\mathrm{4}}\end{pmatrix}\:+\:\begin{pmatrix}{\mathrm{2017}}\\{\:\:\:\:\mathrm{6}}\end{pmatrix}\:+\:...\:+\:\begin{pmatrix}{\mathrm{2017}}\\{\mathrm{2016}}\end{pmatrix} \\ $$$$\mathrm{is}\:\mathrm{equal}\:\mathrm{to}\:... \\ $$

Question Number 21611 Answers: 1 Comments: 0

Sin5° =?

$$\mathrm{Sin5}°\:=? \\ $$

Question Number 21574 Answers: 1 Comments: 0

The cyclic octagon ABCDEFGH has sides a, a, a, a, b, b, b, b respectively. Find the radius of the circle that circumscribes ABCDEFGH in terms of a and b.

$$\mathrm{The}\:\mathrm{cyclic}\:\mathrm{octagon}\:{ABCDEFGH}\:\mathrm{has} \\ $$$$\mathrm{sides}\:{a},\:{a},\:{a},\:{a},\:{b},\:{b},\:{b},\:{b}\:\mathrm{respectively}. \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{radius}\:\mathrm{of}\:\mathrm{the}\:\mathrm{circle}\:\mathrm{that} \\ $$$$\mathrm{circumscribes}\:{ABCDEFGH}\:\mathrm{in}\:\mathrm{terms} \\ $$$$\mathrm{of}\:{a}\:\mathrm{and}\:{b}. \\ $$

Question Number 21571 Answers: 1 Comments: 0

ABCD is a cyclic quadrilateral; x, y, z are the distances of A from the lines BD, BC, CD respectively. Prove that ((BD)/x) = ((BC)/y) + ((CD)/z).

$${ABCD}\:\mathrm{is}\:\mathrm{a}\:\mathrm{cyclic}\:\mathrm{quadrilateral};\:{x},\:{y},\:{z} \\ $$$$\mathrm{are}\:\mathrm{the}\:\mathrm{distances}\:\mathrm{of}\:{A}\:\mathrm{from}\:\mathrm{the}\:\mathrm{lines} \\ $$$${BD},\:{BC},\:{CD}\:\mathrm{respectively}.\:\mathrm{Prove}\:\mathrm{that} \\ $$$$\frac{{BD}}{{x}}\:=\:\frac{{BC}}{{y}}\:+\:\frac{{CD}}{{z}}. \\ $$

Question Number 21490 Answers: 1 Comments: 0

The kinetic energy of a body increases by 100%.Find the % increase in its momentum. please solve with explanations where necessary. Thanks.

$$\mathrm{The}\:\mathrm{kinetic}\:\mathrm{energy}\:\mathrm{of}\:\mathrm{a}\:\mathrm{body} \\ $$$$\mathrm{increases}\:\mathrm{by}\:\mathrm{100\%}.\mathrm{Find}\:\mathrm{the}\:\% \\ $$$$\mathrm{increase}\:\mathrm{in}\:\mathrm{its}\:\mathrm{momentum}. \\ $$$$ \\ $$$$\mathrm{please}\:\mathrm{solve}\:\mathrm{with}\:\mathrm{explanations} \\ $$$$\mathrm{where}\:\mathrm{necessary}.\:\mathrm{Thanks}. \\ $$

Question Number 21401 Answers: 0 Comments: 0

∫_0 ^∞ ((xdx)/(e^x +1))=?

$$\underset{\mathrm{0}} {\overset{\infty} {\int}}\frac{{xdx}}{{e}^{{x}} +\mathrm{1}}=? \\ $$

Question Number 21310 Answers: 1 Comments: 8

What do you guys think of creating a Telegram group to discuss theory and more descriptive questions?

$$\mathrm{What}\:\mathrm{do}\:\mathrm{you}\:\mathrm{guys}\:\mathrm{think}\:\mathrm{of} \\ $$$$\mathrm{creating}\:\mathrm{a}\:\mathrm{Telegram}\:\mathrm{group}\:\mathrm{to} \\ $$$$\mathrm{discuss}\:\mathrm{theory}\:\mathrm{and}\:\mathrm{more}\:\mathrm{descriptive} \\ $$$$\mathrm{questions}? \\ $$

Question Number 21231 Answers: 0 Comments: 0

Consider the areas of the four triangles obtained by drawing the diagonals AC and BD of a trapezium ABCD. The product of these areas, taken two at time, are computed. If among the six products so obtained, two products are 1296 and 576, determine the square root of the maximum possible area of the trapezium to the nearest integer.

Question Number 21228 Answers: 0 Comments: 0

Let P be an interior point of a triangle ABC whose sidelengths are 26, 65, 78. The line through P parallel to BC meets AB in K and AC in L. The line through P parallel to CA meets BC in M and BA in N. The line through P parallel to AB meets CA in S and CB in T. If KL, MN, ST, are of equal lengths, find this common length.

$$\mathrm{Let}\:{P}\:\mathrm{be}\:\mathrm{an}\:\mathrm{interior}\:\mathrm{point}\:\mathrm{of}\:\mathrm{a}\:\mathrm{triangle} \\ $$$${ABC}\:\mathrm{whose}\:\mathrm{sidelengths}\:\mathrm{are}\:\mathrm{26},\:\mathrm{65},\:\mathrm{78}. \\ $$$$\mathrm{The}\:\mathrm{line}\:\mathrm{through}\:{P}\:\mathrm{parallel}\:\mathrm{to}\:{BC}\:\mathrm{meets} \\ $$$${AB}\:\mathrm{in}\:{K}\:\mathrm{and}\:{AC}\:\mathrm{in}\:{L}.\:\mathrm{The}\:\mathrm{line}\:\mathrm{through} \\ $$$${P}\:\mathrm{parallel}\:\mathrm{to}\:{CA}\:\mathrm{meets}\:{BC}\:\mathrm{in}\:{M}\:\mathrm{and}\:{BA} \\ $$$$\mathrm{in}\:{N}.\:\mathrm{The}\:\mathrm{line}\:\mathrm{through}\:{P}\:\mathrm{parallel}\:\mathrm{to}\:{AB} \\ $$$$\mathrm{meets}\:{CA}\:\mathrm{in}\:{S}\:\mathrm{and}\:{CB}\:\mathrm{in}\:{T}.\:\mathrm{If}\:{KL},\:{MN}, \\ $$$${ST},\:\mathrm{are}\:\mathrm{of}\:\mathrm{equal}\:\mathrm{lengths},\:\mathrm{find}\:\mathrm{this} \\ $$$$\mathrm{common}\:\mathrm{length}. \\ $$

Question Number 21001 Answers: 0 Comments: 0

determinant (((a 1 1)),((1 b 1)),((1 1 c)))>0 then showthat abc>−8−99

$$\begin{vmatrix}{{a}\:\mathrm{1}\:\mathrm{1}}\\{\mathrm{1}\:{b}\:\mathrm{1}}\\{\mathrm{1}\:\mathrm{1}\:{c}}\end{vmatrix}>\mathrm{0}\:{then}\:{showthat}\:{abc}>−\mathrm{8}−\mathrm{99} \\ $$

Question Number 20953 Answers: 0 Comments: 2

Question Number 20944 Answers: 1 Comments: 0

Question Number 20599 Answers: 1 Comments: 0

In a rectangle ABCD, E is the midpoint of AB; F is a point on AC such that BF is perpendicular to AC; and FE perpendicular to BD. Suppose BC = 8(√3). Find AB.

$$\mathrm{In}\:\mathrm{a}\:\mathrm{rectangle}\:{ABCD},\:{E}\:\mathrm{is}\:\mathrm{the}\:\mathrm{midpoint} \\ $$$$\mathrm{of}\:{AB};\:{F}\:\mathrm{is}\:\mathrm{a}\:\mathrm{point}\:\mathrm{on}\:{AC}\:\mathrm{such}\:\mathrm{that}\:{BF} \\ $$$$\mathrm{is}\:\mathrm{perpendicular}\:\mathrm{to}\:{AC};\:\mathrm{and}\:{FE} \\ $$$$\mathrm{perpendicular}\:\mathrm{to}\:{BD}.\:\mathrm{Suppose}\:{BC}\:=\:\mathrm{8}\sqrt{\mathrm{3}}. \\ $$$$\mathrm{Find}\:{AB}. \\ $$

Question Number 20545 Answers: 0 Comments: 1

Let ABC be an acute-angled triangle with AC ≠ BC and let O be the circumcenter and F be the foot of altitude through C. Further, let X and Y be the feet of perpendiculars dropped from A and B respectively to (the extension of) CO. Prove that FY ⊥ CA using that ∠CFY = ∠CBY = ∠CAF.

$$\mathrm{Let}\:\mathrm{ABC}\:\mathrm{be}\:\mathrm{an}\:\mathrm{acute}-\mathrm{angled}\:\mathrm{triangle} \\ $$$$\mathrm{with}\:\mathrm{AC}\:\neq\:\mathrm{BC}\:\mathrm{and}\:\mathrm{let}\:\mathrm{O}\:\mathrm{be}\:\mathrm{the} \\ $$$$\mathrm{circumcenter}\:\mathrm{and}\:\mathrm{F}\:\mathrm{be}\:\mathrm{the}\:\mathrm{foot}\:\mathrm{of} \\ $$$$\mathrm{altitude}\:\mathrm{through}\:\mathrm{C}.\:\mathrm{Further},\:\mathrm{let}\:\mathrm{X}\:\mathrm{and} \\ $$$$\mathrm{Y}\:\mathrm{be}\:\mathrm{the}\:\mathrm{feet}\:\mathrm{of}\:\mathrm{perpendiculars}\:\mathrm{dropped} \\ $$$$\mathrm{from}\:\mathrm{A}\:\mathrm{and}\:\mathrm{B}\:\mathrm{respectively}\:\mathrm{to}\:\left(\mathrm{the}\right. \\ $$$$\left.\mathrm{extension}\:\mathrm{of}\right)\:\mathrm{CO}.\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{FY}\:\bot\:\mathrm{CA} \\ $$$$\mathrm{using}\:\mathrm{that}\:\angle\mathrm{CFY}\:=\:\angle\mathrm{CBY}\:=\:\angle\mathrm{CAF}. \\ $$

Question Number 20187 Answers: 0 Comments: 1

t_n =(t_(n−1) /n^2 ), t_1 =3;t_2 ,t_3 ,(n≥2)

$${t}_{{n}} =\frac{{t}_{{n}−\mathrm{1}} }{{n}^{\mathrm{2}} },\:{t}_{\mathrm{1}} =\mathrm{3};{t}_{\mathrm{2}} ,{t}_{\mathrm{3}} ,\left({n}\geqslant\mathrm{2}\right) \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Question Number 20156 Answers: 1 Comments: 0

Solve: inverse laplace. L^(−1) ((s/(s^(2 ) + 6s + 25)))

$$\mathrm{Solve}:\:\mathrm{inverse}\:\mathrm{laplace}.\:\:\:\:\mathrm{L}^{−\mathrm{1}} \left(\frac{\mathrm{s}}{\mathrm{s}^{\mathrm{2}\:} +\:\mathrm{6s}\:+\:\mathrm{25}}\right) \\ $$

Question Number 20131 Answers: 1 Comments: 4

In rectangle ABCD,AB=8, BC=20.P is a point on AD so that ∠BPC=90°.If r_1 ,r_2 ,r_3 are the radii of the incircles of APB, BPC, and CPD. find r_1 +r_2 +r_3

$${In}\:{rectangle}\:{ABCD},{AB}=\mathrm{8}, \\ $$$${BC}=\mathrm{20}.{P}\:{is}\:{a}\:{point}\:{on}\:{AD}\:{so} \\ $$$${that}\:\angle{BPC}=\mathrm{90}°.{If}\:{r}_{\mathrm{1}} ,{r}_{\mathrm{2}} ,{r}_{\mathrm{3}} \:{are}\:{the} \\ $$$${radii}\:{of}\:{the}\:{incircles}\:{of}\:{APB}, \\ $$$${BPC},\:{and}\:{CPD}.\:{find}\:{r}_{\mathrm{1}} +{r}_{\mathrm{2}} +{r}_{\mathrm{3}} \\ $$

Pg 101 Pg 102 Pg 103 Pg 104 Pg 105 Pg 106 Pg 107 Pg 108 Pg 109 Pg 110

Contact: info@tinkutara.com