Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 46481 by MrW3 last updated on 27/Oct/18

Four spheres with radii a,b,c and d touch each other. Find the radii of their circumscribed sphere (R) and their inscribed sphere (r) in terms of a,b,c and d.

$${Four}\:{spheres}\:{with}\:{radii}\:{a},{b},{c}\:{and}\:{d} \\ $$$${touch}\:{each}\:{other}.\:{Find}\:{the}\:{radii}\:{of} \\ $$$${their}\:{circumscribed}\:{sphere}\:\left({R}\right)\:{and} \\ $$$${their}\:{inscribed}\:{sphere}\:\left({r}\right)\:{in}\:{terms}\:{of}\: \\ $$$${a},{b},{c}\:{and}\:{d}. \\ $$

Commented by MJS last updated on 27/Oct/18

plenty of cases. 3 spheres similar to 3 circles: 3^(rd) one could be very small compared to the 2 others, then the 4^(th) is still possible but no circumscribed sphere could exist, even not any sphere or plain touching the 4 spheres from the outside. inscribed sphere doesn′t exist in some cases too. imagine three spheres: their centers are in a plain; the 4^(th) one could exactly fit into the gap between them.

$$\mathrm{plenty}\:\mathrm{of}\:\mathrm{cases}.\:\mathrm{3}\:\mathrm{spheres}\:\mathrm{similar}\:\mathrm{to}\:\mathrm{3}\:\mathrm{circles}: \\ $$$$\mathrm{3}^{\mathrm{rd}} \:\mathrm{one}\:\mathrm{could}\:\mathrm{be}\:\mathrm{very}\:\mathrm{small}\:\mathrm{compared}\:\mathrm{to}\:\mathrm{the} \\ $$$$\mathrm{2}\:\mathrm{others},\:\mathrm{then}\:\mathrm{the}\:\mathrm{4}^{\mathrm{th}} \:\mathrm{is}\:\mathrm{still}\:\mathrm{possible}\:\mathrm{but}\:\mathrm{no} \\ $$$$\mathrm{circumscribed}\:\mathrm{sphere}\:\mathrm{could}\:\mathrm{exist},\:\mathrm{even}\:\mathrm{not} \\ $$$$\mathrm{any}\:\mathrm{sphere}\:\mathrm{or}\:\mathrm{plain}\:\mathrm{touching}\:\mathrm{the}\:\mathrm{4}\:\mathrm{spheres} \\ $$$$\mathrm{from}\:\mathrm{the}\:\mathrm{outside}. \\ $$$$\mathrm{inscribed}\:\mathrm{sphere}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{exist}\:\mathrm{in}\:\mathrm{some}\:\mathrm{cases} \\ $$$$\mathrm{too}.\:\mathrm{imagine}\:\mathrm{three}\:\mathrm{spheres}:\:\mathrm{their}\:\mathrm{centers} \\ $$$$\mathrm{are}\:\mathrm{in}\:\mathrm{a}\:\mathrm{plain};\:\mathrm{the}\:\mathrm{4}^{\mathrm{th}} \:\mathrm{one}\:\mathrm{could}\:\mathrm{exactly}\:\mathrm{fit} \\ $$$$\mathrm{into}\:\mathrm{the}\:\mathrm{gap}\:\mathrm{between}\:\mathrm{them}. \\ $$

Commented by MrW3 last updated on 27/Oct/18

thank you sir! generally you are right. but one can treat at first the case that such a sphere exists. from the result one can then see what is the condition that such a sphere exists. let′s come back to the question with three circles. from the results R=(1/(2(√((1/(ab))+(1/(bc))+(1/(ca))))−((1/a)+(1/b)+(1/c)))) r=(1/(2(√((1/(ab))+(1/(bc))+(1/(ca))))+(1/a)+(1/b)+(1/c))) we can see that the inscribed circle always exits since we get a valuefor r with any values of a,b and c. but the circumcircle doesn′t always exist. if 2(√((1/(ab))+(1/(bc))+(1/(ca))))=(1/a)+(1/b)+(1/c) there is no circumcircle at all, since R=∞. and if 2(√((1/(ab))+(1/(bc))+(1/(ca))))<(1/a)+(1/b)+(1/c) there is a “circumcircle”, but it tangents the three circles from outside, since in this case the value of R is negative. so my opinion is that we should start with the general case and then see which exceptions there are.

$${thank}\:{you}\:{sir}! \\ $$$${generally}\:{you}\:{are}\:{right}. \\ $$$${but}\:{one}\:{can}\:{treat}\:{at}\:{first}\:{the}\:{case}\:{that}\:{such} \\ $$$${a}\:{sphere}\:{exists}.\:{from}\:{the}\:{result}\:{one}\:{can} \\ $$$${then}\:{see}\:{what}\:{is}\:{the}\:{condition}\:{that}\:{such} \\ $$$${a}\:{sphere}\:{exists}.\:{let}'{s}\:{come}\:{back}\:{to}\:{the} \\ $$$${question}\:{with}\:{three}\:{circles}.\:{from}\:{the} \\ $$$${results} \\ $$$${R}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\frac{\mathrm{1}}{{ab}}+\frac{\mathrm{1}}{{bc}}+\frac{\mathrm{1}}{{ca}}}−\left(\frac{\mathrm{1}}{{a}}+\frac{\mathrm{1}}{{b}}+\frac{\mathrm{1}}{{c}}\right)} \\ $$$${r}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\frac{\mathrm{1}}{{ab}}+\frac{\mathrm{1}}{{bc}}+\frac{\mathrm{1}}{{ca}}}+\frac{\mathrm{1}}{{a}}+\frac{\mathrm{1}}{{b}}+\frac{\mathrm{1}}{{c}}} \\ $$$${we}\:{can}\:{see}\:{that}\:{the}\:{inscribed}\:{circle} \\ $$$${always}\:{exits}\:{since}\:{we}\:{get}\:{a}\:{valuefor} \\ $$$${r}\:{with}\:{any}\:{values}\:{of}\:{a},{b}\:{and}\:{c}.\:{but} \\ $$$${the}\:{circumcircle}\:{doesn}'{t}\:{always}\:{exist}. \\ $$$${if}\:\mathrm{2}\sqrt{\frac{\mathrm{1}}{{ab}}+\frac{\mathrm{1}}{{bc}}+\frac{\mathrm{1}}{{ca}}}=\frac{\mathrm{1}}{{a}}+\frac{\mathrm{1}}{{b}}+\frac{\mathrm{1}}{{c}}\:{there}\:{is} \\ $$$${no}\:{circumcircle}\:{at}\:{all},\:{since}\:{R}=\infty.\:{and} \\ $$$${if}\:\mathrm{2}\sqrt{\frac{\mathrm{1}}{{ab}}+\frac{\mathrm{1}}{{bc}}+\frac{\mathrm{1}}{{ca}}}<\frac{\mathrm{1}}{{a}}+\frac{\mathrm{1}}{{b}}+\frac{\mathrm{1}}{{c}}\:{there}\:{is} \\ $$$${a}\:``{circumcircle}'',\:{but}\:{it}\:{tangents}\:{the} \\ $$$${three}\:{circles}\:{from}\:{outside},\:{since}\:{in}\:{this} \\ $$$${case}\:{the}\:{value}\:{of}\:{R}\:{is}\:{negative}. \\ $$$$ \\ $$$${so}\:{my}\:{opinion}\:{is}\:{that}\:{we}\:{should}\:{start} \\ $$$${with}\:{the}\:{general}\:{case}\:{and}\:{then}\:{see} \\ $$$${which}\:{exceptions}\:{there}\:{are}. \\ $$

Commented by MJS last updated on 27/Oct/18

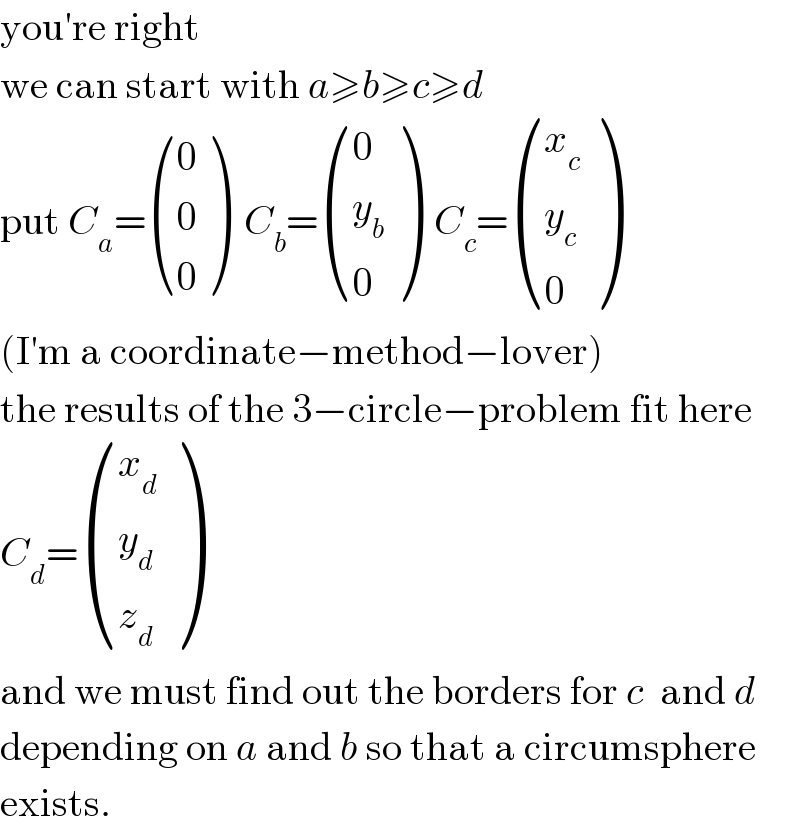
you′re right we can start with a≥b≥c≥d put C_a = ((0),(0),(0) ) C_b = ((0),(y_b ),(0) ) C_c = ((x_c ),(y_c ),(0) ) (I′m a coordinate−method−lover) the results of the 3−circle−problem fit here C_d = ((x_d ),(y_d ),(z_d ) ) and we must find out the borders for c and d depending on a and b so that a circumsphere exists.

$$\mathrm{you}'\mathrm{re}\:\mathrm{right} \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{start}\:\mathrm{with}\:{a}\geqslant{b}\geqslant{c}\geqslant{d} \\ $$$$\mathrm{put}\:{C}_{{a}} =\begin{pmatrix}{\mathrm{0}}\\{\mathrm{0}}\\{\mathrm{0}}\end{pmatrix}\:\:{C}_{{b}} =\begin{pmatrix}{\mathrm{0}}\\{{y}_{{b}} }\\{\mathrm{0}}\end{pmatrix}\:\:{C}_{{c}} =\begin{pmatrix}{{x}_{{c}} }\\{{y}_{{c}} }\\{\mathrm{0}}\end{pmatrix} \\ $$$$\left(\mathrm{I}'\mathrm{m}\:\mathrm{a}\:\mathrm{coordinate}−\mathrm{method}−\mathrm{lover}\right) \\ $$$$\mathrm{the}\:\mathrm{results}\:\mathrm{of}\:\mathrm{the}\:\mathrm{3}−\mathrm{circle}−\mathrm{problem}\:\mathrm{fit}\:\mathrm{here} \\ $$$${C}_{{d}} =\begin{pmatrix}{{x}_{{d}} }\\{{y}_{{d}} }\\{{z}_{{d}} }\end{pmatrix} \\ $$$$\mathrm{and}\:\mathrm{we}\:\mathrm{must}\:\mathrm{find}\:\mathrm{out}\:\mathrm{the}\:\mathrm{borders}\:\mathrm{for}\:{c}\:\:\mathrm{and}\:{d} \\ $$$$\mathrm{depending}\:\mathrm{on}\:{a}\:\mathrm{and}\:{b}\:\mathrm{so}\:\mathrm{that}\:\mathrm{a}\:\mathrm{circumsphere} \\ $$$$\mathrm{exists}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com