
Question Number 114192 by ZiYangLee last updated on 17/Sep/20
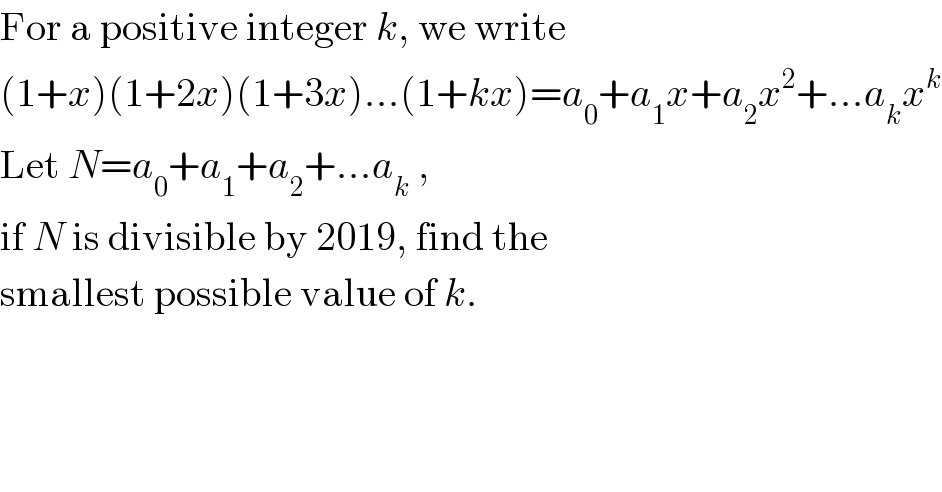
$$\mathrm{For}\:\mathrm{a}\:\mathrm{positive}\:\mathrm{integer}\:{k},\:\mathrm{we}\:\mathrm{write}\: \\ $$$$\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+\mathrm{2}{x}\right)\left(\mathrm{1}+\mathrm{3}{x}\right)...\left(\mathrm{1}+{kx}\right)={a}_{\mathrm{0}} +{a}_{\mathrm{1}} {x}+{a}_{\mathrm{2}} {x}^{\mathrm{2}} +...{a}_{{k}} {x}^{{k}} \\ $$$$\mathrm{Let}\:{N}={a}_{\mathrm{0}} +{a}_{\mathrm{1}} +{a}_{\mathrm{2}} +...{a}_{{k}} \:, \\ $$$$\mathrm{if}\:{N}\:\mathrm{is}\:\mathrm{divisible}\:\mathrm{by}\:\mathrm{2019},\:\mathrm{find}\:\mathrm{the}\: \\ $$$$\mathrm{smallest}\:\mathrm{possible}\:\mathrm{value}\:\mathrm{of}\:{k}. \\ $$
Answered by Olaf last updated on 17/Sep/20
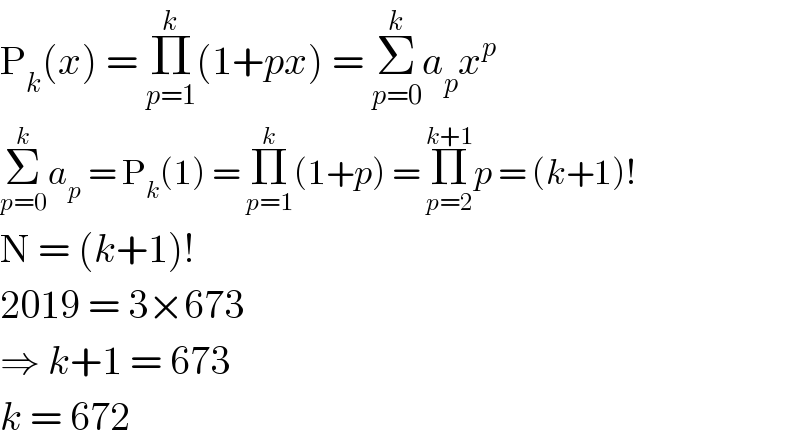
$$\mathrm{P}_{{k}} \left({x}\right)\:=\:\underset{{p}=\mathrm{1}} {\overset{{k}} {\prod}}\left(\mathrm{1}+{px}\right)\:=\:\underset{{p}=\mathrm{0}} {\overset{{k}} {\sum}}{a}_{{p}} {x}^{{p}} \\ $$$$\underset{{p}=\mathrm{0}} {\overset{{k}} {\sum}}{a}_{{p}} \:=\:\mathrm{P}_{{k}} \left(\mathrm{1}\right)\:=\:\underset{{p}=\mathrm{1}} {\overset{{k}} {\prod}}\left(\mathrm{1}+{p}\right)\:=\:\underset{{p}=\mathrm{2}} {\overset{{k}+\mathrm{1}} {\prod}}{p}\:=\:\left({k}+\mathrm{1}\right)! \\ $$$$\mathrm{N}\:=\:\left({k}+\mathrm{1}\right)! \\ $$$$\mathrm{2019}\:=\:\mathrm{3}×\mathrm{673} \\ $$$$\Rightarrow\:{k}+\mathrm{1}\:=\:\mathrm{673} \\ $$$${k}\:=\:\mathrm{672} \\ $$
Commented by ZiYangLee last updated on 18/Sep/20
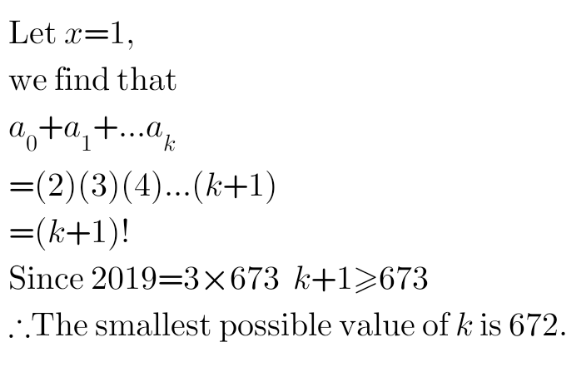
Commented by ZiYangLee last updated on 18/Sep/20

$$\mathrm{nice}\:\mathrm{try}!\: \\ $$
