
Question Number 13982 by tawa tawa last updated on 26/May/17
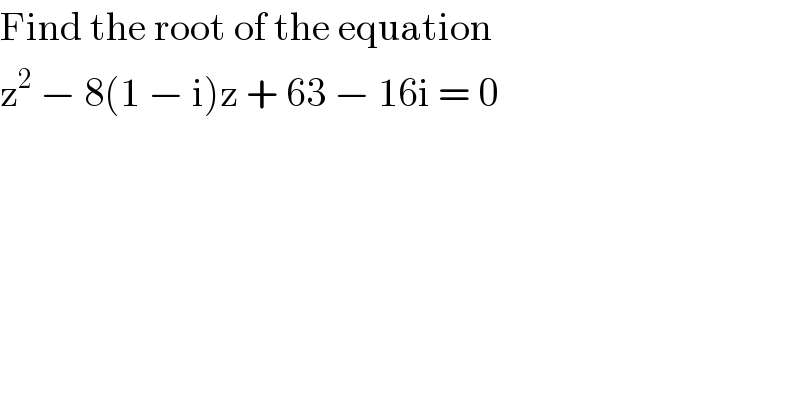
$$\mathrm{Find}\:\mathrm{the}\:\mathrm{root}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\mathrm{z}^{\mathrm{2}} \:−\:\mathrm{8}\left(\mathrm{1}\:−\:\mathrm{i}\right)\mathrm{z}\:+\:\mathrm{63}\:−\:\mathrm{16i}\:=\:\mathrm{0} \\ $$
Answered by ajfour last updated on 26/May/17
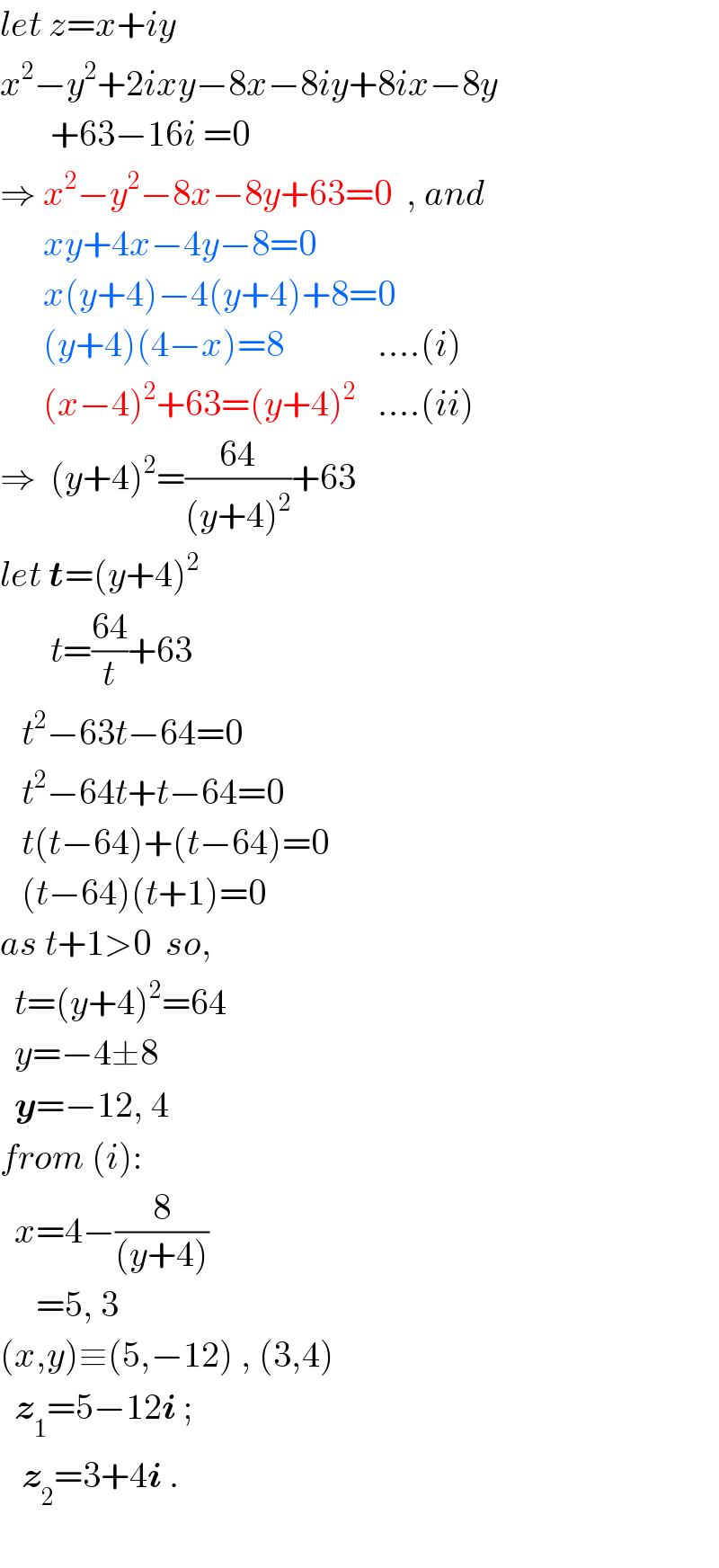
$${let}\:{z}={x}+{iy} \\ $$$${x}^{\mathrm{2}} −{y}^{\mathrm{2}} +\mathrm{2}{ixy}−\mathrm{8}{x}−\mathrm{8}{iy}+\mathrm{8}{ix}−\mathrm{8}{y} \\ $$$$\:\:\:\:\:\:\:+\mathrm{63}−\mathrm{16}{i}\:=\mathrm{0} \\ $$$$\Rightarrow\:{x}^{\mathrm{2}} −{y}^{\mathrm{2}} −\mathrm{8}{x}−\mathrm{8}{y}+\mathrm{63}=\mathrm{0}\:\:,\:{and} \\ $$$$\:\:\:\:\:\:{xy}+\mathrm{4}{x}−\mathrm{4}{y}−\mathrm{8}=\mathrm{0}\: \\ $$$$\:\:\:\:\:\:{x}\left({y}+\mathrm{4}\right)−\mathrm{4}\left({y}+\mathrm{4}\right)+\mathrm{8}=\mathrm{0} \\ $$$$\:\:\:\:\:\:\left({y}+\mathrm{4}\right)\left(\mathrm{4}−{x}\right)=\mathrm{8}\:\:\:\:\:\:\:\:\:\:\:\:\:....\left({i}\right) \\ $$$$\:\:\:\:\:\:\left({x}−\mathrm{4}\right)^{\mathrm{2}} +\mathrm{63}=\left({y}+\mathrm{4}\right)^{\mathrm{2}} \:\:\:....\left({ii}\right) \\ $$$$\Rightarrow\:\:\left({y}+\mathrm{4}\right)^{\mathrm{2}} =\frac{\mathrm{64}}{\left({y}+\mathrm{4}\right)^{\mathrm{2}} }+\mathrm{63} \\ $$$${let}\:\boldsymbol{{t}}=\left({y}+\mathrm{4}\right)^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:{t}=\frac{\mathrm{64}}{{t}}+\mathrm{63} \\ $$$$\:\:\:{t}^{\mathrm{2}} −\mathrm{63}{t}−\mathrm{64}=\mathrm{0} \\ $$$$\:\:\:{t}^{\mathrm{2}} −\mathrm{64}{t}+{t}−\mathrm{64}=\mathrm{0} \\ $$$$\:\:\:{t}\left({t}−\mathrm{64}\right)+\left({t}−\mathrm{64}\right)=\mathrm{0} \\ $$$$\:\:\:\left({t}−\mathrm{64}\right)\left({t}+\mathrm{1}\right)=\mathrm{0} \\ $$$${as}\:{t}+\mathrm{1}>\mathrm{0}\:\:{so}, \\ $$$$\:\:{t}=\left({y}+\mathrm{4}\right)^{\mathrm{2}} =\mathrm{64} \\ $$$$\:\:{y}=−\mathrm{4}\pm\mathrm{8} \\ $$$$\:\:\boldsymbol{{y}}=−\mathrm{12},\:\mathrm{4}\: \\ $$$${from}\:\left({i}\right): \\ $$$$\:\:{x}=\mathrm{4}−\frac{\mathrm{8}}{\left({y}+\mathrm{4}\right)} \\ $$$$\:\:\:\:\:=\mathrm{5},\:\mathrm{3} \\ $$$$\left({x},{y}\right)\equiv\left(\mathrm{5},−\mathrm{12}\right)\:,\:\left(\mathrm{3},\mathrm{4}\right) \\ $$$$\:\:\boldsymbol{{z}}_{\mathrm{1}} =\mathrm{5}−\mathrm{12}\boldsymbol{{i}}\:; \\ $$$$\:\:\:\boldsymbol{{z}}_{\mathrm{2}} =\mathrm{3}+\mathrm{4}\boldsymbol{{i}}\:. \\ $$$$ \\ $$
Commented by ajfour last updated on 26/May/17

$${it}\:{is}\:{correct}\:{now}\:! \\ $$
Commented by tawa tawa last updated on 26/May/17

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$
Answered by b.e.h.i.8.3.4.1.7@gmail.com last updated on 26/May/17
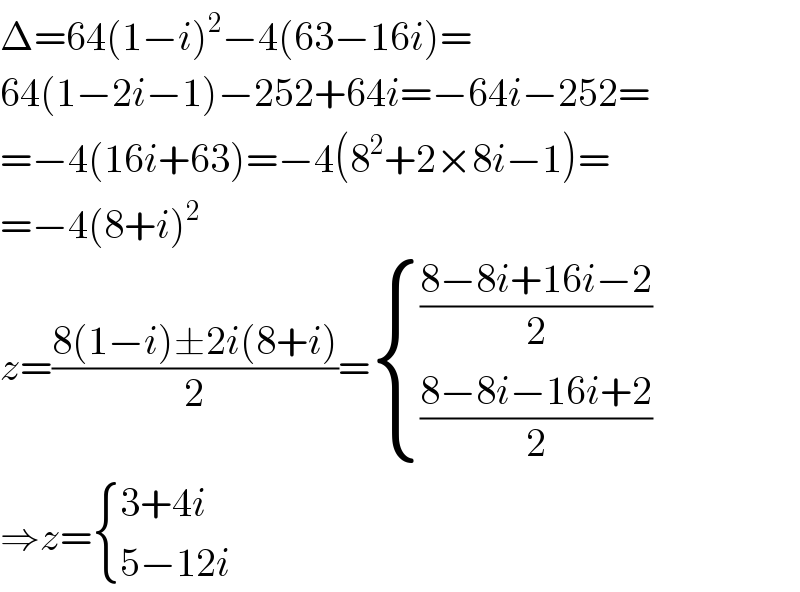
$$\Delta=\mathrm{64}\left(\mathrm{1}−{i}\right)^{\mathrm{2}} −\mathrm{4}\left(\mathrm{63}−\mathrm{16}{i}\right)= \\ $$$$\mathrm{64}\left(\mathrm{1}−\mathrm{2}{i}−\mathrm{1}\right)−\mathrm{252}+\mathrm{64}{i}=−\mathrm{64}{i}−\mathrm{252}= \\ $$$$=−\mathrm{4}\left(\mathrm{16}{i}+\mathrm{63}\right)=−\mathrm{4}\left(\mathrm{8}^{\mathrm{2}} +\mathrm{2}×\mathrm{8}{i}−\mathrm{1}\right)= \\ $$$$=−\mathrm{4}\left(\mathrm{8}+{i}\right)^{\mathrm{2}} \\ $$$${z}=\frac{\mathrm{8}\left(\mathrm{1}−{i}\right)\pm\mathrm{2}{i}\left(\mathrm{8}+{i}\right)}{\mathrm{2}}=\begin{cases}{\frac{\mathrm{8}−\mathrm{8}{i}+\mathrm{16}{i}−\mathrm{2}}{\mathrm{2}}}\\{\frac{\mathrm{8}−\mathrm{8}{i}−\mathrm{16}{i}+\mathrm{2}}{\mathrm{2}}}\end{cases} \\ $$$$\Rightarrow{z}=\begin{cases}{\mathrm{3}+\mathrm{4}{i}}\\{\mathrm{5}−\mathrm{12}{i}}\end{cases} \\ $$
Commented by tawa tawa last updated on 26/May/17

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$
