Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 198968 by necx122 last updated on 26/Oct/23

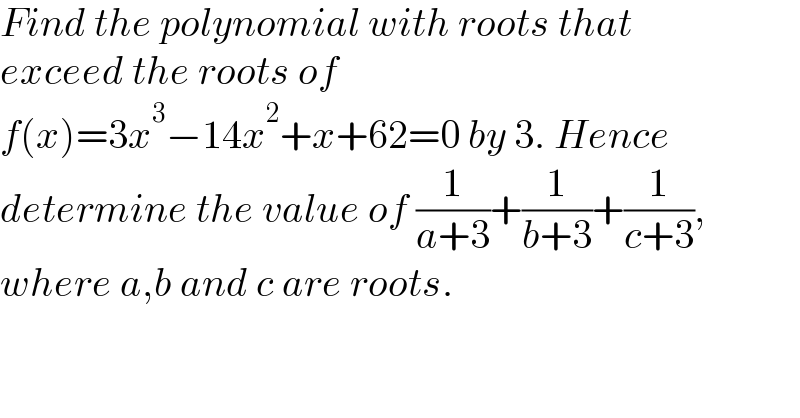
Find the polynomial with roots that exceed the roots of f(x)=3x^3 −14x^2 +x+62=0 by 3. Hence determine the value of (1/(a+3))+(1/(b+3))+(1/(c+3)), where a,b and c are roots.

$${Find}\:{the}\:{polynomial}\:{with}\:{roots}\:{that} \\ $$$${exceed}\:{the}\:{roots}\:{of}\: \\ $$$${f}\left({x}\right)=\mathrm{3}{x}^{\mathrm{3}} −\mathrm{14}{x}^{\mathrm{2}} +{x}+\mathrm{62}=\mathrm{0}\:{by}\:\mathrm{3}.\:{Hence} \\ $$$${determine}\:{the}\:{value}\:{of}\:\frac{\mathrm{1}}{{a}+\mathrm{3}}+\frac{\mathrm{1}}{{b}+\mathrm{3}}+\frac{\mathrm{1}}{{c}+\mathrm{3}}, \\ $$$${where}\:{a},{b}\:{and}\:{c}\:{are}\:{roots}. \\ $$

Answered by Rasheed.Sindhi last updated on 26/Oct/23

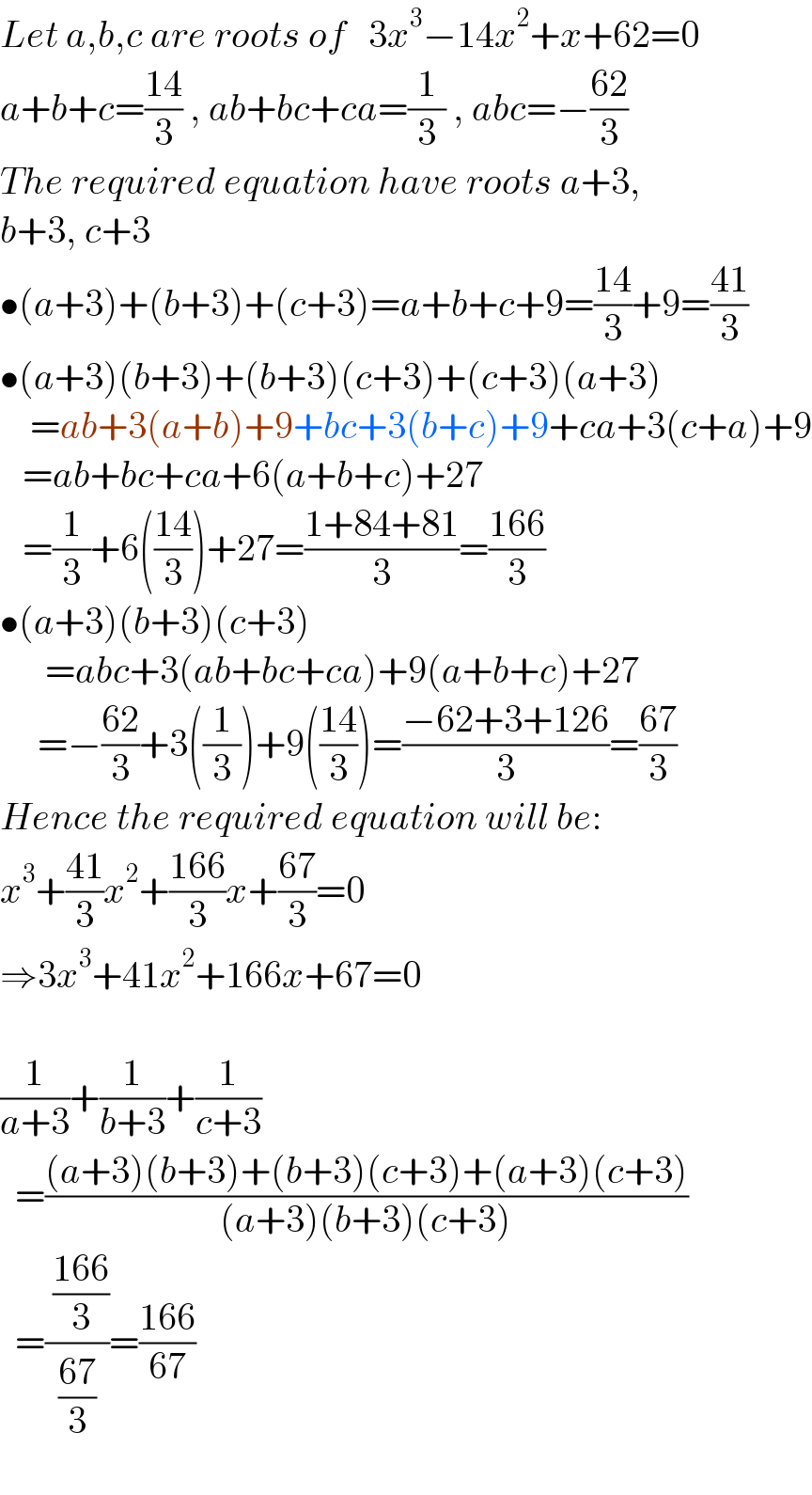
Let a,b,c are roots of 3x^3 −14x^2 +x+62=0 a+b+c=((14)/3) , ab+bc+ca=(1/3) , abc=−((62)/3) The required equation have roots a+3, b+3, c+3 •(a+3)+(b+3)+(c+3)=a+b+c+9=((14)/3)+9=((41)/3) •(a+3)(b+3)+(b+3)(c+3)+(c+3)(a+3) =ab+3(a+b)+9+bc+3(b+c)+9+ca+3(c+a)+9 =ab+bc+ca+6(a+b+c)+27 =(1/3)+6(((14)/3))+27=((1+84+81)/3)=((166)/3) •(a+3)(b+3)(c+3) =abc+3(ab+bc+ca)+9(a+b+c)+27 =−((62)/3)+3((1/3))+9(((14)/3))=((−62+3+126)/3)=((67)/3) Hence the required equation will be: x^3 +((41)/3)x^2 +((166)/3)x+((67)/3)=0 ⇒3x^3 +41x^2 +166x+67=0 (1/(a+3))+(1/(b+3))+(1/(c+3)) =(((a+3)(b+3)+(b+3)(c+3)+(a+3)(c+3))/((a+3)(b+3)(c+3))) =(( ((166)/3))/((67)/3))=((166)/(67))

$${Let}\:{a},{b},{c}\:{are}\:{roots}\:{of}\:\:\:\mathrm{3}{x}^{\mathrm{3}} −\mathrm{14}{x}^{\mathrm{2}} +{x}+\mathrm{62}=\mathrm{0} \\ $$$${a}+{b}+{c}=\frac{\mathrm{14}}{\mathrm{3}}\:,\:{ab}+{bc}+{ca}=\frac{\mathrm{1}}{\mathrm{3}}\:,\:{abc}=−\frac{\mathrm{62}}{\mathrm{3}} \\ $$$${The}\:{required}\:{equation}\:{have}\:{roots}\:{a}+\mathrm{3}, \\ $$$${b}+\mathrm{3},\:{c}+\mathrm{3} \\ $$$$\bullet\left({a}+\mathrm{3}\right)+\left({b}+\mathrm{3}\right)+\left({c}+\mathrm{3}\right)={a}+{b}+{c}+\mathrm{9}=\frac{\mathrm{14}}{\mathrm{3}}+\mathrm{9}=\frac{\mathrm{41}}{\mathrm{3}} \\ $$$$\bullet\left({a}+\mathrm{3}\right)\left({b}+\mathrm{3}\right)+\left({b}+\mathrm{3}\right)\left({c}+\mathrm{3}\right)+\left({c}+\mathrm{3}\right)\left({a}+\mathrm{3}\right) \\ $$$$\:\:\:\:={ab}+\mathrm{3}\left({a}+{b}\right)+\mathrm{9}+{bc}+\mathrm{3}\left({b}+{c}\right)+\mathrm{9}+{ca}+\mathrm{3}\left({c}+{a}\right)+\mathrm{9} \\ $$$$\:\:\:={ab}+{bc}+{ca}+\mathrm{6}\left({a}+{b}+{c}\right)+\mathrm{27} \\ $$$$\:\:\:=\frac{\mathrm{1}}{\mathrm{3}}+\mathrm{6}\left(\frac{\mathrm{14}}{\mathrm{3}}\right)+\mathrm{27}=\frac{\mathrm{1}+\mathrm{84}+\mathrm{81}}{\mathrm{3}}=\frac{\mathrm{166}}{\mathrm{3}} \\ $$$$\bullet\left({a}+\mathrm{3}\right)\left({b}+\mathrm{3}\right)\left({c}+\mathrm{3}\right) \\ $$$$\:\:\:\:\:\:={abc}+\mathrm{3}\left({ab}+{bc}+{ca}\right)+\mathrm{9}\left({a}+{b}+{c}\right)+\mathrm{27} \\ $$$$\:\:\:\:\:=−\frac{\mathrm{62}}{\mathrm{3}}+\mathrm{3}\left(\frac{\mathrm{1}}{\mathrm{3}}\right)+\mathrm{9}\left(\frac{\mathrm{14}}{\mathrm{3}}\right)=\frac{−\mathrm{62}+\mathrm{3}+\mathrm{126}}{\mathrm{3}}=\frac{\mathrm{67}}{\mathrm{3}} \\ $$$${Hence}\:{the}\:{required}\:{equation}\:{will}\:{be}: \\ $$$${x}^{\mathrm{3}} +\frac{\mathrm{41}}{\mathrm{3}}{x}^{\mathrm{2}} +\frac{\mathrm{166}}{\mathrm{3}}{x}+\frac{\mathrm{67}}{\mathrm{3}}=\mathrm{0} \\ $$$$\Rightarrow\mathrm{3}{x}^{\mathrm{3}} +\mathrm{41}{x}^{\mathrm{2}} +\mathrm{166}{x}+\mathrm{67}=\mathrm{0} \\ $$$$ \\ $$$$\frac{\mathrm{1}}{{a}+\mathrm{3}}+\frac{\mathrm{1}}{{b}+\mathrm{3}}+\frac{\mathrm{1}}{{c}+\mathrm{3}} \\ $$$$\:\:=\frac{\left({a}+\mathrm{3}\right)\left({b}+\mathrm{3}\right)+\left({b}+\mathrm{3}\right)\left({c}+\mathrm{3}\right)+\left({a}+\mathrm{3}\right)\left({c}+\mathrm{3}\right)}{\left({a}+\mathrm{3}\right)\left({b}+\mathrm{3}\right)\left({c}+\mathrm{3}\right)} \\ $$$$\:\:=\frac{\:\frac{\mathrm{166}}{\mathrm{3}}}{\frac{\mathrm{67}}{\mathrm{3}}}=\frac{\mathrm{166}}{\mathrm{67}} \\ $$$$ \\ $$

Commented by necx122 last updated on 26/Oct/23

This is great and I'm grateful. well understood. Thank you sir.

Commented by mr W last updated on 26/Oct/23

i think the question suggests one to shift the given polynomial f(x) to the right direction by 3 to get the new polynomial at first. this is the easiest way to determine the new polynomial. please recheck your calculation sir! i think something is wrong in it, see following diagram with (1)=original polynomial f(x) (2)=what you got (3)=what i got (see below)

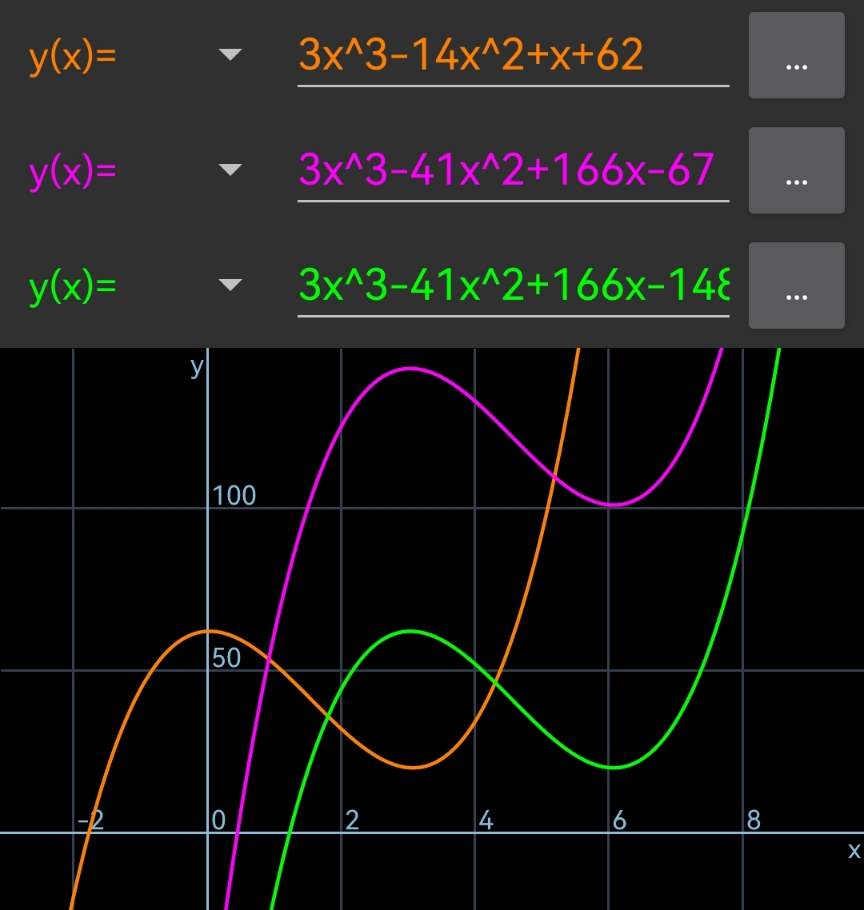
$${i}\:{think}\:{the}\:{question}\:{suggests}\:{one}\:{to} \\ $$$${shift}\:{the}\:{given}\:{polynomial}\:{f}\left({x}\right)\:{to}\:{the} \\ $$$${right}\:{direction}\:{by}\:\mathrm{3}\:{to}\:{get}\:{the}\:{new} \\ $$$${polynomial}\:{at}\:{first}.\:{this}\:{is}\:{the}\:{easiest} \\ $$$${way}\:{to}\:{determine}\:{the}\:{new}\:{polynomial}. \\ $$$$ \\ $$$${please}\:{recheck}\:{your}\:{calculation}\:{sir}! \\ $$$${i}\:{think}\:{something}\:{is}\:{wrong}\:{in}\:{it}, \\ $$$${see}\:{following}\:{diagram}\:{with} \\ $$$$\left(\mathrm{1}\right)={original}\:{polynomial}\:{f}\left({x}\right) \\ $$$$\left(\mathrm{2}\right)={what}\:{you}\:{got} \\ $$$$\left(\mathrm{3}\right)={what}\:{i}\:{got}\:\left({see}\:{below}\right) \\ $$

Commented by mr W last updated on 26/Oct/23

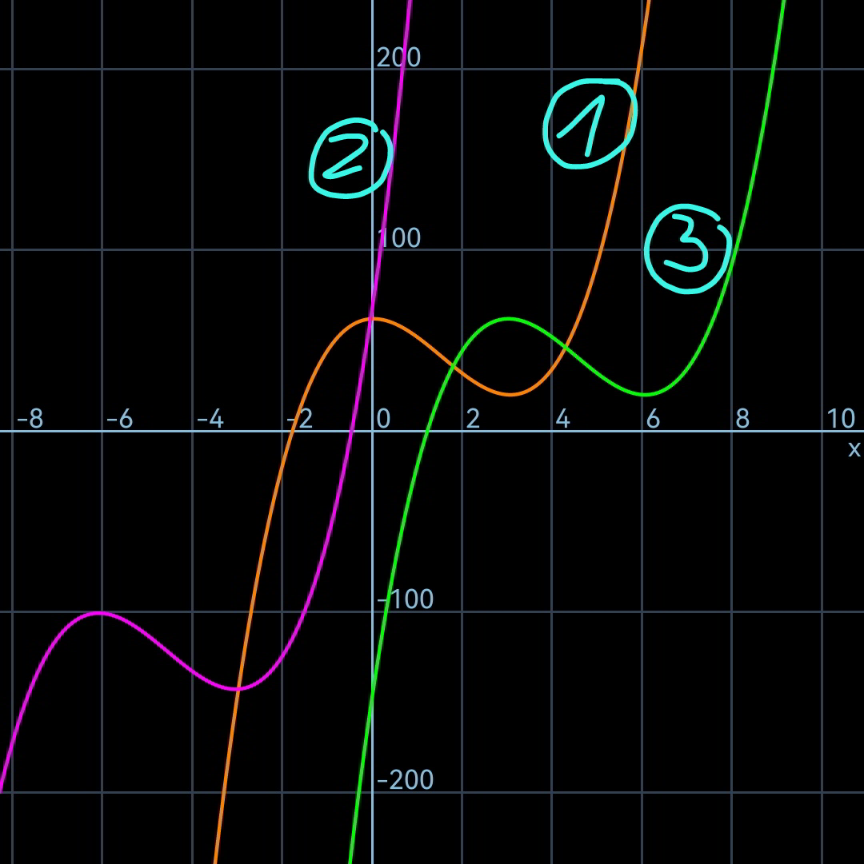
Commented by deleteduser1 last updated on 26/Oct/23

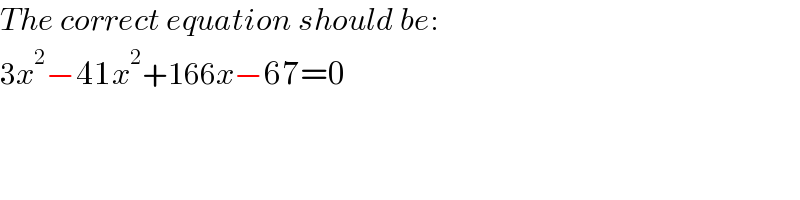
The correct equation should be: 3x^2 −41x^2 +166x−67=0

$${The}\:{correct}\:{equation}\:{should}\:{be}:\: \\ $$$$\mathrm{3}{x}^{\mathrm{2}} −\mathrm{41}{x}^{\mathrm{2}} +\mathrm{166}{x}−\mathrm{67}=\mathrm{0} \\ $$

Commented by mr W last updated on 26/Oct/23

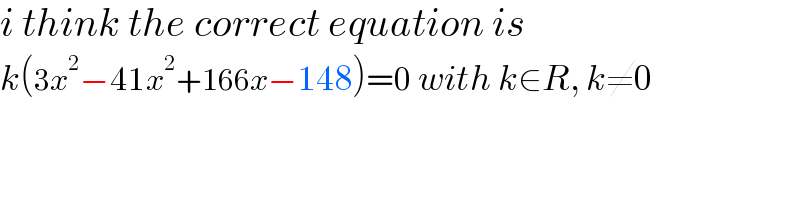
i think the correct equation is k(3x^2 −41x^2 +166x−148)=0 with k∈R, k≠0

$${i}\:{think}\:{the}\:{correct}\:{equation}\:{is} \\ $$$${k}\left(\mathrm{3}{x}^{\mathrm{2}} −\mathrm{41}{x}^{\mathrm{2}} +\mathrm{166}{x}−\mathrm{148}\right)=\mathrm{0}\:{with}\:{k}\in{R},\:{k}\neq\mathrm{0} \\ $$

Commented by mr W last updated on 26/Oct/23

Commented by deleteduser1 last updated on 26/Oct/23

I′m referring to the solution above. The solution would have been correct if a,b and c were all real numbers. (a+3)(b+3)(c+3) =abc+3(ab+bc+ca)+9(a+b+c)+27 is only true when a,b,c∈R When two(in the case of a cubic equation) come from C\R,then we are no longer dealing with variables(a,b,c) but (a,b^− ,c^− ) where b^− =x+yi. ⇒b^− +3=(x+3)+yi;so we are only increasing the real part by 3

$${I}'{m}\:{referring}\:{to}\:{the}\:{solution}\:{above}. \\ $$$${The}\:{solution}\:{would}\:{have}\:{been}\:{correct}\:{if}\:{a},{b}\:{and} \\ $$$${c}\:{were}\:{all}\:{real}\:{numbers}.\: \\ $$$$\left({a}+\mathrm{3}\right)\left({b}+\mathrm{3}\right)\left({c}+\mathrm{3}\right) \\ $$$$={abc}+\mathrm{3}\left({ab}+{bc}+{ca}\right)+\mathrm{9}\left({a}+{b}+{c}\right)+\mathrm{27}\:{is}\:{only}\:{true} \\ $$$${when}\:{a},{b},{c}\in\mathbb{R} \\ $$$${When}\:{two}\left({in}\:{the}\:{case}\:{of}\:{a}\:{cubic}\:{equation}\right)\:{come} \\ $$$${from}\:\mathbb{C}\backslash\mathbb{R},{then}\:{we}\:{are}\:{no}\:{longer}\:{dealing}\:{with} \\ $$$${variables}\left({a},{b},{c}\right)\:{but}\:\left({a},\overset{−} {{b}},\overset{−} {{c}}\right)\:{where}\:\overset{−} {{b}}={x}+{yi}. \\ $$$$\Rightarrow\overset{−} {{b}}+\mathrm{3}=\left({x}+\mathrm{3}\right)+{yi};{so}\:{we}\:{are}\:{only}\:{increasing} \\ $$$${the}\:{real}\:{part}\:{by}\:\mathrm{3} \\ $$

Answered by mr W last updated on 26/Oct/23

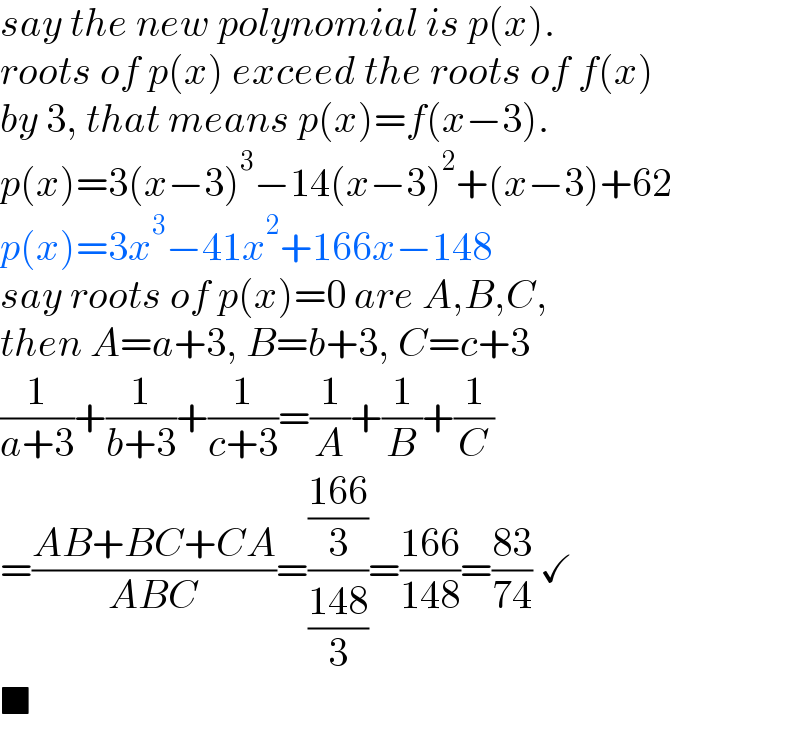
say the new polynomial is p(x). roots of p(x) exceed the roots of f(x) by 3, that means p(x)=f(x−3). p(x)=3(x−3)^3 −14(x−3)^2 +(x−3)+62 p(x)=3x^3 −41x^2 +166x−148 say roots of p(x)=0 are A,B,C, then A=a+3, B=b+3, C=c+3 (1/(a+3))+(1/(b+3))+(1/(c+3))=(1/A)+(1/B)+(1/C) =((AB+BC+CA)/(ABC))=(((166)/3)/((148)/3))=((166)/(148))=((83)/(74)) ✓ ■

$${say}\:{the}\:{new}\:{polynomial}\:{is}\:{p}\left({x}\right). \\ $$$${roots}\:{of}\:{p}\left({x}\right)\:{exceed}\:{the}\:{roots}\:{of}\:{f}\left({x}\right) \\ $$$${by}\:\mathrm{3},\:{that}\:{means}\:{p}\left({x}\right)={f}\left({x}−\mathrm{3}\right). \\ $$$${p}\left({x}\right)=\mathrm{3}\left({x}−\mathrm{3}\right)^{\mathrm{3}} −\mathrm{14}\left({x}−\mathrm{3}\right)^{\mathrm{2}} +\left({x}−\mathrm{3}\right)+\mathrm{62} \\ $$$${p}\left({x}\right)=\mathrm{3}{x}^{\mathrm{3}} −\mathrm{41}{x}^{\mathrm{2}} +\mathrm{166}{x}−\mathrm{148} \\ $$$${say}\:{roots}\:{of}\:{p}\left({x}\right)=\mathrm{0}\:{are}\:{A},{B},{C}, \\ $$$${then}\:{A}={a}+\mathrm{3},\:{B}={b}+\mathrm{3},\:{C}={c}+\mathrm{3} \\ $$$$\frac{\mathrm{1}}{{a}+\mathrm{3}}+\frac{\mathrm{1}}{{b}+\mathrm{3}}+\frac{\mathrm{1}}{{c}+\mathrm{3}}=\frac{\mathrm{1}}{{A}}+\frac{\mathrm{1}}{{B}}+\frac{\mathrm{1}}{{C}} \\ $$$$=\frac{{AB}+{BC}+{CA}}{{ABC}}=\frac{\frac{\mathrm{166}}{\mathrm{3}}}{\frac{\mathrm{148}}{\mathrm{3}}}=\frac{\mathrm{166}}{\mathrm{148}}=\frac{\mathrm{83}}{\mathrm{74}}\:\checkmark \\ $$$$\blacksquare \\ $$

Commented by necx122 last updated on 26/Oct/23

Wow!! Having to exclaim is all i can do at this point. Funnily, both answers appear in the options.

Commented by Rasheed.Sindhi last updated on 27/Oct/23

Most efficient way!

$$\mathrm{Most}\:\mathrm{efficient}\:\mathrm{way}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com