Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 121264 by benjo_mathlover last updated on 06/Nov/20

Find the point on the curve x=2y^2 closest to the point (10,0)

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{point}\:\mathrm{on}\:\mathrm{the}\:\mathrm{curve} \\ $$$$\mathrm{x}=\mathrm{2y}^{\mathrm{2}} \:\mathrm{closest}\:\mathrm{to}\:\mathrm{the}\:\mathrm{point} \\ $$$$\left(\mathrm{10},\mathrm{0}\right) \\ $$

Answered by liberty last updated on 06/Nov/20

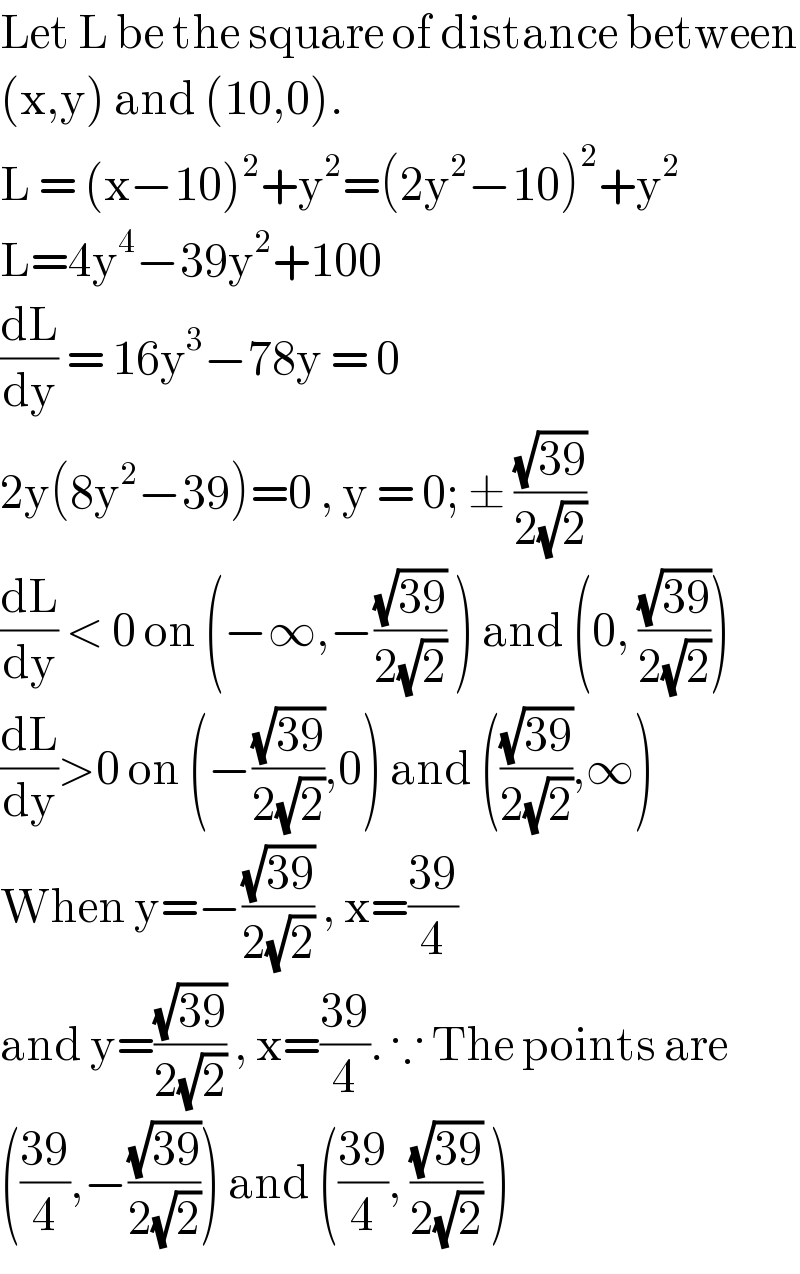
Let L be the square of distance between (x,y) and (10,0). L = (x−10)^2 +y^2 =(2y^2 −10)^2 +y^2 L=4y^4 −39y^2 +100 (dL/dy) = 16y^3 −78y = 0 2y(8y^2 −39)=0 , y = 0; ± ((√(39))/(2(√2))) (dL/dy) < 0 on (−∞,−((√(39))/(2(√2))) ) and (0, ((√(39))/(2(√2)))) (dL/dy)>0 on (−((√(39))/(2(√2))),0) and (((√(39))/(2(√2))),∞) When y=−((√(39))/(2(√2))) , x=((39)/4) and y=((√(39))/(2(√2))) , x=((39)/4). ∵ The points are (((39)/4),−((√(39))/(2(√2)))) and (((39)/4), ((√(39))/(2(√2))) )

$$\mathrm{Let}\:\mathrm{L}\:\mathrm{be}\:\mathrm{the}\:\mathrm{square}\:\mathrm{of}\:\mathrm{distance}\:\mathrm{between} \\ $$$$\left(\mathrm{x},\mathrm{y}\right)\:\mathrm{and}\:\left(\mathrm{10},\mathrm{0}\right). \\ $$$$\mathrm{L}\:=\:\left(\mathrm{x}−\mathrm{10}\right)^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} =\left(\mathrm{2y}^{\mathrm{2}} −\mathrm{10}\right)^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} \\ $$$$\mathrm{L}=\mathrm{4y}^{\mathrm{4}} −\mathrm{39y}^{\mathrm{2}} +\mathrm{100} \\ $$$$\frac{\mathrm{dL}}{\mathrm{dy}}\:=\:\mathrm{16y}^{\mathrm{3}} −\mathrm{78y}\:=\:\mathrm{0}\: \\ $$$$\mathrm{2y}\left(\mathrm{8y}^{\mathrm{2}} −\mathrm{39}\right)=\mathrm{0}\:,\:\mathrm{y}\:=\:\mathrm{0};\:\pm\:\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$$$\frac{\mathrm{dL}}{\mathrm{dy}}\:<\:\mathrm{0}\:\mathrm{on}\:\left(−\infty,−\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}}\:\right)\:\mathrm{and}\:\left(\mathrm{0},\:\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}}\right) \\ $$$$\frac{\mathrm{dL}}{\mathrm{dy}}>\mathrm{0}\:\mathrm{on}\:\left(−\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}},\mathrm{0}\right)\:\mathrm{and}\:\left(\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}},\infty\right) \\ $$$$\mathrm{When}\:\mathrm{y}=−\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}}\:,\:\mathrm{x}=\frac{\mathrm{39}}{\mathrm{4}} \\ $$$$\mathrm{and}\:\mathrm{y}=\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}}\:,\:\mathrm{x}=\frac{\mathrm{39}}{\mathrm{4}}.\:\because\:\mathrm{The}\:\mathrm{points}\:\mathrm{are} \\ $$$$\left(\frac{\mathrm{39}}{\mathrm{4}},−\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}}\right)\:\mathrm{and}\:\left(\frac{\mathrm{39}}{\mathrm{4}},\:\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}}\:\right) \\ $$

Answered by mr W last updated on 06/Nov/20

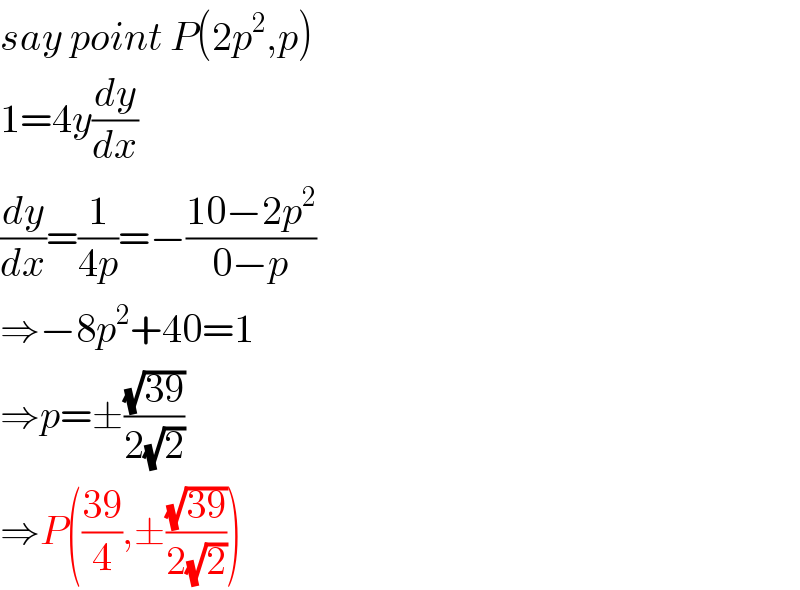
say point P(2p^2 ,p) 1=4y(dy/dx) (dy/dx)=(1/(4p))=−((10−2p^2 )/(0−p)) ⇒−8p^2 +40=1 ⇒p=±((√(39))/(2(√2))) ⇒P(((39)/4),±((√(39))/(2(√2))))

$${say}\:{point}\:{P}\left(\mathrm{2}{p}^{\mathrm{2}} ,{p}\right) \\ $$$$\mathrm{1}=\mathrm{4}{y}\frac{{dy}}{{dx}} \\ $$$$\frac{{dy}}{{dx}}=\frac{\mathrm{1}}{\mathrm{4}{p}}=−\frac{\mathrm{10}−\mathrm{2}{p}^{\mathrm{2}} }{\mathrm{0}−{p}} \\ $$$$\Rightarrow−\mathrm{8}{p}^{\mathrm{2}} +\mathrm{40}=\mathrm{1} \\ $$$$\Rightarrow{p}=\pm\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}} \\ $$$$\Rightarrow{P}\left(\frac{\mathrm{39}}{\mathrm{4}},\pm\frac{\sqrt{\mathrm{39}}}{\mathrm{2}\sqrt{\mathrm{2}}}\right) \\ $$

Commented by liberty last updated on 06/Nov/20

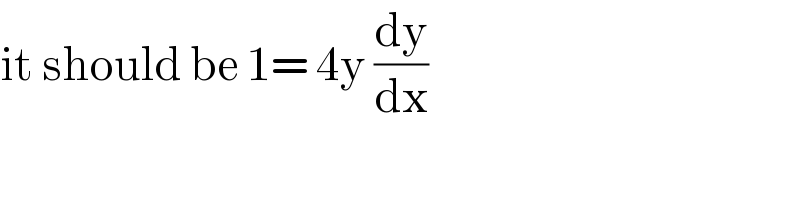
it should be 1= 4y (dy/dx)

$$\mathrm{it}\:\mathrm{should}\:\mathrm{be}\:\mathrm{1}=\:\mathrm{4y}\:\frac{\mathrm{dy}}{\mathrm{dx}} \\ $$$$ \\ $$

Commented by mr W last updated on 06/Nov/20

yes. thanks!

$${yes}.\:{thanks}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com