
Question Number 129758 by I want to learn more last updated on 18/Jan/21
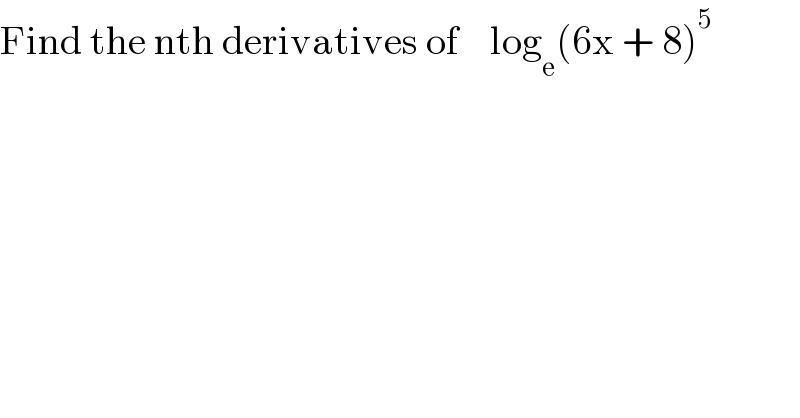
$$\mathrm{Find}\:\mathrm{the}\:\mathrm{nth}\:\mathrm{derivatives}\:\mathrm{of}\:\:\:\:\mathrm{log}_{\mathrm{e}} \left(\mathrm{6x}\:+\:\mathrm{8}\right)^{\mathrm{5}} \\ $$
Commented by Dwaipayan Shikari last updated on 18/Jan/21
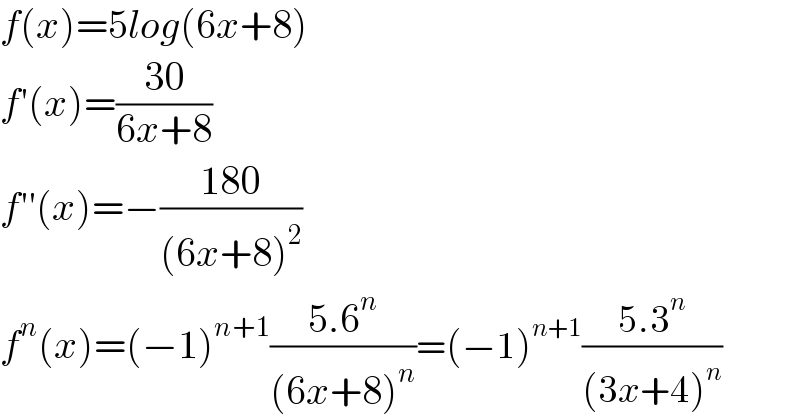
$${f}\left({x}\right)=\mathrm{5}{log}\left(\mathrm{6}{x}+\mathrm{8}\right) \\ $$$${f}'\left({x}\right)=\frac{\mathrm{30}}{\mathrm{6}{x}+\mathrm{8}} \\ $$$${f}''\left({x}\right)=−\frac{\mathrm{180}}{\left(\mathrm{6}{x}+\mathrm{8}\right)^{\mathrm{2}} }\:\: \\ $$$${f}^{{n}} \left({x}\right)=\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} \frac{\mathrm{5}.\mathrm{6}^{{n}} }{\left(\mathrm{6}{x}+\mathrm{8}\right)^{{n}} }=\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} \frac{\mathrm{5}.\mathrm{3}^{{n}} }{\left(\mathrm{3}{x}+\mathrm{4}\right)^{{n}} } \\ $$
Commented by I want to learn more last updated on 18/Jan/21
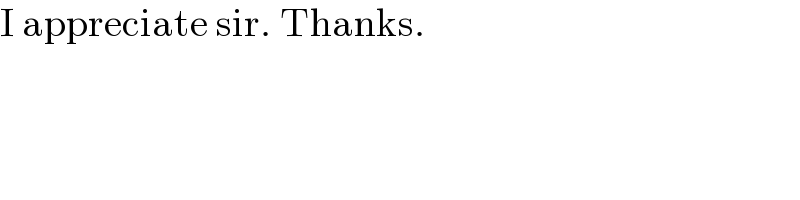
$$\mathrm{I}\:\mathrm{appreciate}\:\mathrm{sir}.\:\mathrm{Thanks}. \\ $$
