Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 203527 by Mastermind last updated on 21/Jan/24

Find the maximum value of the function f(x,y)=x^2 y^2 z^2 subject to the condition that x^2 +y^2 +z^2 =c^2 , where c is the constant. Thank you in advance

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{maximum}\:\mathrm{value}\:\mathrm{of}\:\mathrm{the}\:\mathrm{function} \\ $$$$\mathrm{f}\left(\mathrm{x},\mathrm{y}\right)=\mathrm{x}^{\mathrm{2}} \mathrm{y}^{\mathrm{2}} \mathrm{z}^{\mathrm{2}} \:\mathrm{subject}\:\mathrm{to}\:\mathrm{the}\:\mathrm{condition}\:\mathrm{that} \\ $$$$\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} +\mathrm{z}^{\mathrm{2}} =\mathrm{c}^{\mathrm{2}} ,\:\mathrm{where}\:\mathrm{c}\:\mathrm{is}\:\mathrm{the}\:\mathrm{constant}. \\ $$$$ \\ $$$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{in}\:\mathrm{advance} \\ $$

Answered by aleks041103 last updated on 21/Jan/24

x^2 =X y^2 =Y z^2 =Z c^2 =C max of XYZ if X+Y+Z=C, X,Y,Z,C>0 geom.mean≤arithm.mean ⇒((XYZ))^(1/3) ≤((X+Y+Z)/3)=(C/3) (equality if X=Y=Z) ⇒XYZ≤(C^3 /(27)) ⇒max f(x,y,z)=x^2 y^2 z^2 if x^2 +y^2 +z^2 =c^2 is max(f)=(c^6 /(27)) for ∣x∣=∣y∣=∣z∣=((∣c∣)/( (√3)))

$${x}^{\mathrm{2}} ={X} \\ $$$${y}^{\mathrm{2}} ={Y} \\ $$$${z}^{\mathrm{2}} ={Z} \\ $$$${c}^{\mathrm{2}} ={C} \\ $$$${max}\:{of}\:{XYZ}\:{if}\:{X}+{Y}+{Z}={C},\:{X},{Y},{Z},{C}>\mathrm{0} \\ $$$${geom}.{mean}\leqslant{arithm}.{mean} \\ $$$$\Rightarrow\sqrt[{\mathrm{3}}]{{XYZ}}\leqslant\frac{{X}+{Y}+{Z}}{\mathrm{3}}=\frac{{C}}{\mathrm{3}}\:\left({equality}\:{if}\:{X}={Y}={Z}\right) \\ $$$$\Rightarrow{XYZ}\leqslant\frac{{C}^{\mathrm{3}} }{\mathrm{27}} \\ $$$$\Rightarrow{max}\:{f}\left({x},{y},{z}\right)={x}^{\mathrm{2}} {y}^{\mathrm{2}} {z}^{\mathrm{2}} \:\:\:{if}\:\:\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} ={c}^{\mathrm{2}} \\ $$$${is}\:{max}\left({f}\right)=\frac{{c}^{\mathrm{6}} }{\mathrm{27}}\:{for}\:\mid{x}\mid=\mid{y}\mid=\mid{z}\mid=\frac{\mid{c}\mid}{\:\sqrt{\mathrm{3}}} \\ $$

Commented by Mastermind last updated on 21/Jan/24

Thank you so much but could you please help me with full details Thank you once again

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{so}\:\mathrm{much}\:\mathrm{but}\:\mathrm{could}\:\mathrm{you}\:\mathrm{please} \\ $$$$\mathrm{help}\:\mathrm{me}\:\mathrm{with}\:\mathrm{full}\:\mathrm{details} \\ $$$$ \\ $$$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{once}\:\mathrm{again} \\ $$

Commented by aleks041103 last updated on 21/Jan/24

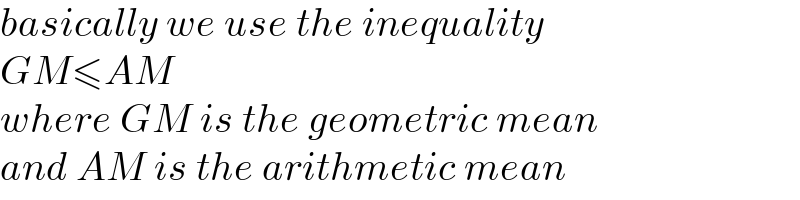
basically we use the inequality GM≤AM where GM is the geometric mean and AM is the arithmetic mean

$${basically}\:{we}\:{use}\:{the}\:{inequality} \\ $$$${GM}\leqslant{AM} \\ $$$${where}\:{GM}\:{is}\:{the}\:{geometric}\:{mean} \\ $$$${and}\:{AM}\:{is}\:{the}\:{arithmetic}\:{mean} \\ $$

Answered by deleteduser1 last updated on 21/Jan/24

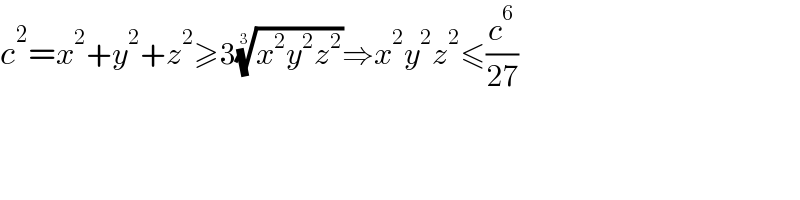
c^2 =x^2 +y^2 +z^2 ≥3((x^2 y^2 z^2 ))^(1/3) ⇒x^2 y^2 z^2 ≤(c^6 /(27))

$${c}^{\mathrm{2}} ={x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \geqslant\mathrm{3}\sqrt[{\mathrm{3}}]{{x}^{\mathrm{2}} {y}^{\mathrm{2}} {z}^{\mathrm{2}} }\Rightarrow{x}^{\mathrm{2}} {y}^{\mathrm{2}} {z}^{\mathrm{2}} \leqslant\frac{{c}^{\mathrm{6}} }{\mathrm{27}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com