Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 139024 by bramlexs22 last updated on 21/Apr/21

Find the exact value of cos (π/5)

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{exact}\:\mathrm{value}\:\mathrm{of}\:\mathrm{cos}\:\frac{\pi}{\mathrm{5}} \\ $$$$ \\ $$

Commented by EDWIN88 last updated on 21/Apr/21

(((√5)+1)/4)

$$\:\frac{\sqrt{\mathrm{5}}+\mathrm{1}}{\mathrm{4}} \\ $$

Answered by Dwaipayan Shikari last updated on 21/Apr/21

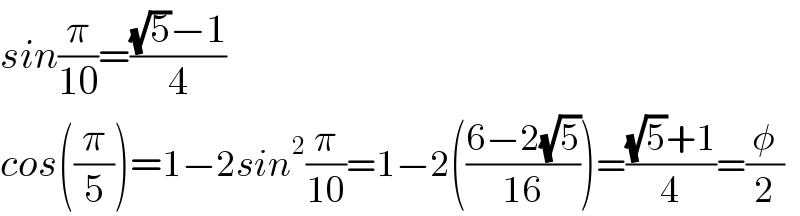
sin(π/(10))=(((√5)−1)/4) cos((π/5))=1−2sin^2 (π/(10))=1−2(((6−2(√5))/(16)))=(((√5)+1)/4)=(φ/2)

$${sin}\frac{\pi}{\mathrm{10}}=\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{4}}\:\:\: \\ $$$${cos}\left(\frac{\pi}{\mathrm{5}}\right)=\mathrm{1}−\mathrm{2}{sin}^{\mathrm{2}} \frac{\pi}{\mathrm{10}}=\mathrm{1}−\mathrm{2}\left(\frac{\mathrm{6}−\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{16}}\right)=\frac{\sqrt{\mathrm{5}}+\mathrm{1}}{\mathrm{4}}=\frac{\phi}{\mathrm{2}} \\ $$

Answered by MJS_new last updated on 21/Apr/21

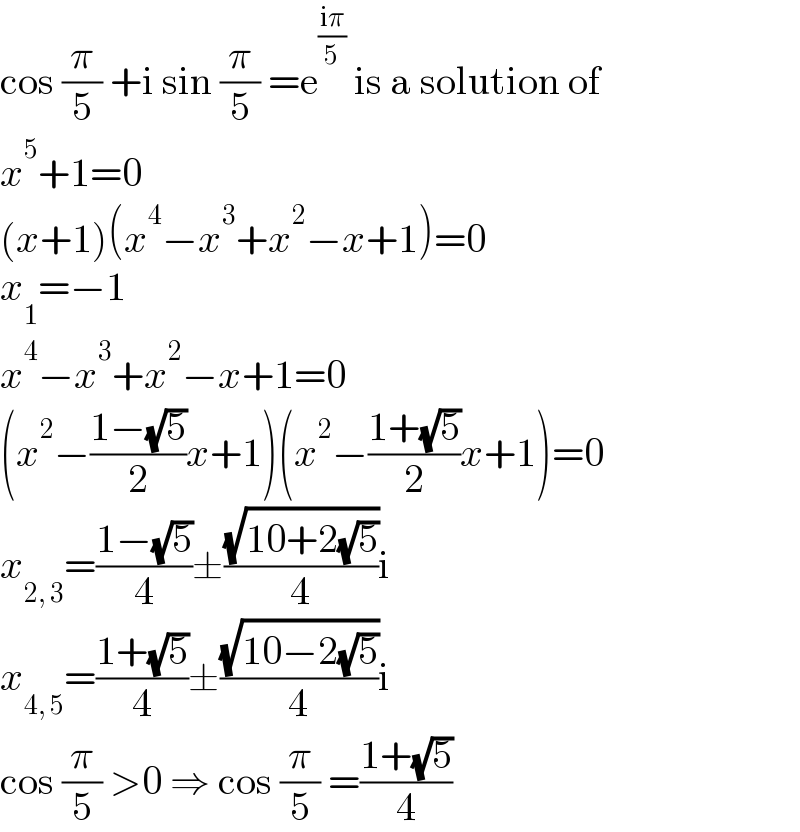
cos (π/5) +i sin (π/5) =e^((iπ)/5) is a solution of x^5 +1=0 (x+1)(x^4 −x^3 +x^2 −x+1)=0 x_1 =−1 x^4 −x^3 +x^2 −x+1=0 (x^2 −((1−(√5))/2)x+1)(x^2 −((1+(√5))/2)x+1)=0 x_(2, 3) =((1−(√5))/4)±((√(10+2(√5)))/4)i x_(4, 5) =((1+(√5))/4)±((√(10−2(√5)))/4)i cos (π/5) >0 ⇒ cos (π/5) =((1+(√5))/4)

$$\mathrm{cos}\:\frac{\pi}{\mathrm{5}}\:+\mathrm{i}\:\mathrm{sin}\:\frac{\pi}{\mathrm{5}}\:=\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{5}}} \:\mathrm{is}\:\mathrm{a}\:\mathrm{solution}\:\mathrm{of} \\ $$$${x}^{\mathrm{5}} +\mathrm{1}=\mathrm{0} \\ $$$$\left({x}+\mathrm{1}\right)\left({x}^{\mathrm{4}} −{x}^{\mathrm{3}} +{x}^{\mathrm{2}} −{x}+\mathrm{1}\right)=\mathrm{0} \\ $$$${x}_{\mathrm{1}} =−\mathrm{1} \\ $$$${x}^{\mathrm{4}} −{x}^{\mathrm{3}} +{x}^{\mathrm{2}} −{x}+\mathrm{1}=\mathrm{0} \\ $$$$\left({x}^{\mathrm{2}} −\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} −\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right)=\mathrm{0} \\ $$$${x}_{\mathrm{2},\:\mathrm{3}} =\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{4}}\pm\frac{\sqrt{\mathrm{10}+\mathrm{2}\sqrt{\mathrm{5}}}}{\mathrm{4}}\mathrm{i} \\ $$$${x}_{\mathrm{4},\:\mathrm{5}} =\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{4}}\pm\frac{\sqrt{\mathrm{10}−\mathrm{2}\sqrt{\mathrm{5}}}}{\mathrm{4}}\mathrm{i} \\ $$$$\mathrm{cos}\:\frac{\pi}{\mathrm{5}}\:>\mathrm{0}\:\Rightarrow\:\mathrm{cos}\:\frac{\pi}{\mathrm{5}}\:=\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{4}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com